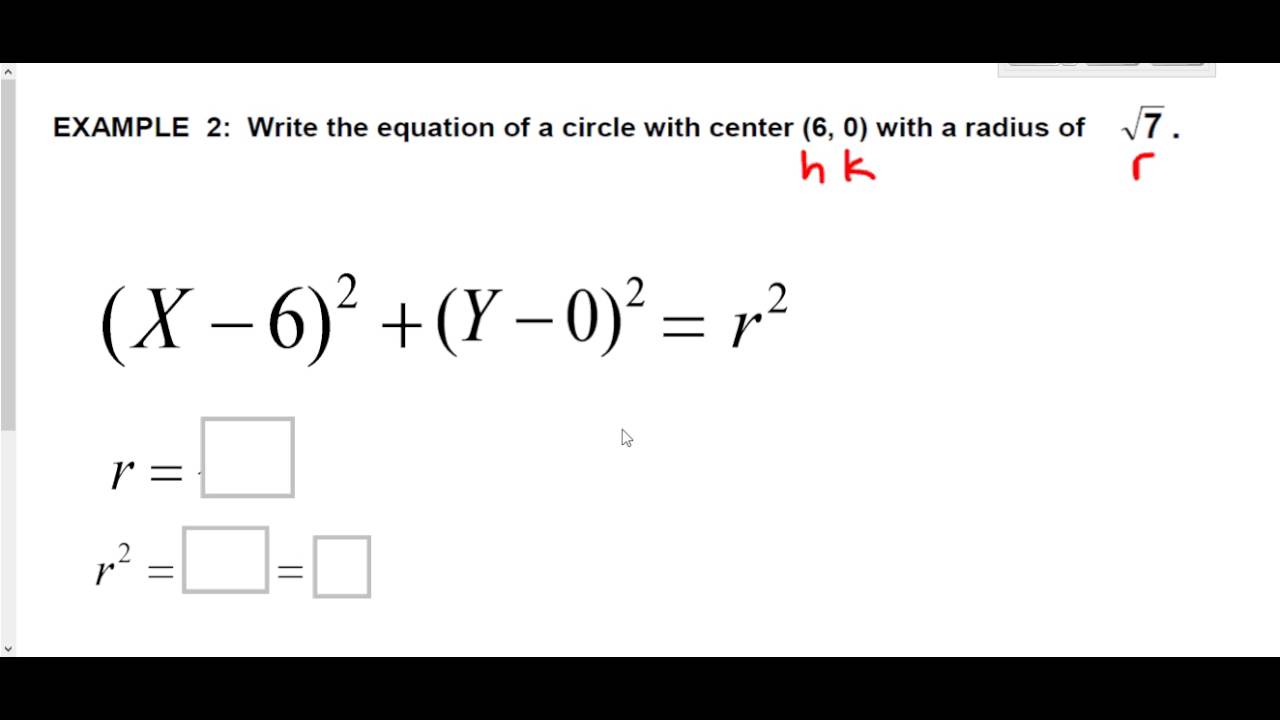
Circle equations questions require us to understand the connection between these equations and the features of circles. Solution this problem is somewhat similar to the second example above. Write the equation of the circle with radius √7 7 and center (−1,−9) ( − 1, − 9).
Equation of a tangent to a circle in 2020 Worksheets
8/5/2018 equation of a circle problems 16/20 10 determine the equation of the circle which has its center at c = (3, 1) and a tangent of 3x − 4y + 5 = 0.
To graph a circle, we.
The equation of this circle is given by: Standard equation of a circle : (x−9)2+(y +4)2 = 25 ( x − 9) 2 + ( y + 4) 2 = 25 solution. The equation represents a circle with a center at and a radius of.
Circumference of a circle = 44 cm.
Find the constant the completes the square for. (−13 , −16) point on circle: (18 , −13) and (4, −3) (x − 11)2 + (y + 8)2 = 74 12) center: Given parameters are center (a, b) = (4, 5);
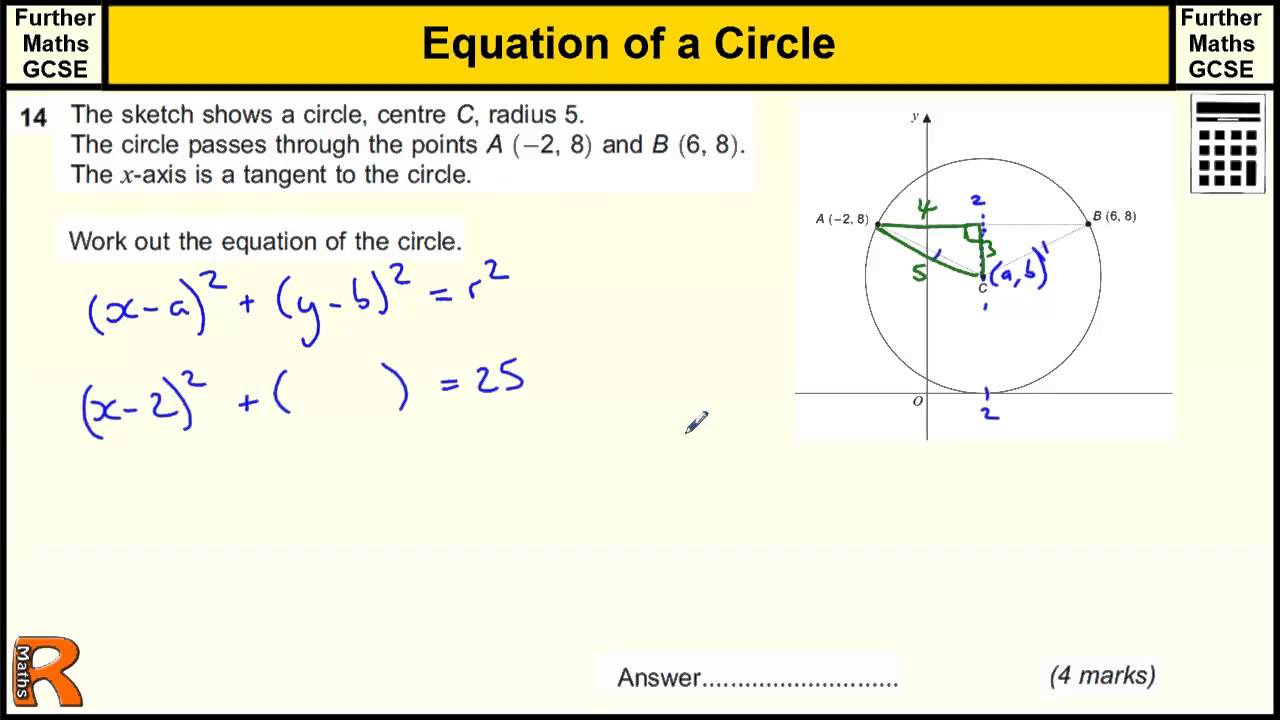
Instead, it is known that the circle passes through a.
H and k are the x and y coordinates of the center of the circle. (−10 , −16) (x + 13)2 + (y + 16)2 = 9 11) ends of a diameter: (x 2 2) 2 1 (y 1 1) 2 5 9 simplify. Use the information provided to write the equation of each circle.
Circle by completing the square, then identify the center or radius).
(x 2 3) 2 1 (y 2 5) 2 = 42 this is an example of the.standard equation of a circle 628 chapter 11 circles write the standard equation of the circle with center (2, 21) and radius 3. R = 44 x (7/44) r = 7 cm. −6 ;2 = 36 for the equation above, what is the coordinate point for the center of the circle as well as the circle’s radius? To rewrite an expanded circle equation in standard form:
2 π r = 44.
(x 2 2)2 1 (y 2 (21)) 2 5 32 substitute 2 for h, 21 for k, and 3 for r. X2 +(y−5)2 = 4 x 2 + (. 4 (x − 13)2 + (y + 13)2 = 16 10) center: 8/5/2018 equation of a circle problems 14/20 2 3x + 4y − 27 = 0.
2 x (22/7) x r = 44 (44/7) x r = 44.
Multiply each side by 7/44. So the circle is all the points (x,y) that are r away from the center (a,b). In this case, the centre is given but not the radius. Circles can also be given in expanded form, which is simply the result of expanding the binomial squares in the standard form and combining like terms.
It is a circle with a center at and a radius of.
( x − 9) 2 + ( y − 6) 2 = 100 is a circle centered at (9, 6) with a radius of 10. It is the same idea as before, but we need to subtract a and b: This means that, using pythagoras’ theorem, the equation of a circle with radius r and centre (0, 0) is given by the formula \ (x^2 + y^2 = r^2\). How do i solve these types of problems?
If thee wire is bent in the form of a circle, then the circumference of the circle is equal to length of the wire.
What is the standard equation of a circle? The formula is ( x − h) 2 + ( y − k) 2 = r 2. Represent this as a circle equation ? (10 , −14) tangent to x = 13 (x − 10)2 + (y + 14)2 = 9
Example 4 find the equation of the circle whose centre is at (3, 4) and passes through the point (2, 6).
If the center point and radius of a circle is given as (4, 5) and 7 respectively. Area of the circle is = π r 2 = (22/7) x 7 x 7 = 154 sq. For example, the equation of the circle centered at with radius is. (x−a) 2 + (y−b) 2 = r 2.
Solution (x 2 h)2 1 (y 2 k)2 5 r2 write the standard equation of a circle.
This is the general standard equation for the circle centered at with radius.