Example 2 find the equation of the circle whose centre is at the origin and which passes through the point (3, 2). How to find the equation of a circle sat math. It is a circle equation, but in disguise!
Ex 1 Write General Equation of a Circle in Standard Form
(x + 9) 2 + (y + 7) 2 = 5 b:
Write the standard form of a circle with radius 3 3 and center (0,0) ( 0, 0).
The standard form is simpler to understand when compared with the general form of the equation of a circle. To find the centre and radius of the circle, we first need to transform the. X2 + y2 + 2gx + 2fy + c + g2 + f2 = g2 + f2. Represent this as a circle equation ?
Convert the equation of a circle in general form below to standard form.
(x−1)2 + (y−2)2 = 32. Equation of a circle in standard form : X2 − 2x + 1 + y2 − 4y + 4 = 9. But it is known that the circle passes through the point (3, 2), which means its coordinates.
If the minus sign is preceding the (h, k), (h, k) will be positive.
By knowing the center and radius of the circle we can write the standard form of a circle. Solution a slight twist in the previous example. Write the equation of a circle in standard form. Standard form of circle equation expii.
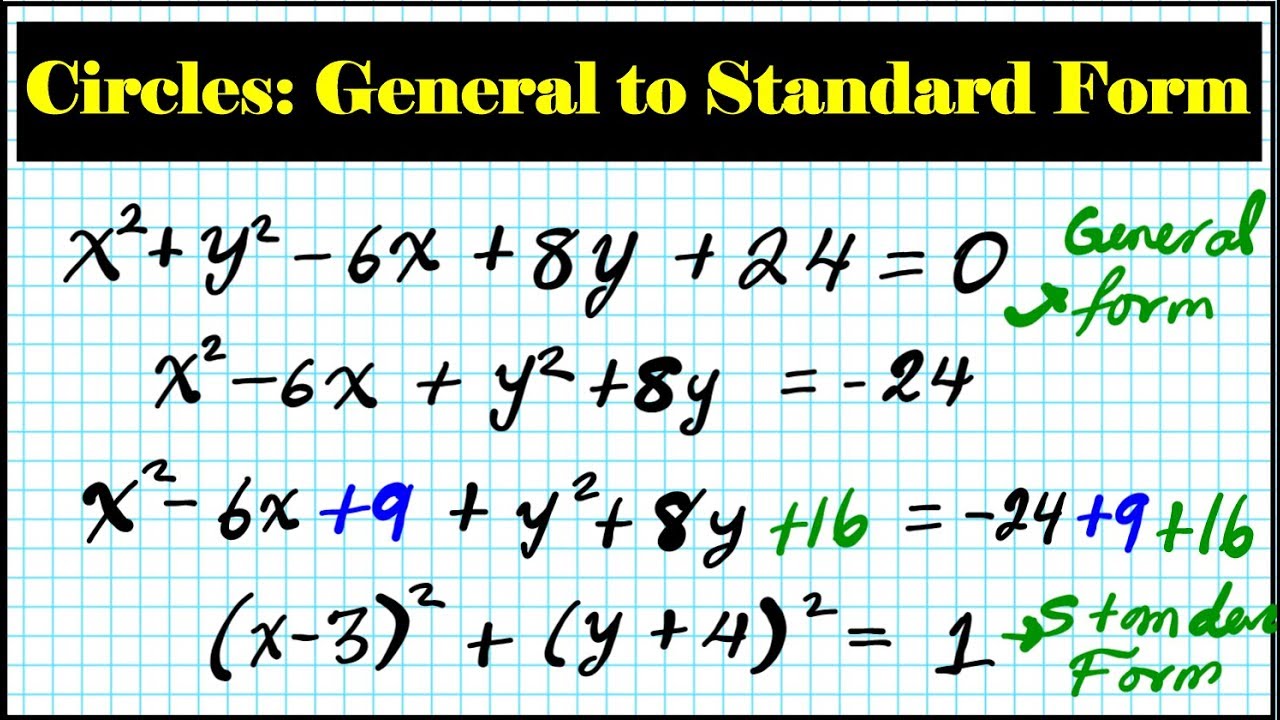
X2 + y2 − 2x − 4y − 4 = 0.
Substitute in the values r= 3,h =0,k = 0 r = 3, h = 0, k = 0. General form of the equation of a circle examples. Find the radius of the standard equation by converting the following standard equation of a circle into the polar form: Use the standard form of a circle.
The required equation is x 2 + y 2 = 4 2.
Equation of a circle in standard form formula practice problems and pictures how to express with given radius the everything you need know mashup math circles review ytic geometry article khan academy ex 1 write general help from arithmetic through calculus beyond example graph an equation of a circle in standard form formula practice problems and. Compare the given equation with the standard form, we get: Chapter 1 graphs section 5 circles a circle. (x + 9) 2 + (y + 7) 2 = 25.
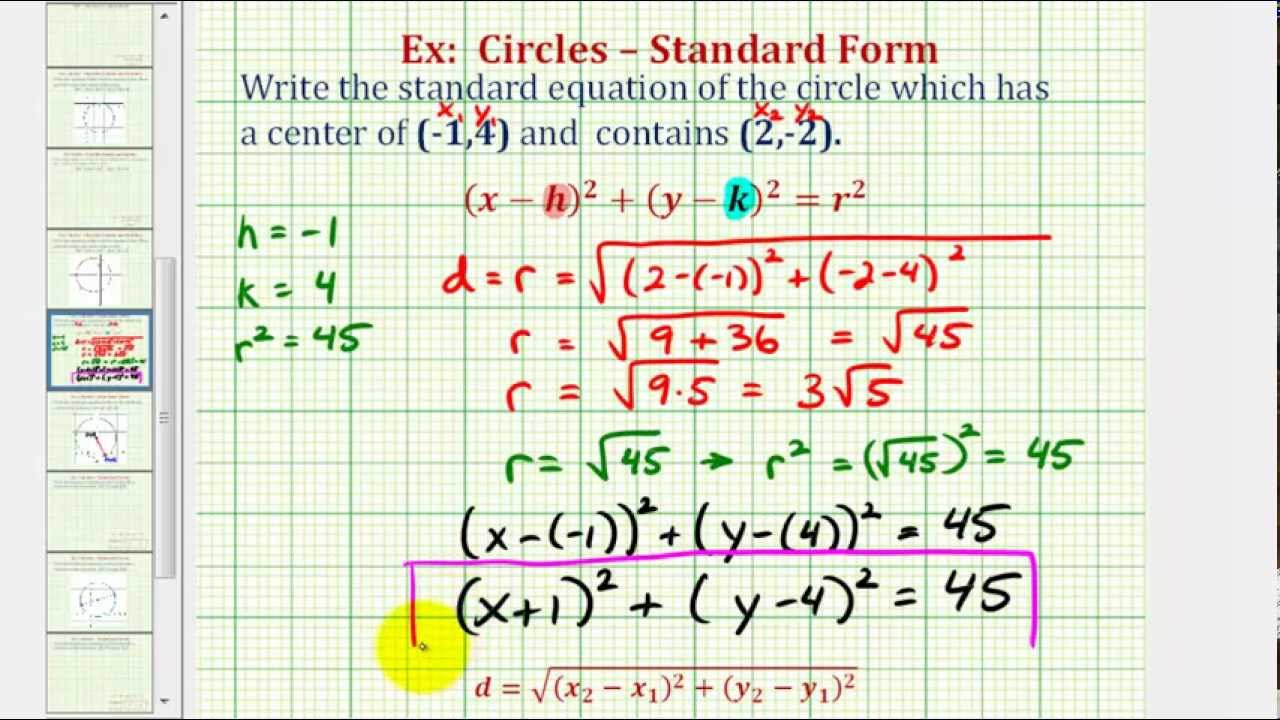
Given parameters are center (a, b) = (4, 5);
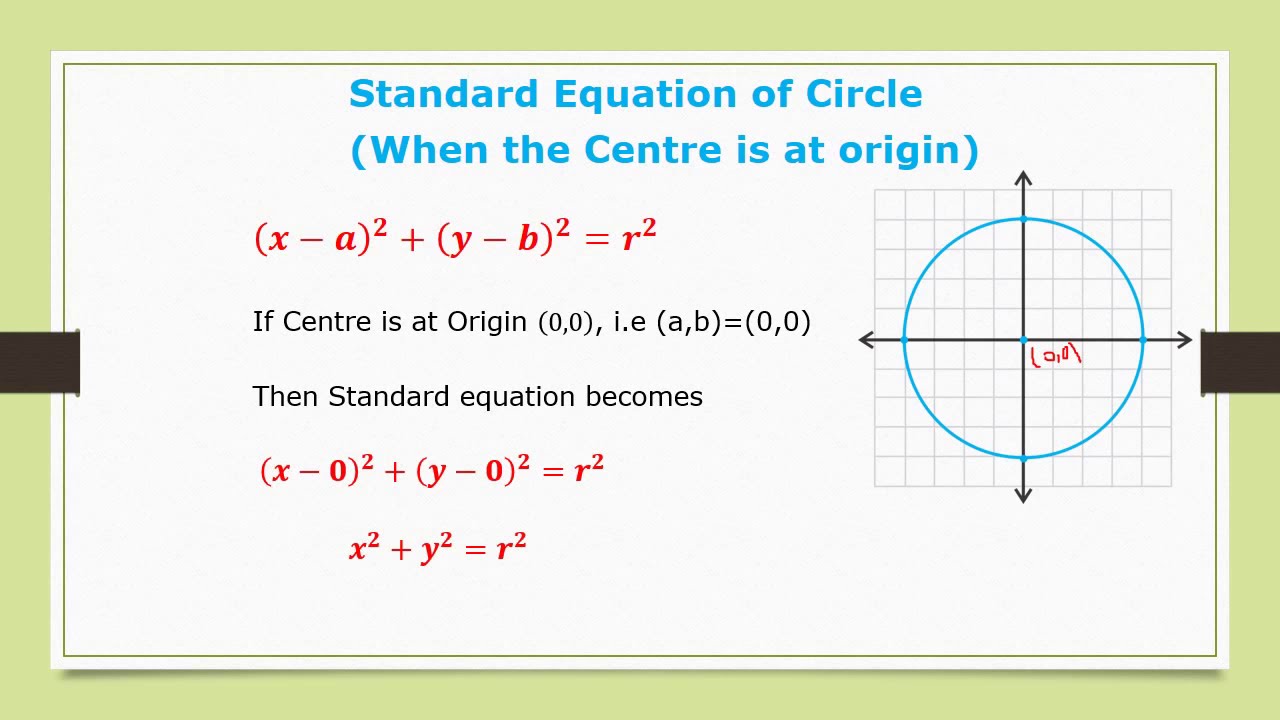
The general equation of any type of circle is represented by: X 2 + y 2 = r 2. Up to 10% cash back with some algebra, we'll multiply both sides by 4 to eliminate the 4's from the left side of equation: X2 + y2 + 2gx + 2fy + c = 0.
We know that the standard equation is.
How to graph a circle 4 easy steps equations examples. Completing the square to write equation in standard form of a circle. The standard form of a circle is that expression we just derived from the pythagorean theorem! Now, we see a few examples of circle equation that include the transformation of the equation from a standard form to the general form.
(x − 9) 2 + (y − 7) 2 = 25 c:
X2 + y2 + 18x + 14y + 105 = 0 a: Write the standard form equation of circle with center: 7 3 equation of a tangent to circle ytical geometry. Let's see some examples based on the standard form.
We cannot use (x, y) ( x, y) for all the graph points, so we use other letters to identify the coordinates of the center of the circle, in this case (a, b) ( a, b):
Convert the equation of a circle in general form below to standard form. Find out the radius and center of a circle from the given equation. So when you see something like that think hmm. 9 rows squaring both sides, we get the standard form of the equation of the circle as:
The calculator will generate a step by step explanations and circle graph.
Adding g2 + f2 on both sides of the equation. To convert the standard equation of the circle into polar form, substitute the value of x with r cos𝜃 and y with r sin𝜃. And we end up with this: X 2 + (y + 3) 2 = 7 2.
To find the number that completes the square for the x group, start with the.
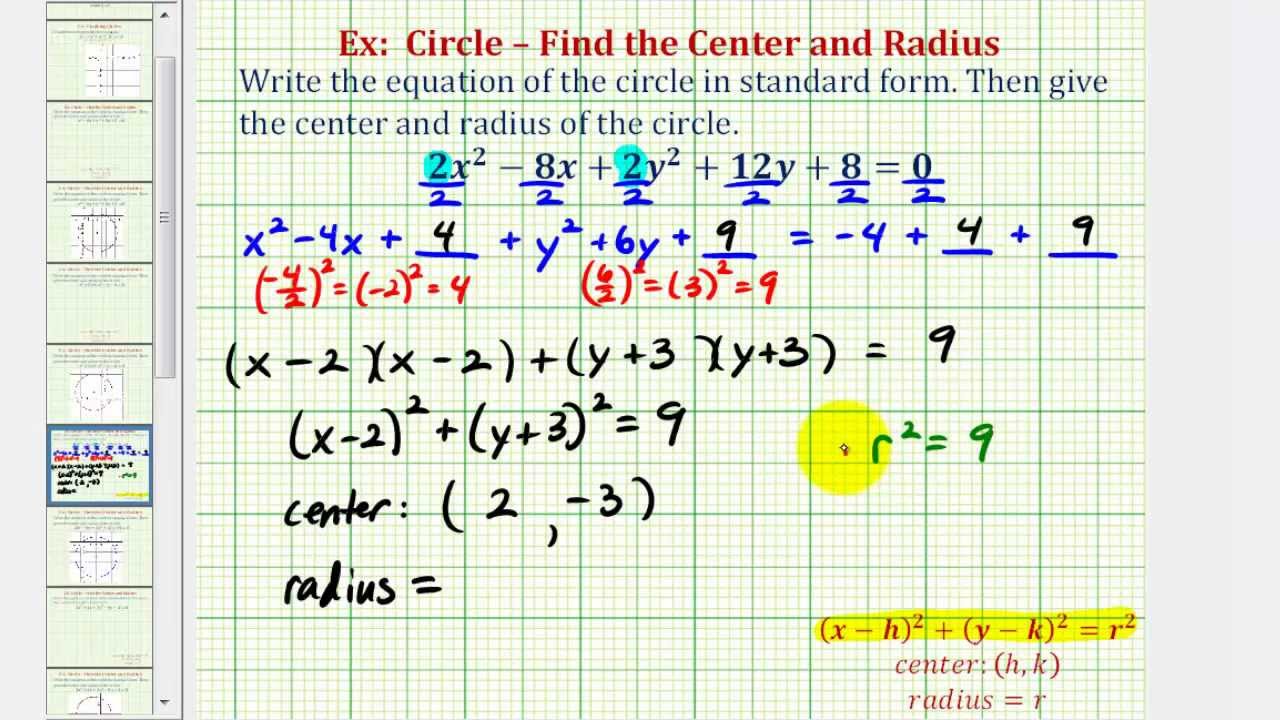
Use completing the square on the group of x terms and the group of y terms. Find the centre and radius? X2 + y2 − 2x − 4y + 1 + 4 − 9 = 0. (x−h)2 +(y−k)2 = r2 ( x − h) 2 + ( y − k) 2 = r 2.
The radius (r) hasn’t been given.
The standard equation of a circle formula everything you need to know mashup math. Radius and center for a circle equation in standard form. Considering a circle with the center located at (2, 3) and radius equal to 100, the standard form of the equation will be: This calculator can find the center and radius of a circle given its equation in standard or general form.
Start by grouping the x terms together, grouping the y terms together and moving the constant to the other side of the equation.