Therefore the equation of circle is= (x − 4)2 + (y − 2)2 = 36. My math teacher taught me that the general form (equation) of a circle is: Pull like terms together, set the equation equal to 0, and we have this:
Question Video Finding the General Form of the Equation
Subtract 49 from each side.
X 2 + y 2 + 2gx + 2fy + c = 0.
This calculator can find the center and radius of a circle given its equation in standard or general form. Where d, e, and f are real numbers. Given parameters are center (a, b) = (4, 5); Find out the radius and center of a circle from the given equation.
We know that the equation of the circle described on the line segment joining ( x 1, y 1) and ( x 2, y 2) as a.
To find the centre and radius of the circle, we first need to transform the. I am really confused on how each of the coefficients change the graph. Consider here an example to find the center and radius of the circle from the general equation of the circle: Write the following equation of a circle in general form.
Also, it can find equation of a circle given its center and radius.
He also asked us this: X 2 + 8x + 16 + y 2 = 49. Note that you have to add same value on both side of the equation. Find the centre and radius?
We know that the general shape of the equation of a circle is x2 + y2 + 2hx + 2ky + c = 0.
To more easily identify the center and radius of a circle given in general form, we can convert the equation to standard form. As given in the question, radius = 4. If the product of c and d is negative, then what 2 quadrants can the graph be in? General form of a circle.
Given the general equation of circle find the coordinates for center of the circle.
For example, if e is negative, the graph would move to the left and reflect across the y axis, so it would be in. X2 + y2 + 18x + 14y + 105 = 0 a: X 2 + 2(x)(4) + 4 2 + y 2 = 49. Find the diameter form of the circle, drawn on the intercept made by the line 2x + 3y = 6 between the coordinates axes as diameter.
X2 + y2 + 6x − 6y + 9 = 0 a:
General form of the equation of a circle examples. Equation of a circle with centre (h, k) and radius r : Now solve for and by completing the square. The radius is 9 because the formula has r 2 on the right side.
Let's expand that so you can more easily see how it turns into the general form:
A x 2 + b y 2 + c x + d y + e = 0. Circles circle equations mathbitsnotebook geo ccss math converting an equation of a from general to standard form key stage 3 review ytic geometry article khan academy in formula practice problems and pictures how express with given radius harder example outcome 4 the mathematics level revision circles circles circle equations mathbitsnotebook. Here a=4, b=2 and r=6. 9 rows squaring both sides, we get the standard form of the equation of the circle as:
Find the equation of the circle, whose centre is at the origin and radius is equal to 9 units.
Represent this as a circle equation ? If the minus sign is preceding the (h, k), (h, k) will be positive. (x + 9) 2 + (y + 7) 2 = 5 b: The circle equation general form is expressed as:
Given general equation of circle.
Equation of the circle in general form : This is called the general equation of a circle. The general equation of the circle is. We take a general point at the boundary of the circle, say (x, y).
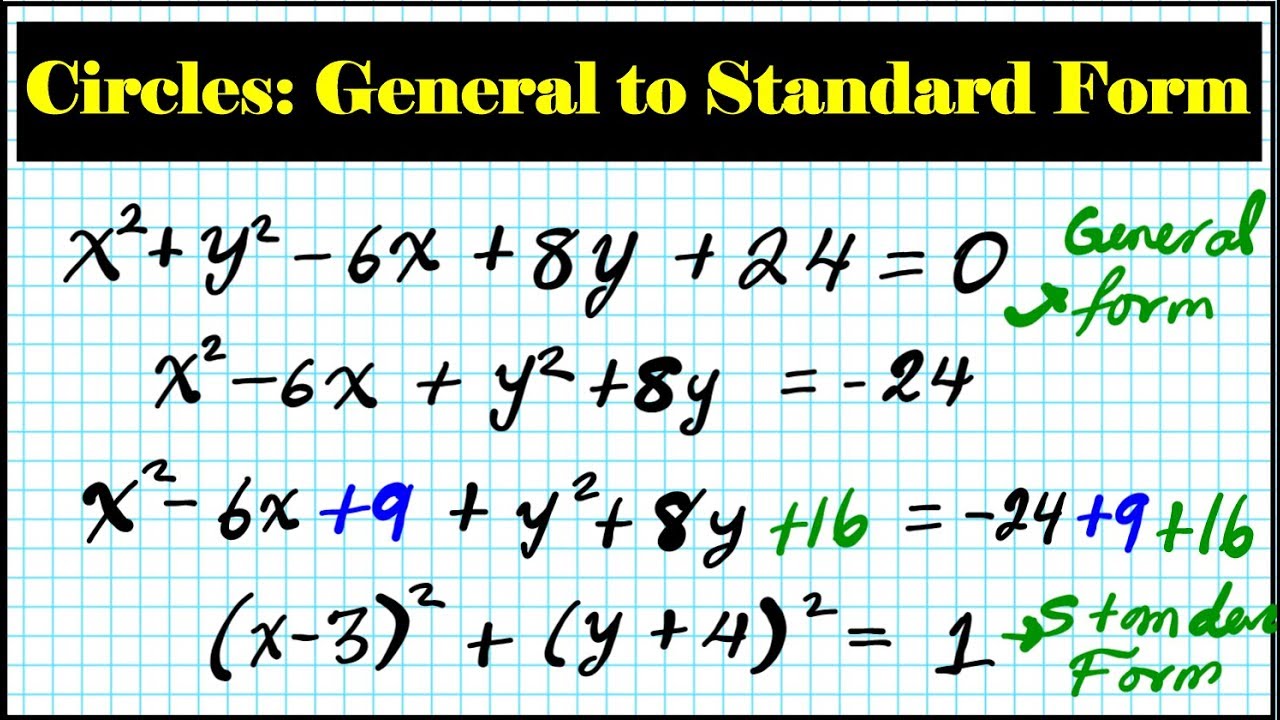
Convert the equation of a circle in general form below to standard form.
(x + 4) 2 + y 2 = 49. The equation of a circle in general form is, x 2 + y 2 + dx +ey + f = 0. X 2 + y 2 + 2gx + 2fy + c = 0. Let's see some examples based on the standard form.
Convert the equation into general form.
X 2 + y 2 + 8x + 16 = 49. Find the centre and radius of the circle with the given equation of a circle. The calculator will generate a step by step explanations and circle graph. The line 2x + 3y = 6 meets x and y axes at a (3, 0) and b (0, 2) respectively.
Compare the given equation with the standard form, we get:
Given centre is at origin that is (0,0) and radius is 9 units. Now, we see a few examples of circle equation that include the transformation of the equation from a standard form to the general form. General form of the equation of a circle :