(x−1)2 + (y−2)2 = 32. Use the example above as a model. Find the diameter form of the circle, drawn on the intercept made by the line 2x + 3y = 6 between the coordinates axes as diameter.
Equation of a Circle Teaching Resources
X2 + y2 − 2x − 4y − 4 = 0.
Example 2 find the equation of the circle whose centre is at the origin and which passes through the point (3, 2).
Therefore, we can find the standard equation of the circle using, therefore, the general equation of circle is where are real constants. And we end up with this: Represent this as a circle equation ? X2 + y2 − 2x − 4y + 1 + 4 − 9 = 0.
The perimeter of the circle is known as the.
Given the general equation of circle find the coordinates for center of the circle. Find the normal to the circle \(x^2 + y^2\) = 0 at the point (1, 2). The standard equation of a circle formula everything you need to know mashup math X 2 + y 2 = 1.5 2 simplify the equation by squaring the radius x 2 + y 2 = 2.25 x 2 + y 2 = 1.5 2 simplify the equation by squaring the radius x 2 + y 2 = 2.25.
Tell whether the point is on the circle, inside the circle, or outside the circle.
(c) if \(x^2\) + \(y^2\) = \(a^2\) is the equation of the circle then at any point ‘t’ of this circle (acost, asint), the equation of normal is. X 2 + y 2 = 2.25 x 2 + y 2 = 2.25 x^2+y^2=2.25 x 2 + y 2 = 2.25. Circle equations harder example khan academy. Let the radius of a circle be 2 and coordinate of the center of the circle is origin i.
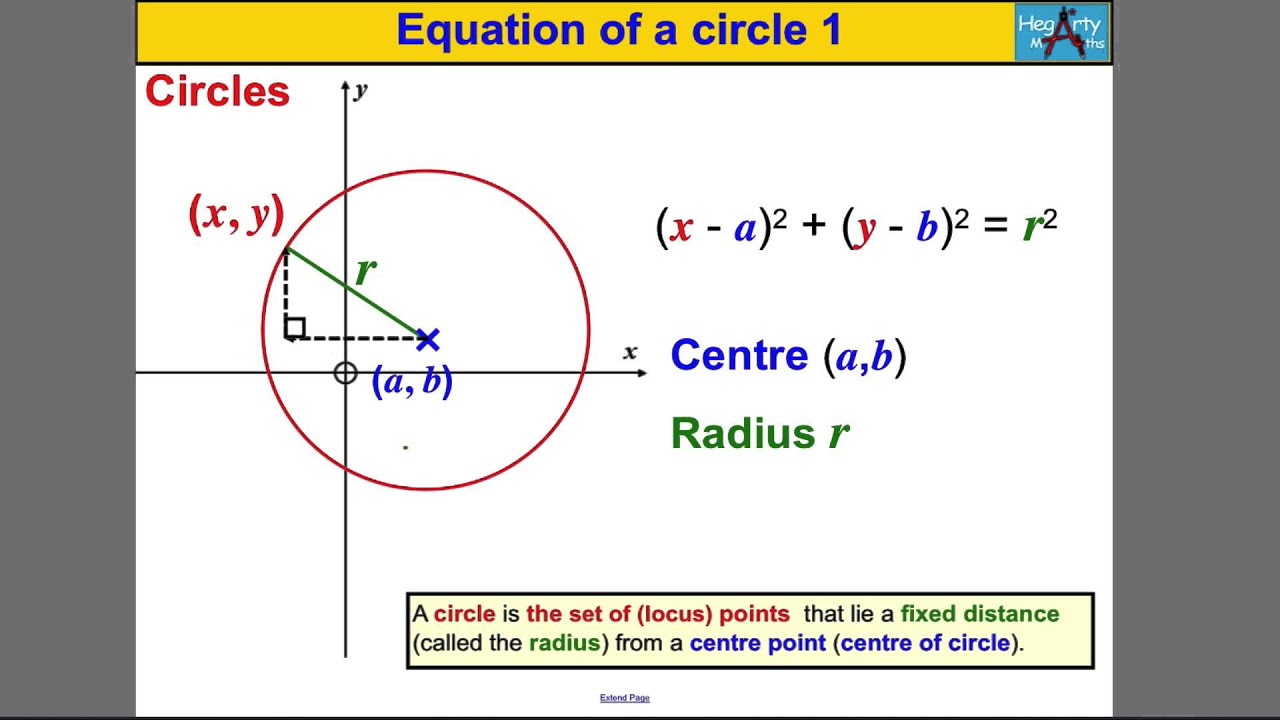
Equation of a circle examples circle on a graph when you consider a circle on a coordinate graph is the set of all points equidistant from a center point, you can see that those points can be described as an ( x , y ) value on the graph.
We know that the standard equation is. 9 rows squaring both sides, we get the standard form of the equation of the circle as: It is a circle with a center at and a radius of. X2 − 2x + 1 + y2 − 4y + 4 = 9.
Find the equation of a circle whose centre passes through the origin.
X 2 + y 2 = r 2. The circle equation general form is expressed as: H and k are the x and y coordinates of the center of the circle. Here, the fixed point is known as the centre of the circle, while the distance between the boundary points and the centre is known as the radius.
Equation of a circle in standard form formula practice problems and pictures how to express with given radius.
If the center point and radius of a circle is given as (4, 5) and 7 respectively. To find the centre and radius of the circle, we first need to transform the equation from general form to standard form. To change the equation from center radius form to general form, you must expand and simplify the equation. The formula is ( x − h) 2 + ( y − k) 2 = r 2.
Another form of the circle equation is called the general form and is written as \(ax^2+by^2+cx+dy+e=0\).
Equation of the circle is: We have, \(x^2 + y^2\) = 0 since normal on any point (\(x_1, y_1\)) of circle \(x^2\) + \(y^2\) = \(a^2\) is \(y\over x\) = \(y_1\over x_1\). It is a circle equation, but in disguise! Is a way to express the definition of a circle on the coordinate plane.
Therefore the equation of circle is= (x − 4)2 + (y − 2)2 = 36.
Z(2.5, 23) cell phones in exercises 36 and 37, use the following information. Equation of a circle mathematics level revision. General form of the equation of a circle examples. Up to 10% cash back the standard form of a circle is given below:
The area of the circle is pi times the square of its radius.
Circle equations questions require us to understand the connection between these equations and the features of circles. Here a=4, b=2 and r=6. The required equation is x 2 + y 2 = 4 2. Circles outcome 4 equations of a circle the.
Solution a slight twist in the previous example.
The radius (r) hasn’t been given. ( x − 9) 2 + ( y − 6) 2 = 100 is a circle centered at (9, 6) with a radius of 10. The line 2x + 3y = 6 meets x and y axes at a(3, 0) and b(0, 2) respectively But it is known that the circle passes through the point (3, 2), which means its coordinates.
Find the equation of the circle, whose centre is at the origin and radius is equal to 9 units.
Radius and center for a circle equation in standard form. Find the centre and radius? Given parameters are center (a, b) = (4, 5); So when you see something like that think hmm.
Given centre is at origin that is (0,0) and radius is 9 units.