General form of the equation of a circle examples. D=2r, where ‘d’ is the diameter and ‘r’ is the radius. A circle had a radius of r unit and a center c (a,b).
Equation of a Circle YouTube
In the above diagram r is the radius of the circle, d is the perpendicular distance from the chord to the center of the circle.
To find the centre and radius of the circle, we first need to transform the equation from general form to standard form.
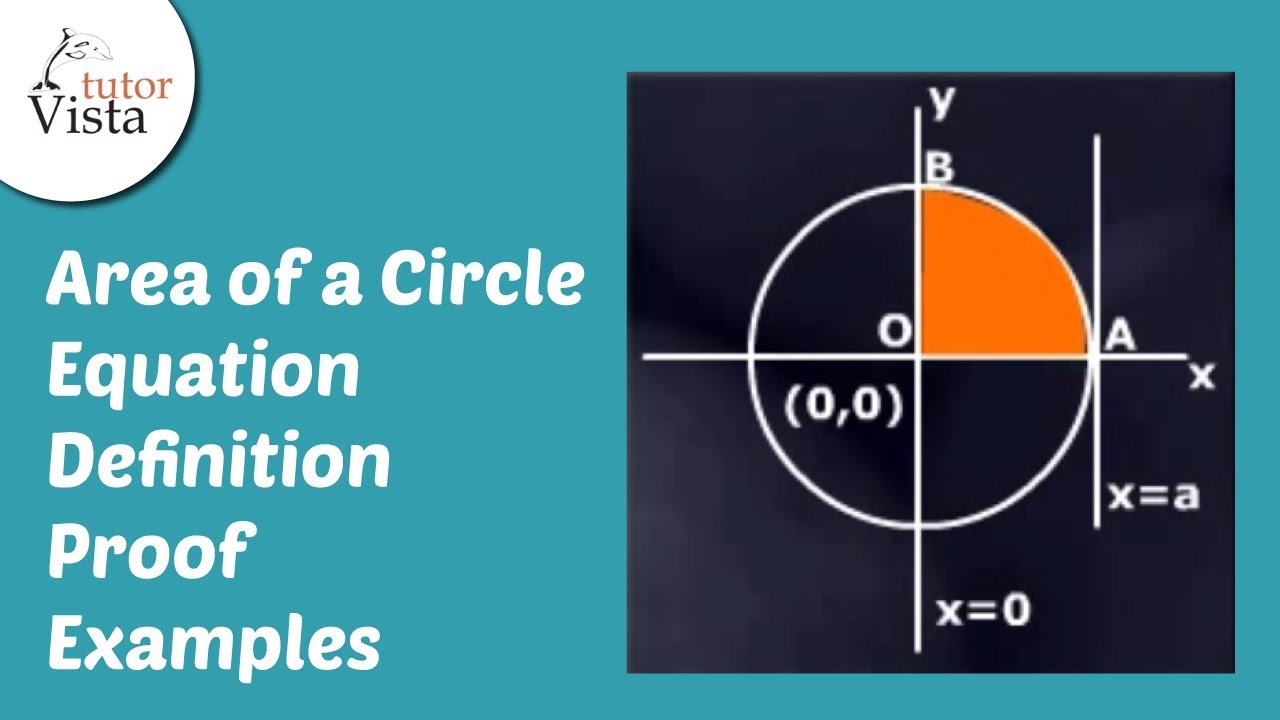
Let’s go back to the cartesian plane. C = πd c = π d. Let the coordinate of a center be (0, 0) and the radius of a circle is r. As we know a perpendicular bisector from the chord to the center of the circle bisects the chord in two halves.
Then, according to the definition of the circle, the distance between p and o will always be equal to ‘r’.
Circumference of circle = 2πr units. And that is the standard form for the equation of a circle! If the circle cuts the origin and both the axis at respective points. (x−(−2))2 +(y−1)2 =32 ( x − ( − 2)) 2 + ( y − 1) 2 = 3 2.
The general equation of a circle is r2 =(x −h)2 +(y − k ) 2 where r is the radius and the point (h, k) is the center of the circle.
Equation of director circle the equation of the director circle of the circle x 2 + y 2 = a 2 is x 2 + y 2 = 2 a 2 Where (h,k) is the center of circle. Let p(x, y) be any point on the circle. To find the centre and radius of the circle, we first need to transform the equation from general form to standard form.
(x−h)2 +(y−k)2 = r2 ( x − h) 2 + ( y − k) 2 = r 2.
A triangle is formed where r will be the hypotenuse of the triangle. If the center of the circle is at the origin of the coordinate plane, the equation is where r is the radius. The ~[ ⇑] written in the form (x−h)2+ (y−k)2=r2 where (h,k) is the center and r is the radius. Diameter of a circle = 2 x radius.
The center (a,b) and the radius r.
D (x,y) is a point on the circle’s circumference, and the equation of a tangent to a circle equation is: Condition for a second degree equation to be a circle. To find its circumference, multiply that measurement times π π: The center is (−2,1) ( − 2, 1), and the radius is 3 3.
This is also known as the center of the circle equation.
The center of this circle is located at ( 2 , 3 ) on the coordinate. Find the equation of a circle with radius=3 and a center (2,. When the center of the circle is at the point ( h, k ), the equation becomes. Use the standard form of a circle.
The equation of a circle is a way to express the definition of a circle on the coordinate plane.
This diameter is twice that of the radius of a circle i.e. That is op = r. Equation of circle whose centre (h,k)and radius r is given (x−h)2+(y−k)2=r2. Where (h,k) is the center of the circle and r is the radius.
In a circle, if the coordinates of the center are (h,k), r is the radius, and (x,y) is any point on the circle, then the center of circle formula is given below:
C = 2 × 22. Radius of circle from area A circle is divided into lower and upper semicircles. By standard equation of circle:
The radius is r, the center of the circle is (h , k), and (x , y) is any point on the circle.
A circle’s equation can be derived from its definition. It is the same idea as before, but we need to subtract a and b: At d, the tangent line ab intersects the circle. So, h = 0, and k = 0;
Find the centre and radius?
If the radius of the circle is 3.5 cm, then find its area. A tangent is a straight line that only touches the circle’s circumference once. (x−a) 2 + (y−b) 2 = r 2. A = 154 cm 2.
General second degree equation in x and y is ax2+2hxy+by2+2gx+2fy+c=0, where a, h, b, g, f and c are constants.
Every curve has an equation. A = (22/7)× 7× 7. The center of circle formula is also known as the general equation of a circle. Find the centre and radius?
Here we have a circle with a given diameter of 12,756.274 kilometers 12,756.274 k i l o m e t e r s:
Therefore, the area and the circumference of the circle are 154 cm 2 and 44 cm, when the radius is 7 cm. From this equation we can find all the information we require to draw the curve and know each one of its properties. It shows all the important information at a glance: Identify the center (h,k) ( h, k), and radius r r.
If the center of a circle is (0, 0), then the equation of the circle is of the form \ (\ x^ {2}+y^ {2}=r^ {2}\), where \ (\ r\) is the radius.
C = π × 12,756.274 km c = π × 12,756.274 k m. We did not select the diameter randomly. C = 2× (22/7) × 7. General form of the equation of a circle examples.
The equation of a circle includes the radius and it is given by:
Radius of a circle = diameter/2 or. C = 40,075.016 km c = 40,075.016 k m.