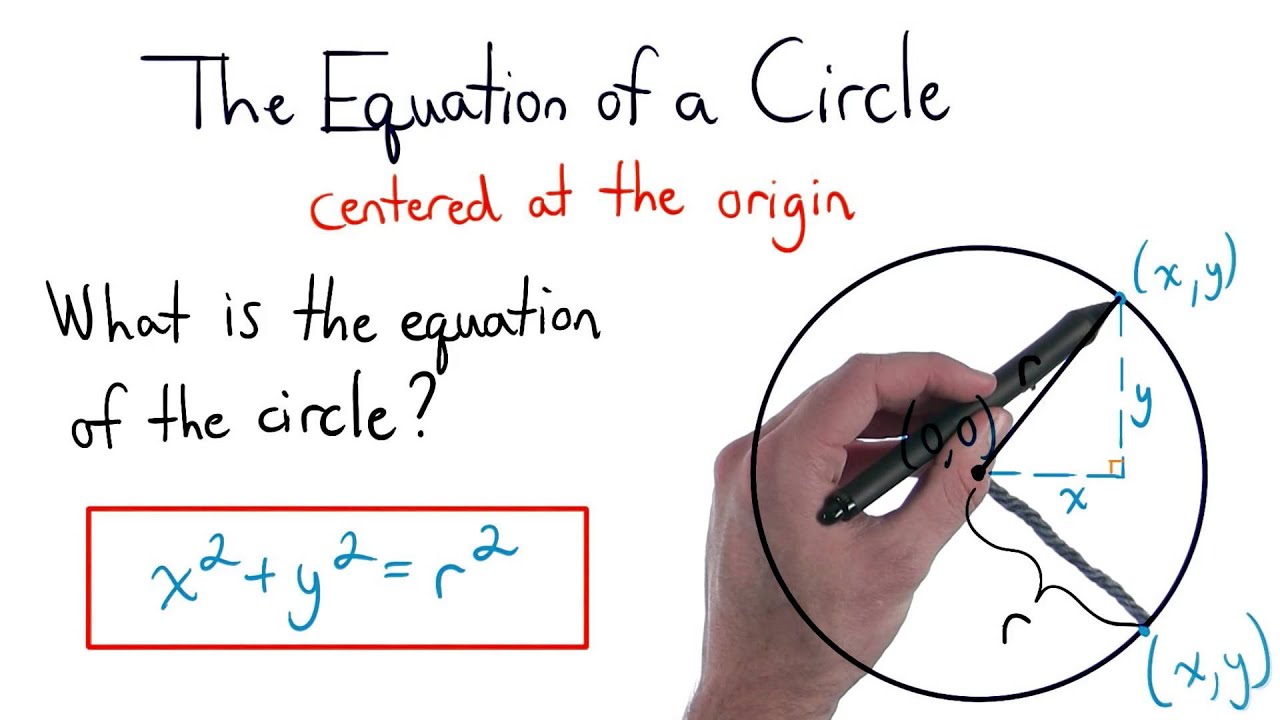
R²=x²+y² click an item in the list or group of pictures at the bottom of the problem and, holding the button down, drag it into the correct position in the answer box. Solution the radius is 4 and the center is at the origin. This is an equation of a circle with center at the origin.
Equation of a Circle with Center at Origin YouTube
It shows all the important information at a glance:
What is the equation of a circle centered?
Equation of a circle with centre at the origin: The polar form of the equation of a circle is usually written for the circle centred at the origin. These two halves are referred to as the semicircle. In the above figure, line ab is called the diameter of the circle.
Substitute the value of x = pcosθ and y = psinθ in the equation.
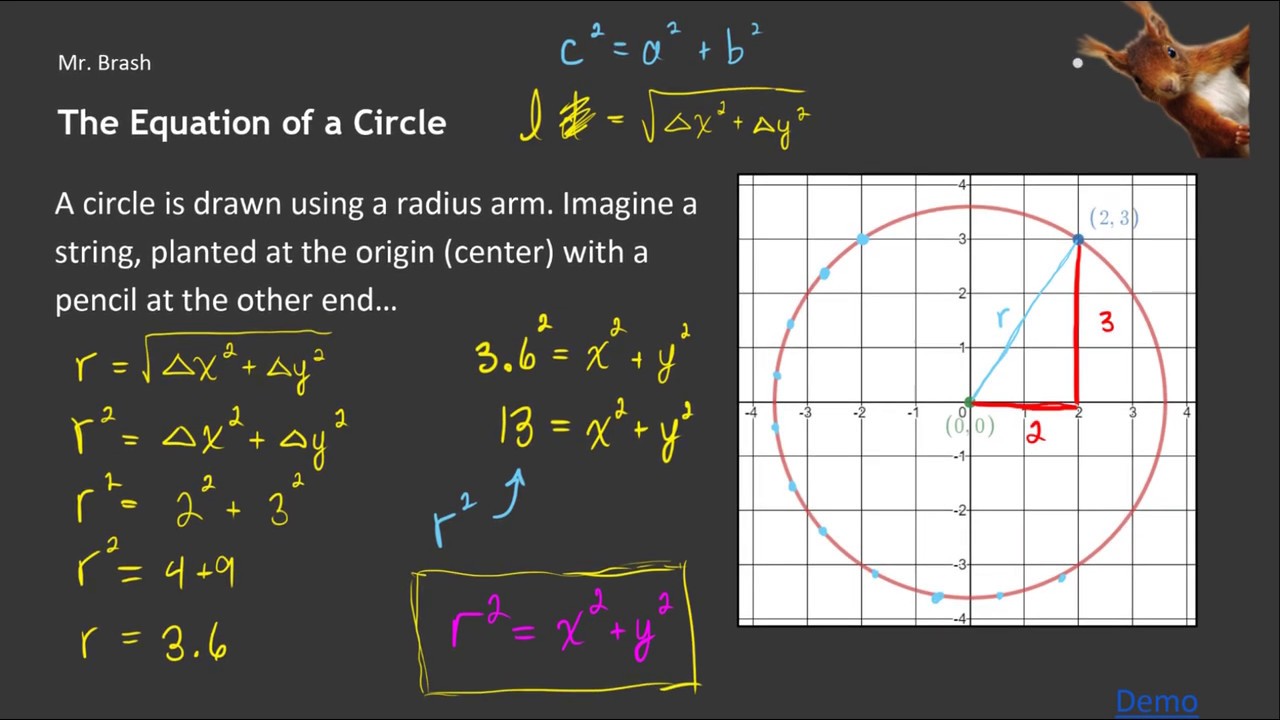
The center (a,b) and the radius r. Given, median of the equilateral triangle is 3a say ldin δlmd, we have(lm) 2=(ld) 2+(md) 2⇒(lm) 2=9a 2+( 2lm ) 2⇒ 43 (lm) 2=9a 2⇒(lm) 2=12a 2again in triangle omd,(om) 2=(od) 2+(md) 2⇒r 2=(3a−r) 2+( 2lm ) 2⇒r 2=9a 2+r 2−6ar+3a 2⇒6ar=12a 2⇒r=2aso, equation of circle is(x−0) 2+(y−0) 2=(2a) 2⇒x 2+y 2=4a 2. The equation x^2+y^2=25 defines a circle at the origin and radius of 5. The general equation is x 2 + y 2 = r 2, so the equation when r = 4 is:
Consider the point p (rcosθ, rsinθ) on the boundary of the circle, with an r radius.
Therefore, the equation for the circle centered at the origin with a diameter of 6 is {eq}\mathbf{x^{2} + y^{2} = 9} {/eq}. X2 1 y2 5 r2 x2 1 y2 5 42 substitute 4 for r. What is the equation of the circle centered at the origin with radius 4? Circle centered at the origin.
What is the equation for a circle centered at the origin?
0) and radius r r, then the equation of the circle is: Y) is a point on a circle with centre o(0; How to write the equation of a. If p (x;y) p ( x;
The diameter divides the circle into two halves such that they are equal in area.
X 2 + y 2 = r 2. Equation of a circle (centre origin) linear equations of the form yx= mx+ c, for example y = 2 + 1, will always be a straight line when plotted on a graph paper. The equation of a circle, centered at the origin, is x2+y2=r2, where r is the radius and (x, y) is any point on the circle. X2 1 y2 5 16 simplify.
Help us to improve mathematics monster.
The standard form of the equation of the circle with center at the origin and a radius of {eq}1 {/eq} is given by {eq}\underline{\hspace{0.5in}} {/eq}. X2 + y2 = r2 x 2 + y 2 = r 2. X 2 + y 2 = 16. (x − 0) 2 + (y − 0) 2 = r 2 x2 + y2 = r2.
It can be expressed in algebraic form, based on the geometrical relation between the circle and cartesian coordinate system.
This is the general equation of a circle that is centered at the origin. It also follows that any point not on the circle does not satisfy this pair of equations. Equation of circle centred at the origin is: A circle can be passed through the origin of the cartesian coordinate system.
Basic equation of a circle.
(center at 0,0) a circle can be defined as the locus of all points that satisfy the equation. From the equation you can also tell the gradient of the line and the point the line crosses the yaxis. So in general we can say that a circle centered at the origin, with radius r, is the locus of all points that satisfy the equations. It is the same idea as before, but we need to subtract a and b:
X 2 + y 2 = 4 2.
The line y = x + 1 passes through the circle. And that is the standard form for the equation of a circle! (x−a) 2 + (y−b) 2 = r 2. 11.7 equations of circles write an equation of the circle.
Answer © an equation of the circle is x2 1 y2 5 16.
X2 + y2 = r2. We are told that r = 4. 2mg(s)+o2(g)→2mgo(s) when 10.1 g of mg are allowed to react with 10.5 g of o2, 14.5 g of mgo are collected. What are the point(s) at which the line intersects the circle?
Here, you learn how to develop an equation of a circle in algebraic form expression when the circle passes through the origin of the cartesian.
X 2 + y 2 = r 2. If it has a radius r, the equation is: This is the equation for a circle centered at the origin. Note that when a = b then f = 0 it means that the ellipse is a circle.
Where x,y are the coordinates of each point and r is the radius of the circle.
To complete the square for the x terms, add 4 to both sides. Write an equation of an ellipse in standard form with the center at the origin and with the given characteristics: X = r cos (t) y = r sin (t) for all values of t. {eq}x^2 + y^2 = 1 {/eq}
The balanced equation for the reaction is:
Form an ellipse and its value is: Is polar equation of a circle with radius r and a center at the pole (origin). Write an equation of a circle with center at the origin.