Work done on elastic springs, and hooke's law. Answer in units of mj. An apple weighs about `1\ n`.
PPT 11/14 do now PowerPoint Presentation, free download
Provided inelastic deformation has not happened, the work done is equal to the elastic.
Let the spring be stretched through a small distance d x dx d x.
The formula for work done by a spring is: Therefore, work = (1/2)kx 2 = (1/2) (100 n/m) (4 m) 2 = 800 joules. It is expressed in newton per meter (n/m). Viewgraphs viewgraph 1 viewgraph 2 viewgraph 3 viewgraph 4
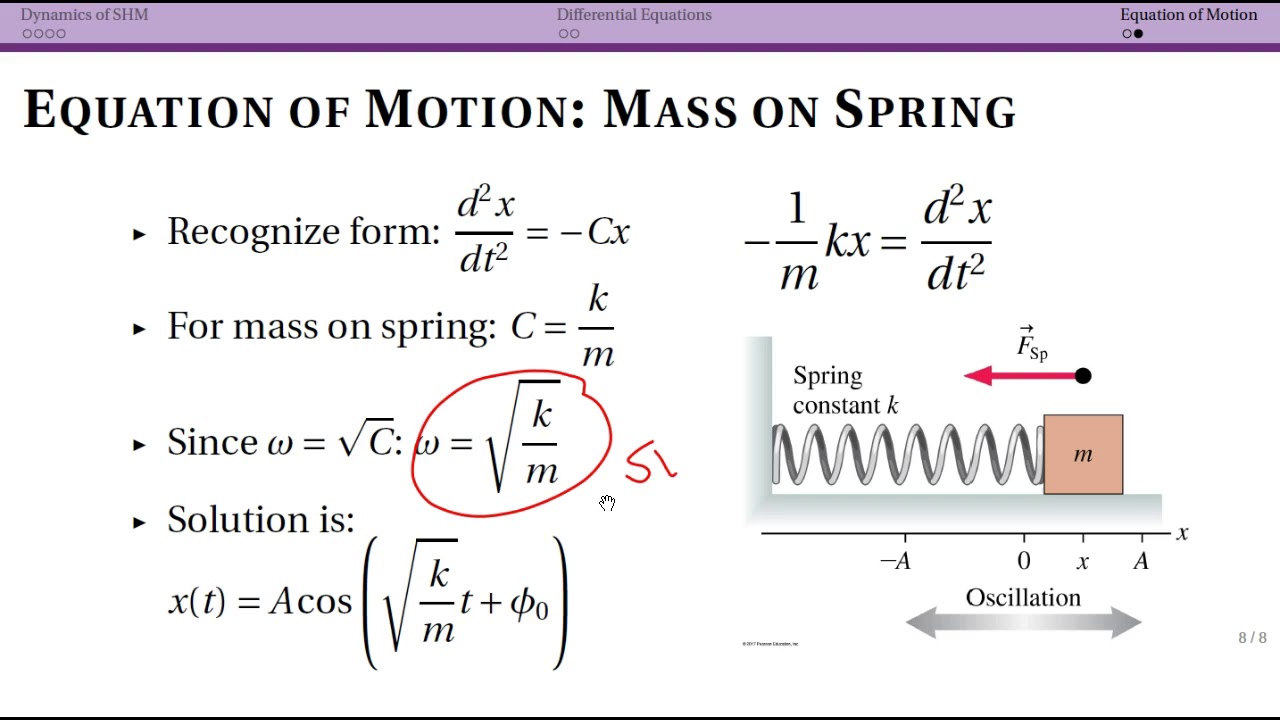
When a spring pulls something, or pushes something, over a distance x, it does work 2 work = 1/2 * k * x if a spring is compressed (or stretched) it stores energy equal to the work performed to compress (or stretch) it.
When the spring is stretched by an external force, the work done by the spring, \[w = \dfrac{1}{2}k{x^2}\] where \[k\]=the force constant of the spring, \[x\]= the extended or stretched of the spring. Compressing a spring) we need to use calculus to find the work done. For this hooke's law force of f ext = k x, the work done to the spring by the external force f ext is. Work done by a variable force.
Work is done when a spring is extended or compressed.
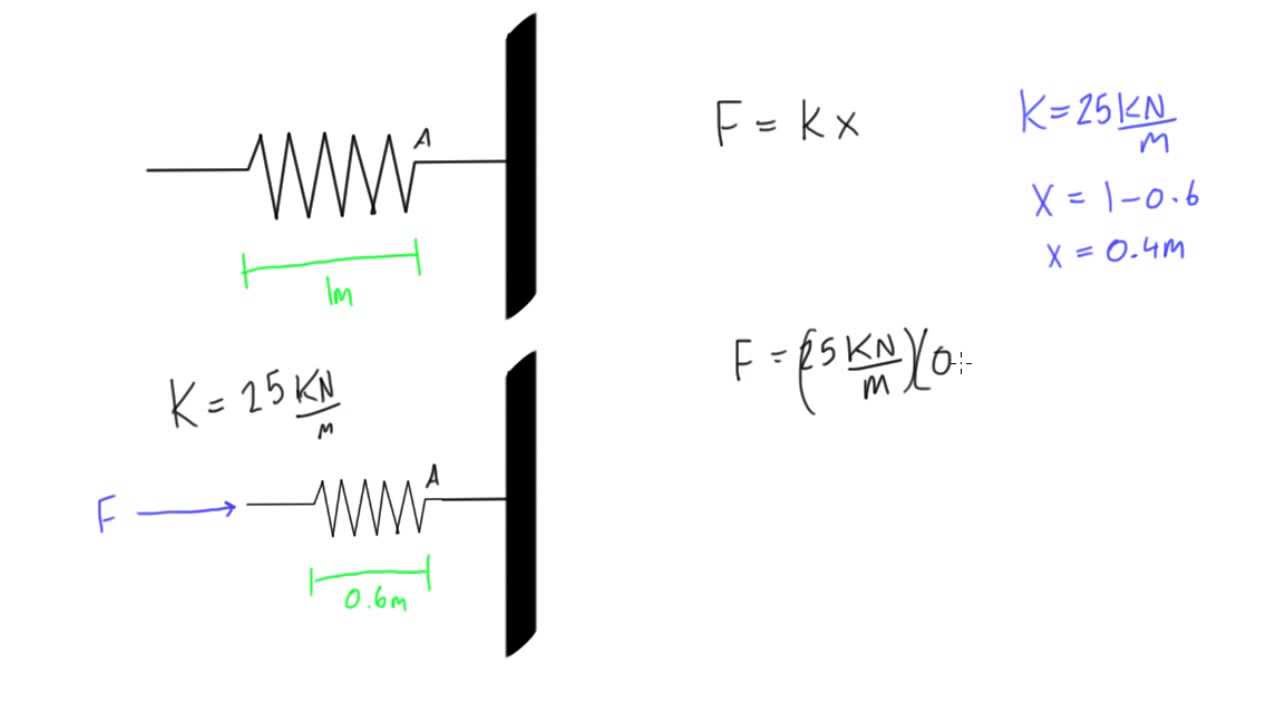
F = −α x + β x3 , where α = 12 n/m and β = 890 n/m3. Plugging the first equation into the second yeilds: A spring is stretched 60 cm, and it takes a force of 40 newtons to hold it there. The spring constant formula is given as, k = − f x where, f = force applied, x = displacement by the spring.
Example of work done by a constant force.
The displacement x is measured from the undisturbed position of the spring (that is, x=0 when f=0). F=kx where k is the spring constant. In case 2, the force in the spring is still f = k x i, but it is being displaced to the right (so dx is negative), and the work done by the spring is then. We might call this spring potential energy.
Then work done in stretching the spring through a distance d x dx d x is d w = f d x, dw=fdx, d w = f d x, where f f f is the force applied to stretch the spring.
The work done on a spring calculator computes the work (w) to further elongate or compress a spring based on the spring constant (k) and the initial and final positions of. When a force is applied on a spring, and the length of the spring changes by a differential amount dx, the work done is fdx. Where w w w is the work done, f ( x) f (x) f ( x) is the force equation, and [ a, b] [a,b] [ a, b] is the distance over. F = − k x.
W = (1 / 2) k x 2.
W = ∫ x 1 x 2 − k x ⋅ d x = 1 2 k x 1 2 − 1 2 k x 2 2. Calculate the work done by the spring when it is stretched from its equilibrium position to 0.15 m past its equilibrium. The work required to stretch or compress a spring. If you lift the apple `1\ m` above a table, you have done approximately `1\ newton meter (nm)` of work.
This means that work is being done on the spring.
To calculate the work done when we stretch or compress an elastic spring, we’ll use the formula. The minus sign indicates that the spring force is always opposite in direction from the displacement of its free end. Where x is the distance from the equilibrium point of the spring. W = ∫ a b f ( x) d x w=\int^b_af (x)\ dx w = ∫ a b f ( x) d x.
$$ pe_{spring} = \frac{1}{2}k(\delta x)^2 $$ if i understand the question correctly, your $\delta x$ is the 30 centimeters, or 0.3 meters.
How much work is done stretching the spring to x = +2.0 m from its equilibrium position at x = 0.0 m? The force exerted by a spring is. At a certain point, when a spring is stretched near its elastic limit, the spring force satisfies the equation. For linear elastic springs, the displacement x is proportional to the force applied:
Let's start with the derivation of the above equation.
Elastic potential energy is stored in the spring. If the force varies (e.g. W = ∫ x 1 x 2 f ⋅ d x. The constant k is called the spring constant and is a measure of the stiffness of the spring.
The force f exerted by a spring is.
The negative sign tells that the visualized spring force is a restoring force and acts in the opposite direction. W = ∫ 0 x o f ⋅ d s = − k x 0 2 2. We have k = 100 n/m and x = 4 m. This work done on the spring as it is stretched (or compressed) can be recovered.