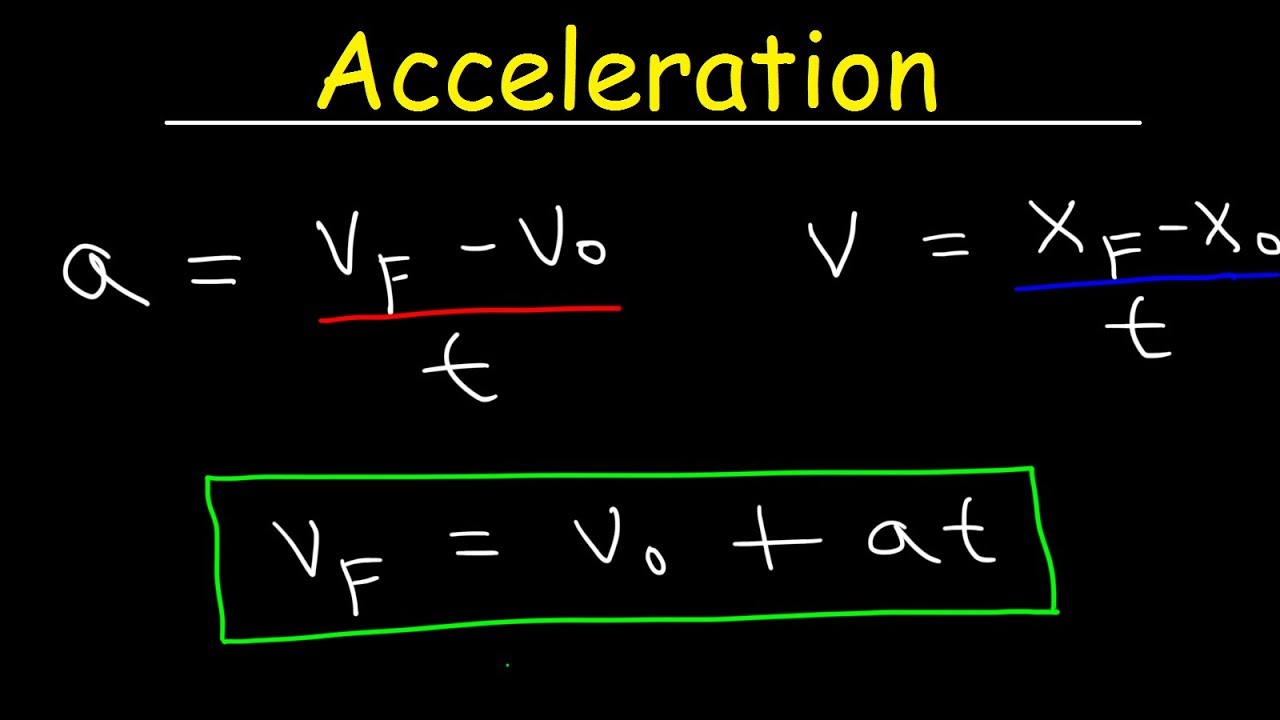
V = v o + at (1) x = x o + v o t + ½ a t 2 (2) using eq. Here is a sample problem and its solution showing the use of this equation: V 2 = v 0 2 + 2 a ( x − x 0) or sometimes written as, v 2 = v 0 2 + 2 a d.
ap2.4 motion with constant acceleration YouTube
V is the initial velocity.
Introduction to the displacement and acceleration equation it reads:
Acceleration as a function of velocity:.or… v a(v) = dv dt t ∫ 1dt = ∫ 1 a(v) dv t = ∫ 1 a(v) dv a(v) = v dv dx x ∫ T is the time of travel. 6 rows a popular way to display the constant acceleration formula is to make speed a function of time. Definition of constant velocity motion
The formula for the distance traveled with constant acceleration is:
The equations of straight line motion with constant acceleration are equation \eqref{2}, equation \eqref{6}, equation \eqref{8} and equation \eqref{9}. X = x 0 + v 0 t + 1 2 a t 2 or sometimes written as, d = v 0 t + 1 2 a t 2. The concept of constant velocity motion; Displacement equals the original velocity multiplied by time plus one half the acceleration multiplied by the square of time.
The constant velocity motion, also known as uniform rectilinear motion (u.r.m.), is the one with constant velocity, i.e., the trajectory is a straight line and the speed is constant.in this section we are going to explain:
Combining these two statements gives rise to a third — one that is independent of time. Here, v 0 is the initial [ [velocity]] of the particle and. V ( t) = ∫ a d t + c 1 = a t + c 1. Velocity is the rate at which an object moves.
Constant acceleration equations for an object that has an initial velocity u and that is moving in a straight line with constant acceleration a, the following equations connect the final velocity v and displacement s in a given time t.
V is the final velocity of the particle. From the relation between velocity, acceleration, distance, and time, we have the equation of velocity. (constant acceleration) 0 ( ), 0 0 and t t t v t v by definition acceleration a o t v t v a o the velocity is increasing at a constant rate v 0 v t v(t) v o at (1) velocity equation since a=const, v is a straight line and it doesn’t matter which acceleration to use, instantaneous or average. V = vo + at (here u = vo ) in both the approaches the terms mean as given.
Hence, first equation of motion can be given as follows:
But if you do have a constant acceleration like this, you can assume that the average velocity is going to be the average of the initial velocity and the final velocity. Velocity is directly proportional to time when acceleration is constant (v ∝ t). These equations of motion of a moving particle with constant acceleration a are. V = u + a t {\text{v}} = {\text{u}} + at v = u + a t.
In a race, the racer rides the bike with an initial velocity of 9 m/s.
Solving for vxf we get an expression for the nal velocity in terms of the initial velocity, the acceleration, and the time vxf = vxi +axt: In constant acceleration, since the acceleration is constant, it can be represented as below: When a velocity is changing as a result of a constant acceleration, the average velocity can be found by adding the initial and final velocities, and dividing by 2. The second approach of how to find velocity with acceleration and initial velocity is by using the central equation of motion.
X is the initial distance.
If this was a curve or if the acceleration was changing, you could not do that. The second equation of motion is as below: If the initial velocity is v (0) = v0, then. Velocity as a function of displacement:
These equations are valid only when the acceleration is constant.
Acceleration as a function of displacement: It has both a magnitude (a value) and a direction. In part (a) of the figure, acceleration is constant, with velocity increasing at a constant rate. These equations relate displacement, velocity, acceleration, and time, and apply for constant acceleration in the +x direction starting at t=0.
A is the constant acceleration.
V ( t) = ∫ a d t + c 1 = a t + c 1. The equations of this motion; Displacement is proportional to time squared when acceleration is constant ( ∆ s ∝ t 2 ). Dx is the distance traveled under constant acceleration.
(1) the average velocity can be written as vx = 1 2 (vxi.
V = u + at. For example, if you steadily increase your velocity (that is, with constant acceleration) from 30 to 60 km/h, then your average velocity during this steady increase is 45 km/h. V = u+at (1) s = 1 2 (u+v)t (2) s = ut+ 1 2 at2 (3) s = vt− 1 2 at2 (4) v2 = u2 +2as (5) The equation [latex]\bar{v}=\frac{{v}_{0}+v}{2}[/latex] reflects the fact that, when acceleration is constant, v is just the simple average of the initial and final velocities.
With a ( t) = a a constant, and doing the integration in equation 3.18, we find.
V 0 = 0 + c 1. After time t, the velocity changes, and the acceleration is 3 m/s2. To find the velocity of the motor vehicle, v = 32.31 m/s. V = v 0 + a t.
If we let ti = 0 and tf = t, we get ax = vxf vxi t:
We can derive the kinematic equations for a constant acceleration using these integrals. An object is moving with a velocity of 5.0 m/s.