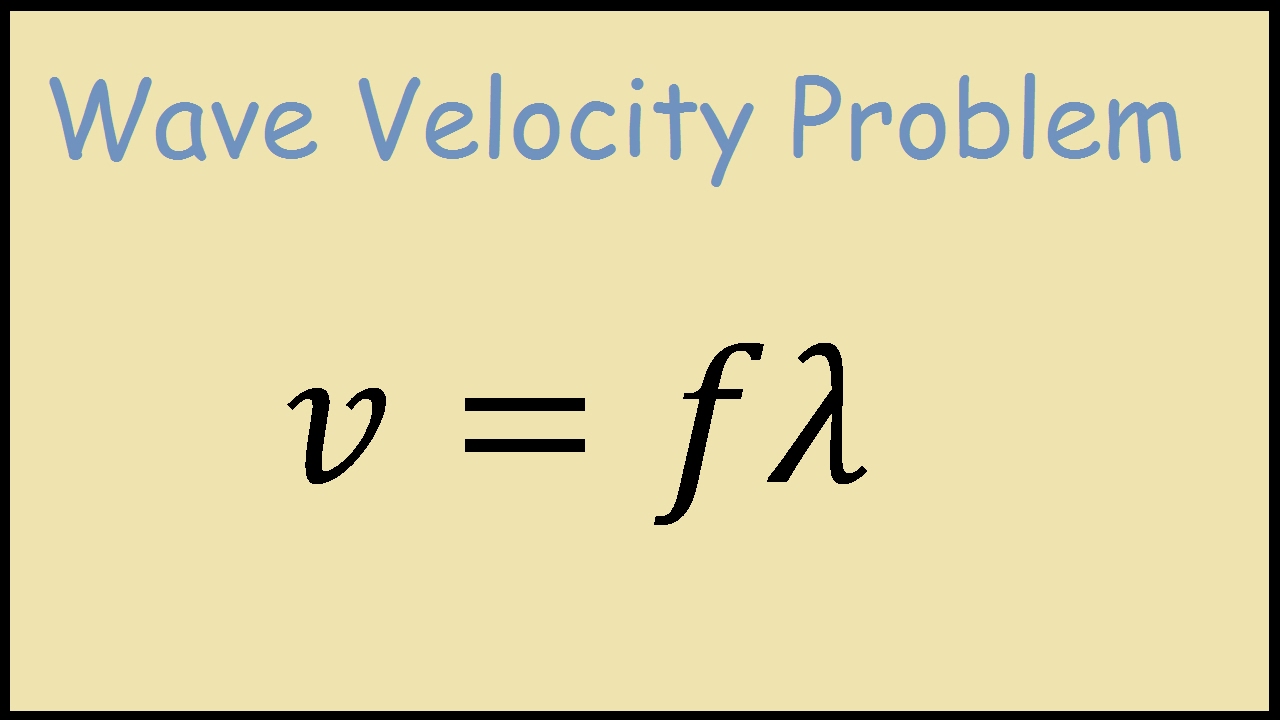Wavelength λ = 600 nm, velocity of light v = The wave velocity is also known as wave speed. C = / k the velocity is the wavelength / period:
What is the speed of a wave measured in
A transverse wave is described by the equation y y0 sin 2 pie ft x lemda maximum particle velocity four times if sahay lms.
Phase velocity of a point a of the wave.
In this case how would i find the wave velocity? The velocity is distance between a and b per time. A light wave travels with a wavelength of 700 nm. The frequency is calculated by, f = v / λ
Mathematically it is given by, \[\rightarrow v = \lambda\nu \]
V is the transverse velocity of the wave; So generally, e x (z,t)= f [(x±vt)(y ±vt)(z ±vt)] in practice, we solve for either e or h and then obtain the. Velocity is a reference distance divided by a reference time. Where, v is the velocity of the wave;
The wave speed formula which involves wavelength and frequency is given by:
C = / = f z the wave moves one wavelength, , in one period,. The wave formula for the velocity of sound is given by, v = f λ. Wavelength λ = 700 nm, velocity of light v = 3 x 10 8 m/s. Energy, power & intensity of a wave :
Δ(b) = mu0*eps0*(d^2 b/dt^2) is satisfied.
Where, v = velocity of the wave, f = frequency of the wave, λ = wavelength. It states the mathematical relationship between the speed (v) of a wave and its wavelength (λ) and frequency (f). E x t b kx t c kx t The transverse velocity of a wave equation is:
The velocity of the wave can be calculated by knowing the wavelength of the wave and the frequency of the wave.
A transverse wave is represented by the equation y 0sin 2pi lamda vt x for what value of maximum particle. Show transcribed image text expert answer. The derivation of the wave equation certainly varies depending on context. Maxwell deduced that the speed of propagation of an electromagnetic wave through a vacuum is entirely determined by the constants μ 0 and ϵ 0 as the following:c= μ 0 ϵ 0 1 we know that μ 0 =4π×10 −7ns 2c −2 and ϵ 0 =8.854×10 −12c 2n −1m −2 which gives:c= 4π×10 −78.854×10 −12 1 =2.998×10 8 m/s.
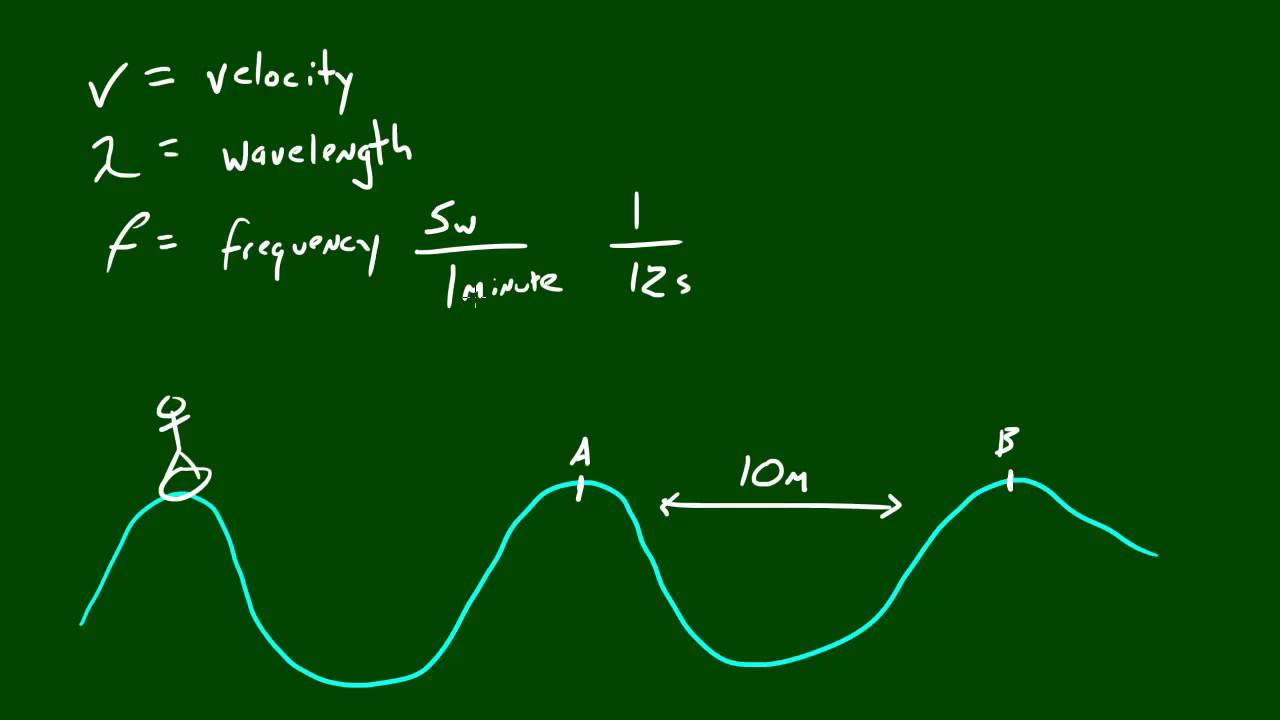
So consider any point on the wave, for example the top of a wave crest (point a in illustration 1).
This distance corresponds by definition to the wavelength \(\lambda\). Which is exactly the wave equation in one dimension for velocity v = t μ v = \sqrt{\frac{t}{\mu}} v = μ t. The solution represents a wave travelling in the +z direction with velocity c. The velocity how fast is the wave traveling?
Usually, f(x+ t) is called a traveling wave to the left with speed 1;
The above equation is known as the wave equation. Other field using the appropriate curl equation. Gravity wave, deep water √ g k g k 1 2 g k 1 2 g = acceleration of gravity gravity wave, shallow water √ g k tanhkh g k tanhkh cp·(cg/cp) 1 2+ kh sinh(2hk) h = water depth capillary wave √ t k3 √ t k 3 t k 2 3 2 t = surface tension quantum mechanical particle wave hk2 4πm hk 4πm hk 2πm 2 h = planck’s constant m = particle mass cg = particle velocity F is the frequency of the wave;
V = f × λ where, v = speed of f = frequency λ = wavelength.
University physics with modern physics. K is the wave number; A simpler equation for a harmonic wave: V = f • λ
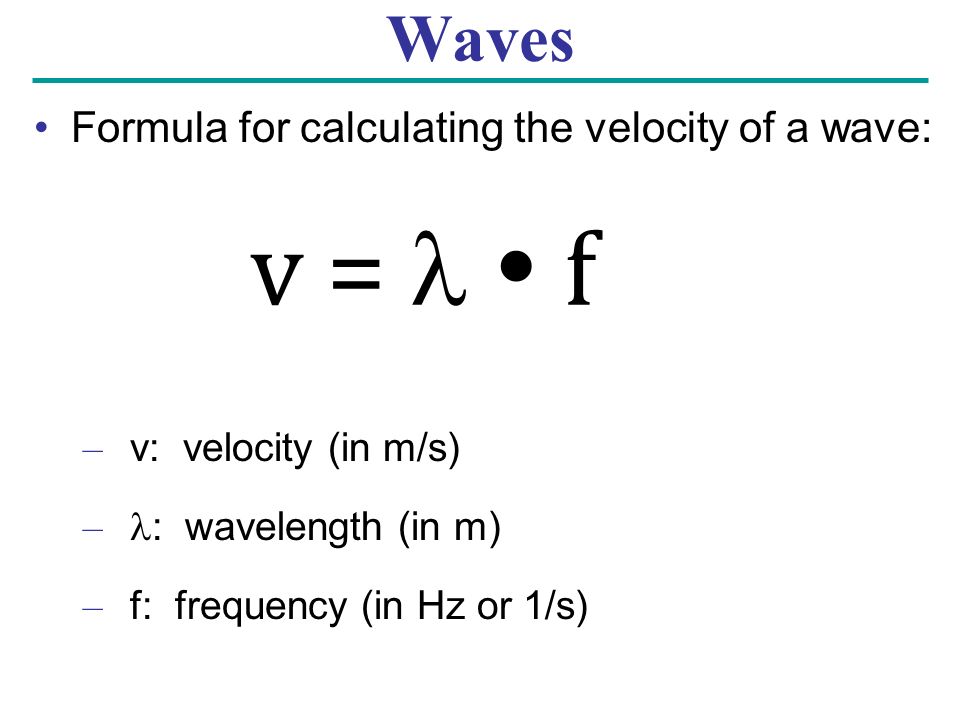
Speed = wavelength • frequency.
G(x t) is called a traveling wave to the right with speed 1. The wavelength λ =10.0 m and the period t=5.00s, are given and we need to find v. Therefore, the equation or formula can rewritten as. = 1 2 ρ ( δ y δ t) 2.
We can calculate wave velocity with the help of below formula:where,v = wave velocity (m/s)f = wave frequency (sec)w = wavelength (meters)enter the wave frequency and wavelength in the below online wave velocity calculator and click calculate button to find the wave velocity.
Using the symbols v, λ, and f, the equation can be rewritten as. For a free spring, the wave velocity is v = √(k / m) x l, where l is the displacement of one end right? Ω is the angular frequency; Young, hugh and freeman, roger.
It gives the mathematical relationship between speed of a wave and its wavelength and frequency.
Below, a derivation is given for the wave equation for light which takes an entirely different approach. Many derivations for physical oscillations are similar. We want to find out how fast this point moves from a to b. Similarly, f(z+vt) is a solution as well.
T) sin(θ) which is the same result as before, as long as:
Formula that allows you to calculate the phase velocity of a wave, given the wavelength and period. Calculate the wave velocity of the ocean wave if the distance between wave crests is 10 m and the time for a ball floating in surface to bob up and down is 5 sec. Rearranging the equation yields a new equation of the form: I see some sources state that the equation i mentioned above is applicable.
A light wave travels with a wavelength of 600 nm.
Thus the wave equation does not have the smoothing e ect like the heat equation has.