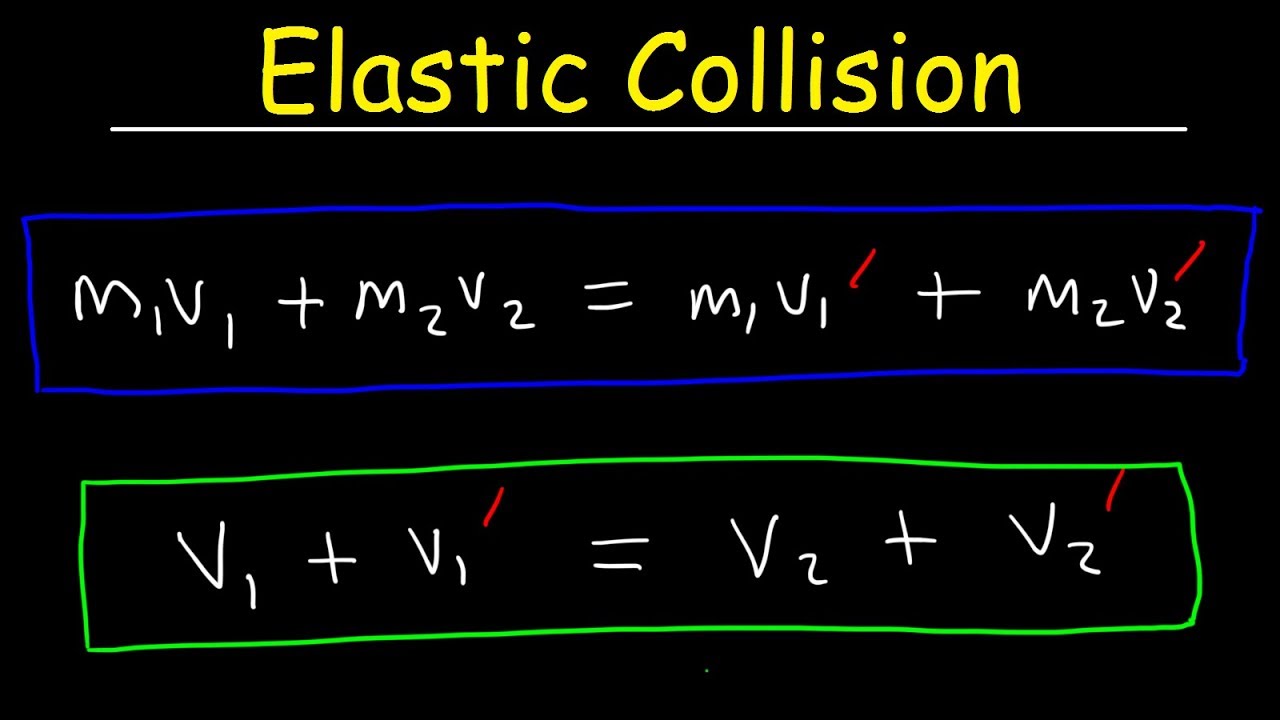
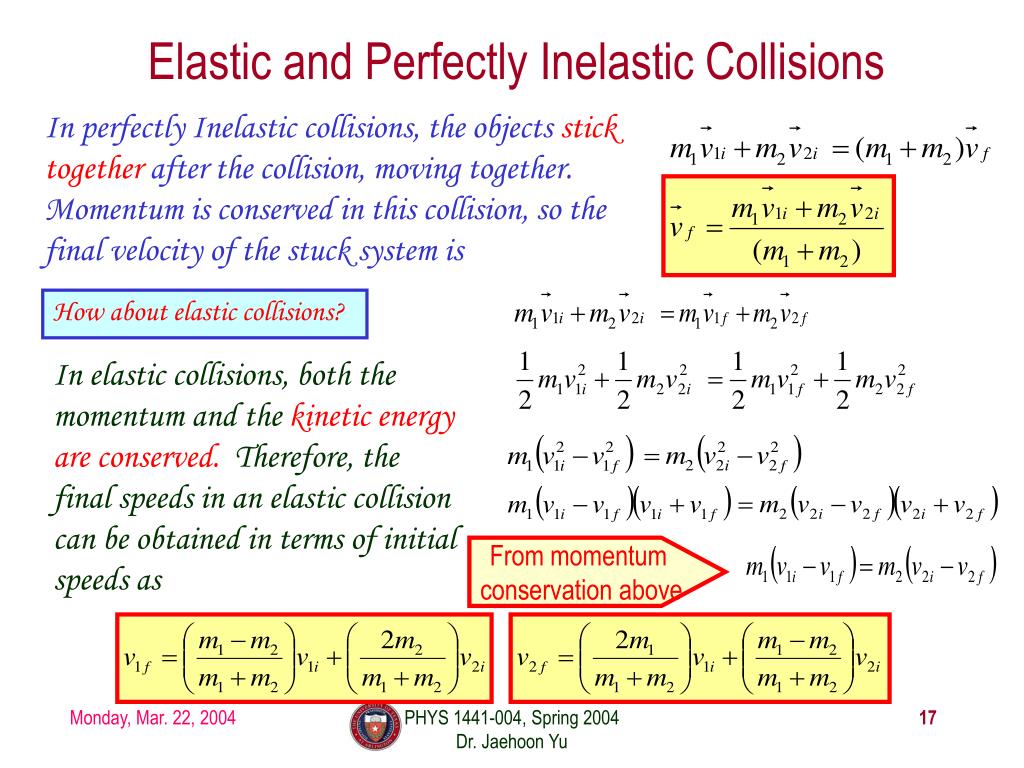
Substitute the given quantities into the equation. If the collision is elastic, both objects move away from each other with different velocities v 1, v 2 in opposite directions. The inelastic collision formula is articulated as.
PPT Chapter 9 PowerPoint Presentation, free download
V2 =1.2 / 0.20 = 6 m/s.
The elastic collision formula is given as.
A simple example of elastic collision is the striking of balls when striking with the stick while playing pool or snooker. Learn about what's conserved and not conserved during elastic and inelastic collisions. P 1x + p 2x = p ′ 1x + p ′ 2x. The formula for inelastic collision:
The value of e > 0 or e < 1 in all other kinds of forceful interactions.
In “regular” collisions involving “regular” sized objects (like people, watermelons, and asteroids, things that are macroscopic), kinetic energy is notconserved. The crash in which kinetic energy of the system is not conserved but the momentum is conserved, then that collision is termed as inelastic collision. 1.2 kg × m/s = 0.20 kg × v2. But if the collision is inelastic, both objects move with one final velocity v in the same direction.
Please refer to the lab manual for the percent loss formula.
• if the objects bounce apart instead of sticking together, the. Inelastic collision is defined as one in which the kinetic energy is converted into another kind of energy such as heat during the impact whereas a fully elastic collision is defined as one in which the kinetic energy loss is zero. Mass of body 2 = m 2 the initial velocity of body 1 = u 1 the initial velocity of body 2 = u 2 M\[_{1}\]u\[_{1}\] + m\[_{2}\]u\[_{2}\] = m\[_{1}\]v\[_{1}\] + m\[_{2}\]v\[_{2}\] 15 x 16 + 10 x 6 = 15 x 0 + 10 x v\[_{2}\] 240 + 60 = 10v\[_{2}\] v\[_{2}\] = \[\frac{300}{10}\] v\[_{2}\] = 30 m/s.
Where mass of body 1 = m 1.
If you're seeing this message, it means we're having trouble loading external resources on our website. Inelastic collision is the type of collision that occurs when both the collided bodies lose kinetic energy and momentum. In these cases the kinetic energy after the collision is most often less than the kinetic energy before. In case of an inelastic collision the momentum is conserved but not the kinetic energy.
Now, we will take the conservation of momentum equation, p1 + p2 = p ′ 1 + p ′ 2 and break it into its x and y components.
= 1/2 (100,000 kg)(3 m/s)2. A special case of this is sometimes called the perfectly inelastic collision. For elastic collisions, e = 1 while for inelastic collisions,e = 0. After the collision, the smaller marble moves to the left at 0.315 m/s.
An inelastic collision is any collision between objects in which some energy is lost.
In terms of masses and velocities, this equation is. • energy is not conserved in a perfectly inelastic collision. In a perfectly elastic collision, the 2 objects attach togethe r after the collision, and; (4 points) for the elastic collision data, please calculate the total momenta of both carts before and after collision, and determine the moment percent loss.
The momentum is also conserved after the collision.
P 1x + p 2x = p ′ 1x + p ′ 2x. To determine whether the collision is elastic or inelastic, calculate the total kinetic energy of the system both before and after the collision. Hence the velocity after elastic collision for second ball is. What is the velocity of the 0.030 kg marble after the collision?
To obtain expressions for the individual velocities after the collision.
{eq}v' = \frac { (800 \text { kg}) (30 \text { m/s}) + (1500 \text { kg}) (0)} {800\text { kg} + 1500 \text {. What is the formula for inelastic collisions? Therefore, the momentum p f after inelastic collision becomes m 1 v + m 2 v or v(m 1 +m 2) Mass of object 1 × initial velocity 1 + mass of object 1 × initial velocity 1 = (mass of 1 + mass of 2) × final velocity of combined objects) in.
Total energy is conserved after the collision.
The momentum of the objects before the collision is conserved, but the total energy is not conserved. May be used along with conservation of momentum equation. In a perfectly inelastic collision, two objects collide and stick together. Inelastic collision formula v= final velocity m1= mass of the first object in kgs m2= mas of the second object in kgs v1= initial velocity of the first object in m/s v2= initial velocity of the second object in m/s what is the formula for perfectly elastic collision?