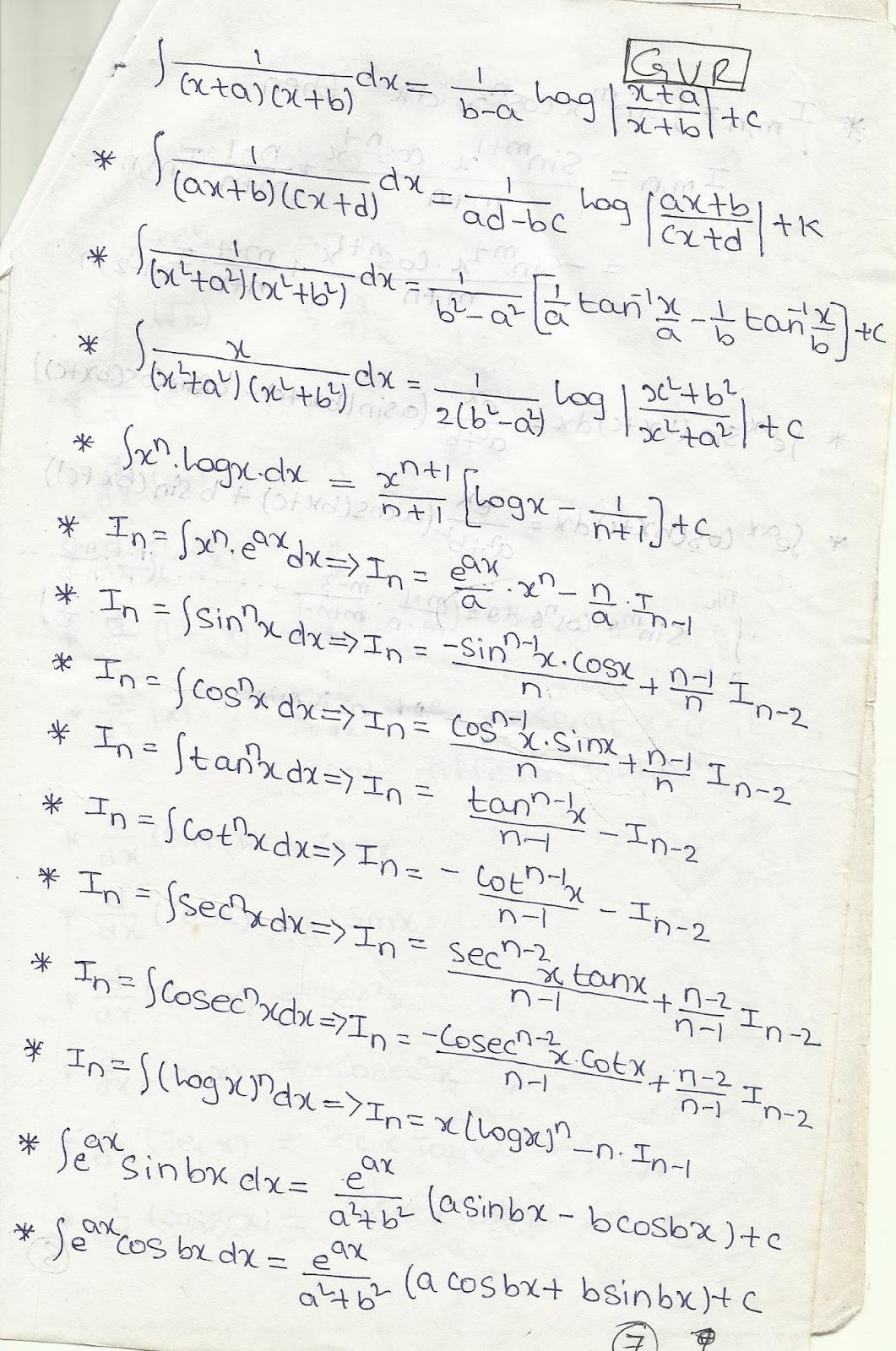Symbols f(x) → integrand f(x)dx → element of integration ∫→ sign of integral φ(x) → anti. F ( x) = ∫ f ( x) d x. Differentiation is an important concept in calculus, on the other hand integration also involves the usage of differentiation formulas and concepts to solve the integration questions.
Integration formulas with examples pdf
Calculus has a wide variety of applications in many fields of science as well as the economy.
Numerical differentiation and interpolation chapter 6 numerical differentiation and integration.
A bx x2 22 a sin and cos 1 sin2 2 b − ⇒= θ θθ −= 22 2 sec and tan sec 12 2 a bx a x b − ⇒=θ θθ = − a bx x2 22 a tan and sec 1 tan2 2 b + ⇒= θ θθ += partial fractions if integrating ( ) ( ) px dx qx ⌠ ⌡ ∫ sec 2 x dx = tan x + c: ∫ (1/x) dx = ln x + c: For the following, let u and v be functions of x, let n be an integer, and let a, c, and c be constants.
Integration formulas for class 12 pdf download:
If the integral contains the following root use the given substitution and formula. Integration formulas pdf file download is very important to crack ssc competitive exams. Differentiation formulas pdf class 12: I = z b a f(x)dx.
To find the particular solution that also satisfies y(2) = 12, as desired, we simply replace the y(2) in the general solution with its given value, y(x) = x3− 8 + y(2) = x3− 8 + 12 = x3+ 4.
D/dx sin x = cos x: Integration as inverse operation of differentiation. 2 1 sin ( ) 1 cos(2 )x 2 sin tan cos x x x 1 sec cos x x cos( ) cos( ) x x 22sin ( ) cos ( ) 1xx 2 1 cos ( ) 1 cos(2 )x 2 cos cot sin x x x 1 csc sin x x sin( ) sin( ) x x 22tan ( ) 1 sec ( )x x geometry fomulas: The formulas include basic integration formulas, integration of trigonometric ratios, inverse trigonometric functions, the product of functions, and some advanced set of integration formulas.
Therefore, the definite integral of f over that interval is shown by:
28 integration and differential equations. Differentiation is used to find the derivative of a defined function, it is. Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= + That is, yex if and only if xy ln.
Integration is the inverse operation of differentiation.
F x e x3 ln , 1,0 example: In this article, we will have some differentiation and integration formula ∫ x n dx = (x n+1 /n+1) + c: Properties of the natural exponential function:
D/dx ln x = 1/x:
These are some of the most frequently encountered rules for differentiation and integration. 0 0 sin sin 1 cos lim 1 lim 0 lim 0 x x x x x x −> −>∞ −>x x x − = = = Z b a fn(x)dx where fn(x) = a0 +a1x+a2x2 +:::+anxn. Differentiation is an important topic of class 12th mathematics.
Integration and differentiation are two very important concepts in calculus.
9781133105060_app_g.qxp 12/27/11 1:47 pm page g1 appendix g.1 differentiation and integration formulas g1 g formulas g.1 differentiation and integration formulas use differentiation and integration tables to supplement differentiation and integration techniques. ∫ cos x dx = sin x + c: Integration and differentiation are some of the m ost frequently needed methods in computational physics. D/dx (a) = 0 where a is constant:
So to integrate a function f(x), you write ∫ f (x)dx
This article on integration formulas pdf download for iit jee exam is prepared for ssc examinations. D/dx e x = e x Also, we may find calculus in finance as well as in stock market analysis. Thus the basic integration formula is ∫ f'(x).dx = f(x) +.
∫ a dx = ax + c:
A s2 1 area of a triangle: Fundamental rules ( ) 𝑥 =0 ∫ 𝑥=𝑥+𝐶 (i) the process of differentiation and integration are inverses of each other in the sense of the following results : This is a general solution to our differential equation.
Best important formula of indefinite integration pdf notes designed according to ssc latest.
Integration formulas y d a b x c= + −sin ( ) a is amplitude b is the affect on the period (stretch or shrink) c is vertical shift (left/right) and d is horizontal shift (up/down) limits: D f x dx dx∫ = f(x) and ∫f x dx′() = f(x) + c,. Integration formulas z dx = x+c (1) z xn dx = xn+1 n+1 +c (2) z dx x = ln|x|+c (3) z ex dx = ex +c (4) z ax dx = 1 lna ax +c (5) z lnxdx = xlnx−x+c (6) z sinxdx = −cosx+c (7) z cosxdx = sinx+c (8) z tanxdx = −ln|cosx|+c (9) z cotxdx = ln|sinx|+c (10) z secxdx = ln|secx+tanx|+c (11) z cscxdx = −ln |x+cot +c (12) z sec2 xdx = tanx+c (13) z csc2 xdx = −cotx+c (14) z Definite integrals relate differentiation with the definite integral:
Check the formula sheet of integration.
D/dx tan x = sec 2 x: All integration and differentiation formulas pdf. Numerical integration and differentiation part i: Because the derivative of a constant function is always zero, so the differentiation process eliminates the ‘c’.
If d/dx {φ(x)) = f(x), ∫f(x)dx = φ(x) + c, where c is called the constant of integration or arbitrary constant.
Integration formulas the following list provides some of the rules for finding integrals and a few of the common antiderivatives of functions. Differentiation (cu) ' — cu' (c constm1t) integration u'vdx(byparts) uv —cos x + c sin x + c in eos + c in + c in + + c in — cot xl + c — amtan — arcsin — arcs inh — arccosh — + c — åsin2r+c tan x — x + c —cot x— x + c du sin x tan sec dr esc dr sin2 x dr dr tan2 x dr cot2 x dr du dy (chain rule) ae —sin cosh x sinh x This is called indefinite integral and is written as: ∫ 1 dx = x+c:
Numerical integration and differentiation part i:
Basically, integration is a way of uniting the part to find a whole.