The integrated rate expression is used to calculate the rate constant of the reaction. Rate = − d a dt d [ a] dt = k [a]. Define half life of a reaction.
PPT Part III Integrated Rate Laws PowerPoint
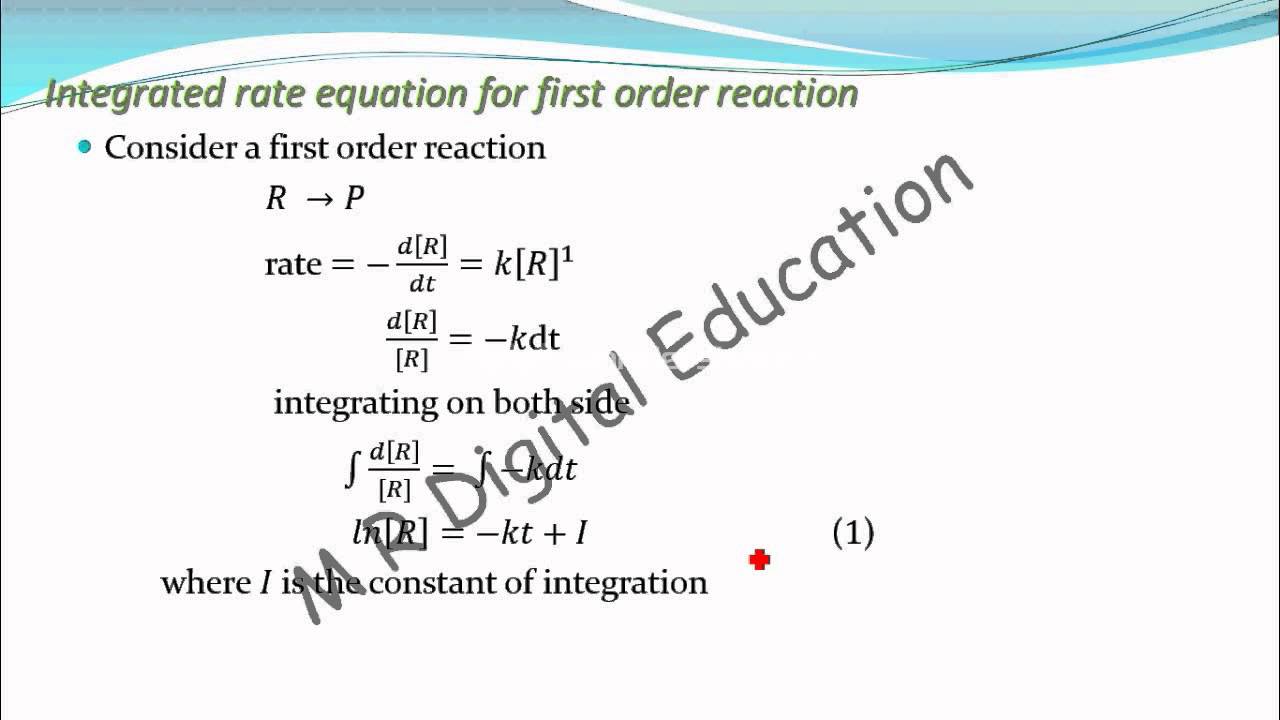
Derive the integrated rate law for first order reaction a or derive the rate equation for first order reaction.
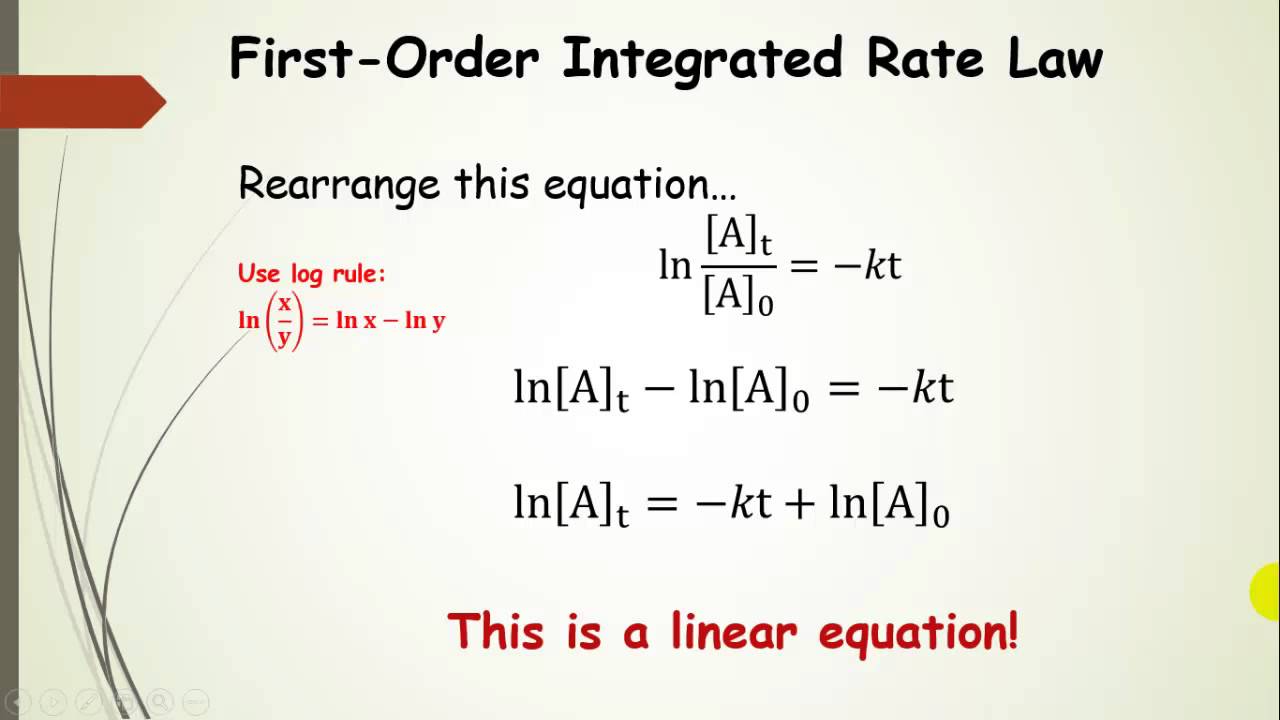
This equation (2) is in natural logarithm.
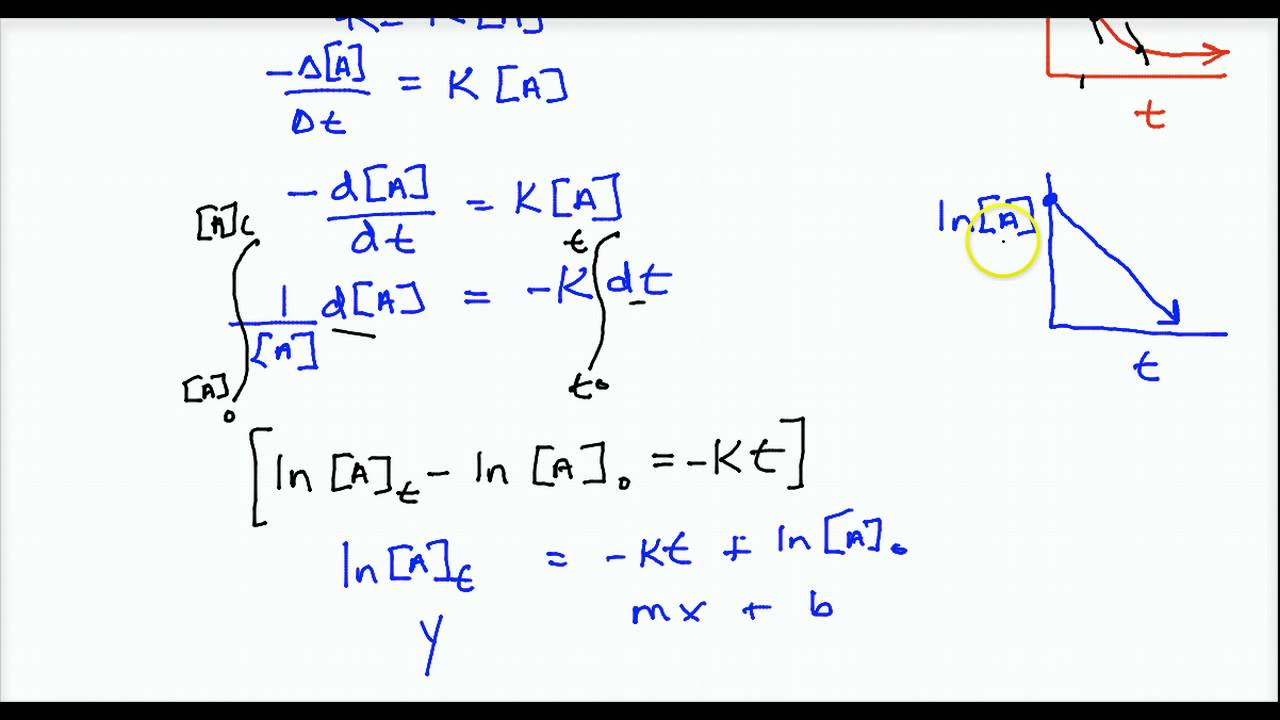
Thus, we can determine the concentration and rate of reaction at any moment with the help of integrated rate equation for zero and first order reaction. Derive integrated rate equation for first order reaction from tessshebaylo.com. The differential rate equation for the first order reaction is − d t d [a] = k [a] rearrange and integrate between the limits [a] = [a] 0 at t = 0 and [a] = [a] t at t = t reaction is ∫ [a] 0 [a] t [a] d [a] = − k ∫ 0 t d t [l n [a]] [a] 0 [a] t = − k (t) 0 t l n [a] t − l n [a] 0 = − k t The concentration is now expressed in terms of.
The rate of reaction is directly proportional to the power of the concentration of reactant.
They are used to determine the rate constant and the reaction order from experimental data. If this is a first order reaction then n = 1: (2) let [a] 0 be the initial concentration of the reactant a at time t = 0. The integrated rate law turns out to be.
Derive the relationship between half life and rate constant for a fi.
T is the time elapsed since the reaction began because hi is the only reactant and the only species that appears in the rate law, the reaction is also second order overall. Izvoru47 and 7 more users found this answer helpful. The differential rate law can be integrated with time to describe the change in concentration of reactants with respect to time. Derive the integrated rate law for the first order reaction, a (g) → b (g) + c (g) in terms of pressure.
R=−dadt=k [a] r = − da dt = k [ a ].
K = t log a a t 2.303 t log 10 [ a] 0 [ a] t. (1) where, [a] is the concentration of reactant at time t. Rate law can be expressed as, rate = k [a]1. When integrated from time 0 to time t and initial concentration [a] 0 to [a] t which is the concentration at time t, the integrated form is this:
On the other hand, integrated rate laws express the reaction rate as a function of the initial concentration and a measured (actual) concentration of one or more reactants after a specific amount of time (t) has passed;
Ln[ ]=− g p+ln[ ]0 Let us derive the integrated rate equation for a 1st order reaction with a rate constant, k. Where, k is the first order rate constant. Using the integrated rate law expressions, we can find the concentration of a reaction or product present after sometime in the reaction.
1 → 2 → (85) where k1 and k2 are the rate constants for the first and second steps, respectively.
The reaction is given below. A) integrated rate equation for the first order reaction: Complete step by step answer: Integrate the above equation (i) between the limits of time t = 0 and time equal to t, while the concentration varies from initial concentration [a0] to [a] at the later time.
In this section, we will look at the integration of 1st, 2nd and 0th order reactions and some interesting graphs that.
The equation (2) is integrated between limits [a] = [a] 0. In first order reaction, in rate law the sum of the powers of concentration of reactant is equal to 1. The pressure of a at time t is obtained by substitution of eq. First order reaction is a → product.