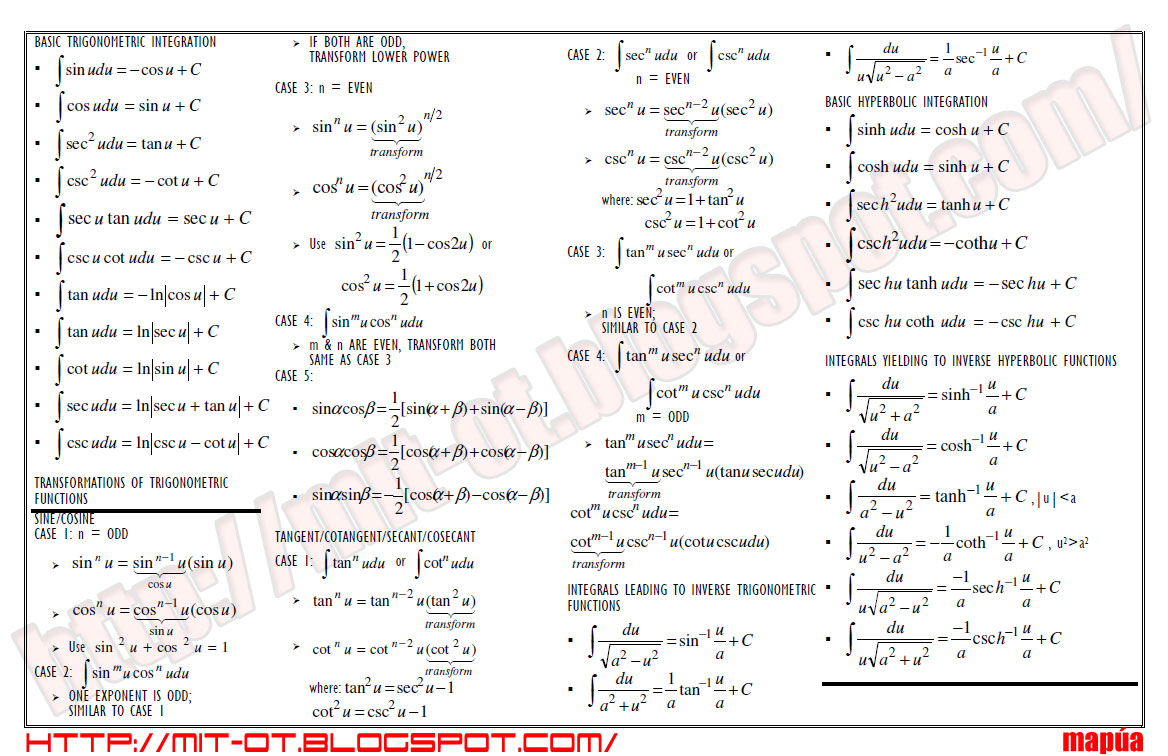
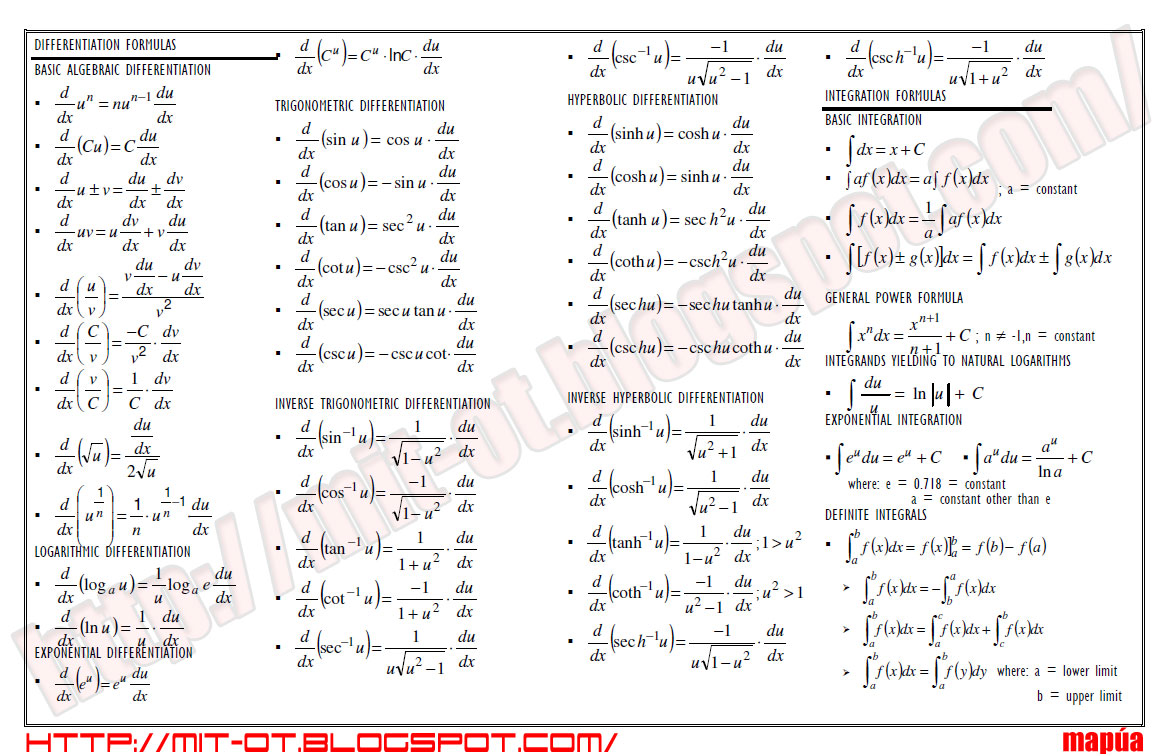
Z xn dx = xn+1 n+1 if n 6= −1 d dx (xn) = nxn−1 z sinxdx = −cosx+c d dx (cosx) = −sinx z cosxdx = sinx+c d dx (sinx) = cosx z sec2 xdx = tanx+c d dx. Derivatives basic properties/formulas/rules d(cf()x)cfx() dx =¢, c is any constant. Formula for the derivative of a specific function corresponds to a formula for the derivative of an elementary function.
Calculus Derivatives Limits
Differentiation is an important concept in calculus, on the other hand integration also involves the usage of differentiation formulas and concepts to solve the integration questions.
Calculus trigonometric derivatives and integrals trigonometric derivatives d dx (sin( x)) = cos( )·0 d dx (cos( )) = sin(0 d dx (tan( x)) = sec2( )· 0 d dx (csc( x)) = csc( )cot( )·0 d dx (sec( )) = sec( )tan(0 d dx (cot(x)) = csc2( x)· 0 d dx (sin 01 (x)) =p 1 1x2 ·xd dx (cos (tan1(x)) = p 1 1x2 0 d dx 1 1 1+x2 ·x 0 d dx (csc 1(x)) = 1 x p x21 ·x0 d dx (sec (cot1 (x)) =1 x p x21 ·x0 d.
()0 d c dx =, c is any constant. Differentiation and integration rules a derivative computes the instantaneous rate of change of a function at different values. Formulas 1 lim 1 n x e →∞ n + = ( ) 1 lim 1 n x n e →∞ + = 0 sin lim 1 x x → x = 0 tan lim 1 x x → x = 0 cos 1 lim 0 x x → x − = lim 1 n n n x a x a na x a − → − = − 0 1 lim ln n x a a → x − = 2. Differentiation is an important topic of class 12th mathematics.
Vs 1/s2 vs n 1/s3/2 vs a 2, n—l 1)!
Derivatives definition and notation if yfx then the derivative is defined to be 0 lim h fx h fx fx h. Integral calculus formula sheet derivative rules: (1) f 0(x) = lim h!0 f(x+h) f(x) h (2) d dx (c) = 0; C any constant (3) d dx (x) = 1 (4) d dx (xp) = pxp 1;
Differentiation formulas pdf class 12:
Because the derivative of a constant function is always zero, so the differentiation process eliminates the ‘c’. So to integrate a function f(x), you write ∫ f (x)dx 0 0 sin sin 1 cos lim 1 lim 0 lim 0 x x x x x x −> −>∞ −>x x x − = = = If yfx then all of the following are equivalent notations for the derivative.
A definite integral is used to compute the area under the curve these are some of the most frequently encountered rules for.
Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= + '( ) 3 ( ) '( ) 3 ( ) '( ) 3 ( ) 2 2 2 h x x f x g x x f x f x x f x = = = = = = although integration has been introduced as an antiderivative, the symbol for integration is ‘∫’. Integration formulas y d a b x c= + −sin ( ) a is amplitude b is the affect on the period (stretch or shrink) c is vertical shift (left/right) and d is horizontal shift (up/down) limits: Derivative rules and formulas rules:
The following table lists integration formulas side by side with the corresponding differentiation formulas.
Fx y fx dfx df dy d dx dx dx if yfx all of the following are equivalent notations for derivative evaluated at x a. Integration formulas z dx = x+c (1) z xn dx = xn+1 n+1 +c (2) z dx x = ln|x|+c (3) z ex dx = ex +c (4) z ax dx = 1 lna ax +c (5) z lnxdx = xlnx−x+c (6) z sinxdx = −cosx+c (7) z cosxdx = sinx+c (8) z tanxdx = −ln|cosx|+c (9) z cotxdx = ln|sinx|+c (10) z secxdx = ln|secx+tanx|+c (11) z cscxdx = −ln |x+cot +c (12) z sec2 xdx = tanx+c (13) z csc2 xdx = −cotx+c (14) z An indefinite integral computes the family of functions that are the antiderivative. © 2005 paul dawkins integrals basic properties/formulas/rules òòcf(x)dx= cf(x)dx, c is a constant.
P 6= 1 (5) d dx [f(x) g(x)] = f0(x) g0(x) (6) d dx (cf(x)) = cf0(x) (7) d dx [ f x)g)] = )+ (8) d dx f(x) g(x) = f0(x)g(x) f(x)g0(x) (g(x))2 (9) d dx 1 g(x) = g0(x) (g(x))2 (10) d dx [ f (g x))] = 0)) 0) (11) d dx f 1(x) = f 1 0 (x) = 1 f0(f 1(x))
0 d c dx nn 1 d xnx dx sin cos d x x dx sec sec tan d x xx dx tan sec2 d x x dx cos sin d x x dx csc csc cot d x xx dx cot csc2 d x x dx d aaaxxln dx d eex x dx dd cf x c f x dx dx