\ [ ∫ a b \] f ( x) d x. Interpret the constant of integration graphically. These properties are used in this section to help understand functions that are defined by integrals.
Math Pdf Math Class 10 Trigonometry Formula / Maths
All these integrals differ by a constant.
Of a definite integral as a riemann sum, but they also have natural interpretations as properties of areas of regions.
Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2; 3 + p 2;cos2 ax (69) z cos3 axdx= 3sinax 4a + sin3ax 12a (70) z cosaxsinbxdx=. Properties of the definite integral 5.33 15 27x 18x 27x 18x 243 90 + units f(x) 5.33 15.75 (0,.75 above area:
7.1.3 geometrically, the statement∫f dx()x = f (x) + c = y (say) represents a family of.
7.1 indefinite integrals calculus learning objectives a student will be able to: (this just means we nd the antiderivative using ibp and then plug in the limits of integration the way we do with other de nite integrals.) trigonometric integrals for integrals involving only powers of sine and cosine (both with the same argument): Change of variables formula in words, we can change the variable from u to x, provided we 3 2;cos2 ax (65) z sin3 axdx= 3cosax 4a + cos3ax 12a (66) z cosaxdx= 1 a sinax (67) z cos2 axdx= x 2 + sin2ax 4a (68) z cosp axdx= 1 a(1 + p) cos1+p ax 2f 1 1 + p 2;
∫ 1 dx = x + c.
They will be used in future sections to help calculate the values of definite integrals. ∫ sec x (tan x) dx = sec x + c. Again, we note that for any real number c, treated as constant function, its derivative is zero and hence, we can write (1), (2) and (3) as follows : ∫ cos x dx = sin x + c.
Integration formulas z dx = x+c (1) z xn dx = xn+1 n+1 +c (2) z dx x = ln|x|+c (3) z ex dx = ex +c (4) z ax dx = 1 lna ax +c (5) z lnxdx = xlnx−x+c (6) z sinxdx = −cosx+c (7) z cosxdx = sinx+c (8) z tanxdx = −ln|cosx|+c (9) z cotxdx = ln|sinx|+c (10) z secxdx = ln|secx+tanx|+c (11) z cscxdx = −ln |x+cot +c (12) z sec2 xdx = tanx+c (13) z csc2 xdx = −cotx+c (14) z
7.1 overview 7.1.1 let d dx f (x) = f (x). ∫ x n dx = ( (x n+1 )/ (n+1))+c ; (sin +c) cos= d xx dx, 3 ( +c) 2 3 = dx x dx and ( +c)x = x d ee dx thus, anti derivatives (or integrals) of the above cited functions are not unique. Integral calculus formula sheet derivative rules:
0 d c dx nn 1 d xnx dx sin cos d x x dx sec sec tan d x xx dx tan sec2 d x x dx cos sin d x x dx csc csc cot d x xx dx cot csc2 d x x dx d aaaxxln dx d eex x dx dd cf x c f x dx dx
∫a→b f (x) dx = ∫a→b f (t) dt. The list of basic integral formulas are. 16 x2 49 x2 dx ∫ − 22 x = ⇒ =33sinθ dx dcosθθ 49− x2=−= =4 4sin 4cos 2cos22θ θθ recall xx2=. Formula to convert into an integral involving trig functions.
Because we have an indefinite
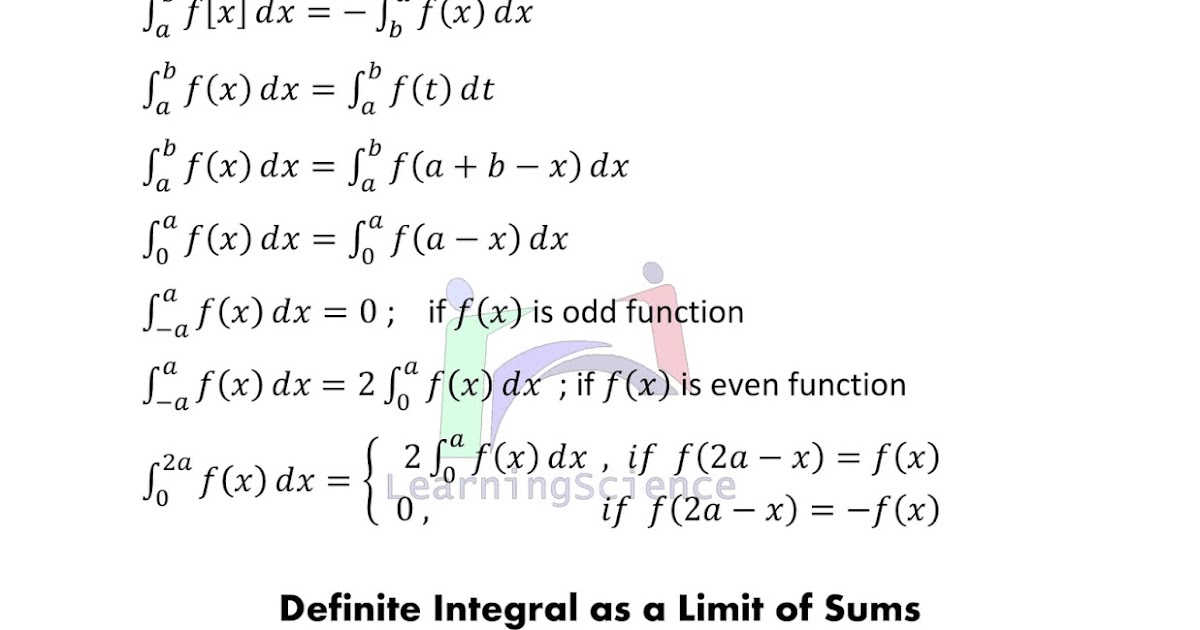
The formula is the most important reason for including dx in the notation for the definite integral, that is, writing z b a f(x)dx for the integral, rather than simply z b a f(x), as some authors do. • apply definite integrals to find the area of a bounded region. These integrals are called indefinite integrals or general integrals, c is called a constant of integration. Up to 24% cash back definite integrals (vi) ( ) ( ) 2aa 00 ∫∫fxdx= 2fxdx if f( 2a−=x) fx( ) i=0 f( 2a−x) =−fx( ) (vii) ( ) ( ) aa a0 fxdx2fxdx − ∫∫= if f is an even function of x = 0 if f is an odd function of x.
2 22 a sin b a bx x− ⇒= θ cos 1 sin22θθ= − 22 2 a sec b bx a x− ⇒= θ tan sec 122θθ= − 2 22 a tan b a bx x+ ⇒= θ sec 1 tan2 2θθ= + ex.
(7) z d c f(u)du = z b a f(u(x)) du dx dx, u = u(x), c = u(a), d = u(b). Then, we write∫f dx()x = f (x) + c. Table of integrals basic forms (1)!xndx= 1 n+1 xn+1 (2) 1 x!dx=lnx (3)!udv=uv!vdu (4) u(x)v!(x)dx=u(x)v(x)#v(x)u!(x)dx rational functions (5) 1 ax+b!dx= 1 a ln(ax+b) (6) 1 (x+a)2!dx= 1 x+a (7)!(x+a)ndx=(x+a)n a 1+n + x 1+n #$ % &', n!1 (8)!x(x+a)ndx= (x+a)1+n(nx+xa) (n+2)(n+1) (9) dx!1+x2 =tan1x (10) dx!a2+x2 = 1 a tan1(x/a) (11) xdx!a2+x2. Integrals with trigonometric functions z sinaxdx = 1 a cosax (63) z sin2 axdx = x 2 sin2ax 4a (64) z sinn axdx = 1 a cosax 2f 1 1 2, 1 n 2, 3 2,cos2 ax (65) z sin3 axdx = 3cosax 4a + cos3ax 12a (66) z cosaxdx = 1 a sinax (67) z cos2 axdx = x 2 + sin2ax 4a (68) z cosp axdx = 1 a(1 + p) cos1+p ax⇥ 2f 1 1+p 2, 1 2, 3+p 2,cos 2ax (69) z cos3 axdx = 3sinax 4a + sin3ax 12a (70) z.
Next, construct the definite integral.
∫ a dx = ax+ c. Cos(x)dx = 2sin(x) π/2 0. With de nite integrals, the formula becomes z b a udv= u(x)v(x)]b a z b a vdu: ∫a→b f (x) dx = ∫a→c f (x) dx + ∫c→b f (x) dx.
Ex are the anti derivatives (or integrals) of x2 and ex, respectively.
Expected background knowledge • knowledge of integration • area of a bounded region ∫ sec 2 x dx = tan x + c.