B → c be two functions such that the range of g equals the domain of f. G, is another function defined as The resulting function is known as a composite function.
Functions Composite functions (L6) Core 3 Edexcel A
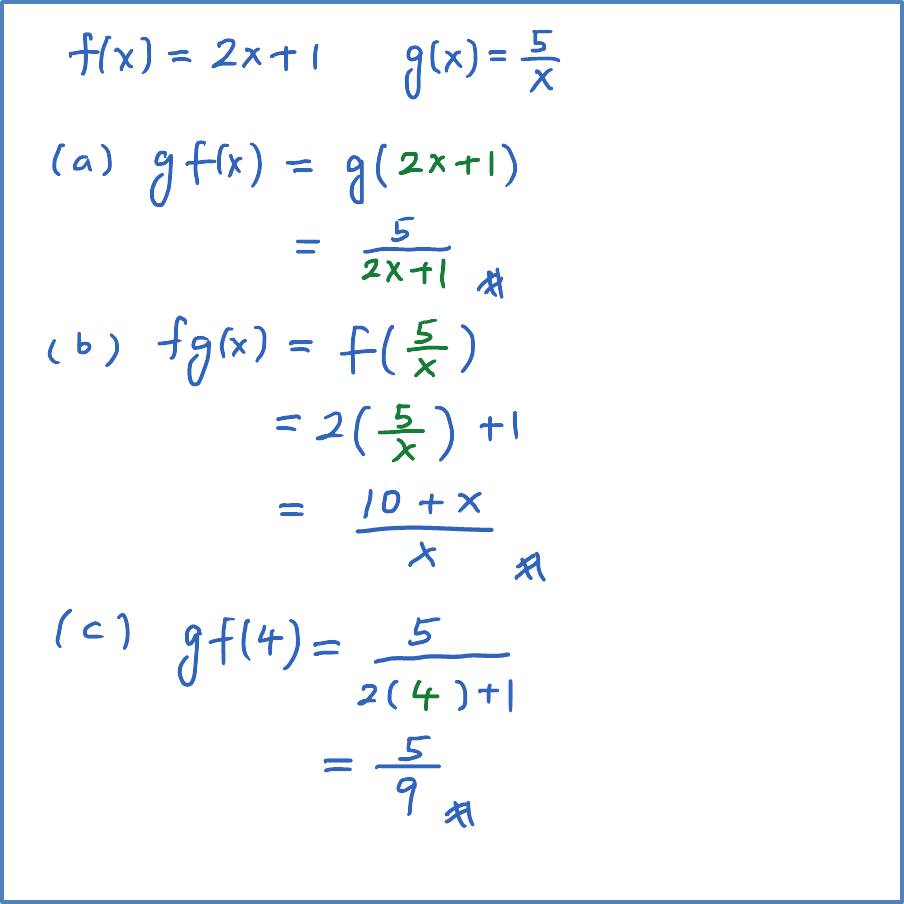
For the functions f (x) and g (x), when g (x) is used as the input of f (x), the composite function is written as:
A function made of other functions, where the output of one is the input to the other.
The composition of the functions f and g, denoted by f ∘ If we have a function f and another function g , the function fg(x) , said as “ f of g of x ”, or “ fg of x ”, is the composition of the two functions. A function that is the composite of several functions. Composing functions that are sets of point, composing functions at points, composing functions with other functions, word problems using composition, inverse functions and composition.
The order of how the functions are applied is important.
The functions 2x+3 and x 2 together make the composite function (2x+3) 2 A composite function is a function created when one function is used as the input value for another function. A → b and f: The notation is (fdg)(x) or f(g(x)), read f of g of x, where f and g are both functions of x.
Similarly to relations, we can compose two or more functions to create a new function.
This operation is called the composition of functions. It explains how to evaluate composite functions. A composite function is one in which the output of one function becomes the input for another. In mathematics, the composite function is an action in which two functions, f, and g, are combined to produce a new function, h, with the formula h(x) = g(f(x)).
You can also evaluate compositions symbolically.
Composition of functions:composing functions with functions (page 3 of 6) sections: Suppose there are two functions, and , then if function becomes input for function , it is called “composition of function with function or simply a composite function. This algebra video tutorial provides a basic introduction into composite functions. (g º f)(x) = g(f(x)), first apply f(), then apply g() we must also respect the domain of the first function;
We represent this combination by the following notation:
Function composition is applying one function to the results of another. If the range $y_i$ of a function $f_i$ is contained in the domain $x_ {i+1}$ of a function $f_ {i+1}$, that is, if. Essentially, the output of the inner function (the function used as the input value) becomes the input of the outer function (the resulting value). The composite functions or the idea of composition of functions is simple.
We represent this combination by the following notation:
In f(g(x)), the g(x) function is substituted for x in the f(x) function. Composite functions are when the output of one function is used as the input of another. X → y is defined as invertible if a function g: Y → x exists such that gof = i_x and fog = i_y.
A composite function is a function obtained when two functions are combined so that the output of one function becomes the input to another function.