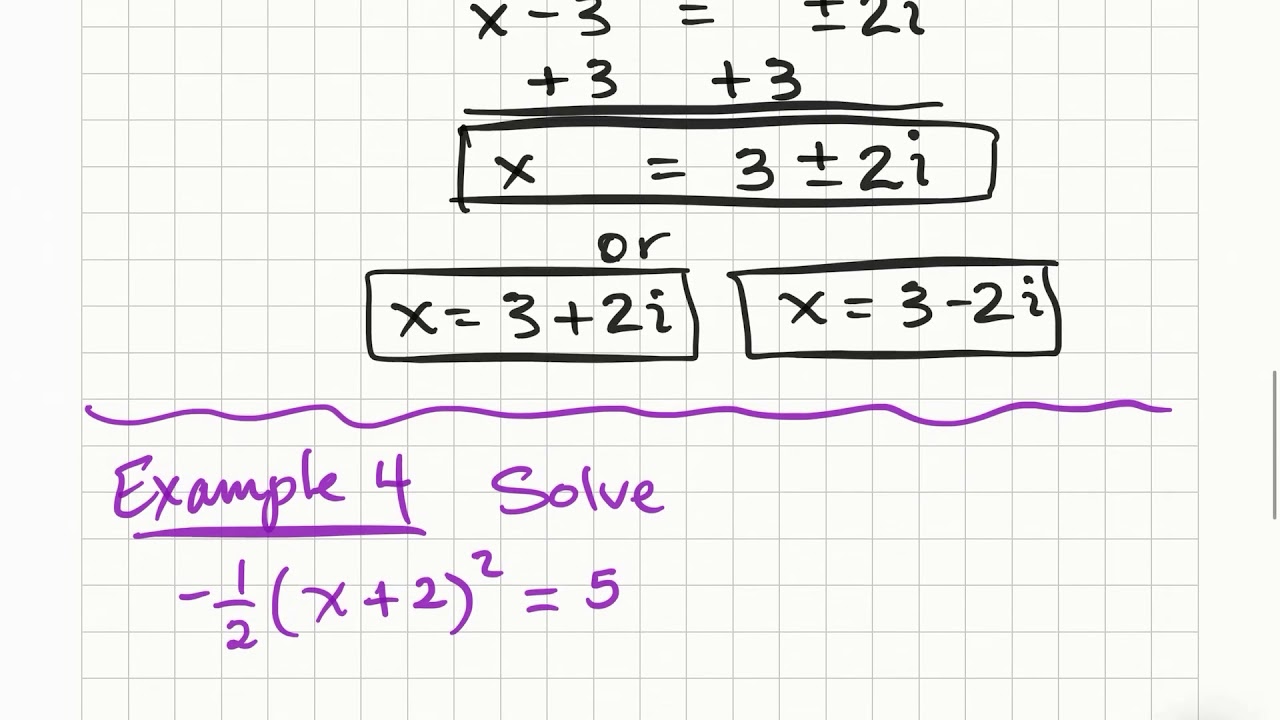1 2 x 1 2 rewrite the inequality. For example, we could evaluate t (5) t (5). Therefore, the domain of [ f g](x) is 1 2 x 1 2, x 0.
Algebra 1 10.4 Solving with Square Roots YouTube
F(x) =( x−1 x)3 f ( x) = ( x − 1 x) 3.
F(x) = x/(√ 2 + x(1 − √ 2)) is a functional square root of g(x) = x/(2 − x).
The composition of a function and itself is called iteration. To avoid confusion let's just call it input: A → b and g : Since square roots are positive, 3.
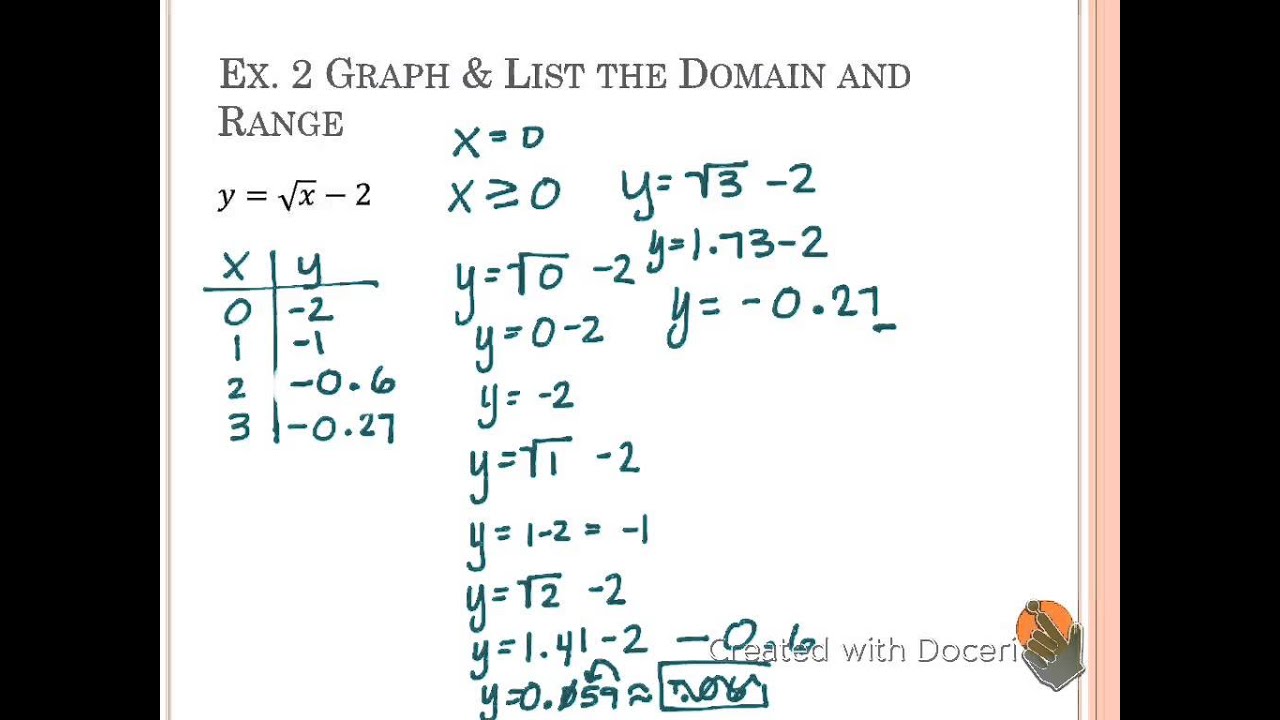
So, the domain is a set of all values that used in a function and a certain function must work for all given values.
As one possibility, we might notice that the expression. X is just a placeholder. Linear, polynomial, square (quadratic), absolute value, square root, rational, sine or cosine. Then the composition of f and g, denoted by g ∘ f, is defined as the function g ∘ f :
A of w is equal to 0.2 times w squared, gives the area, a, in square meters under flox's parachute when it has a width of w meters.
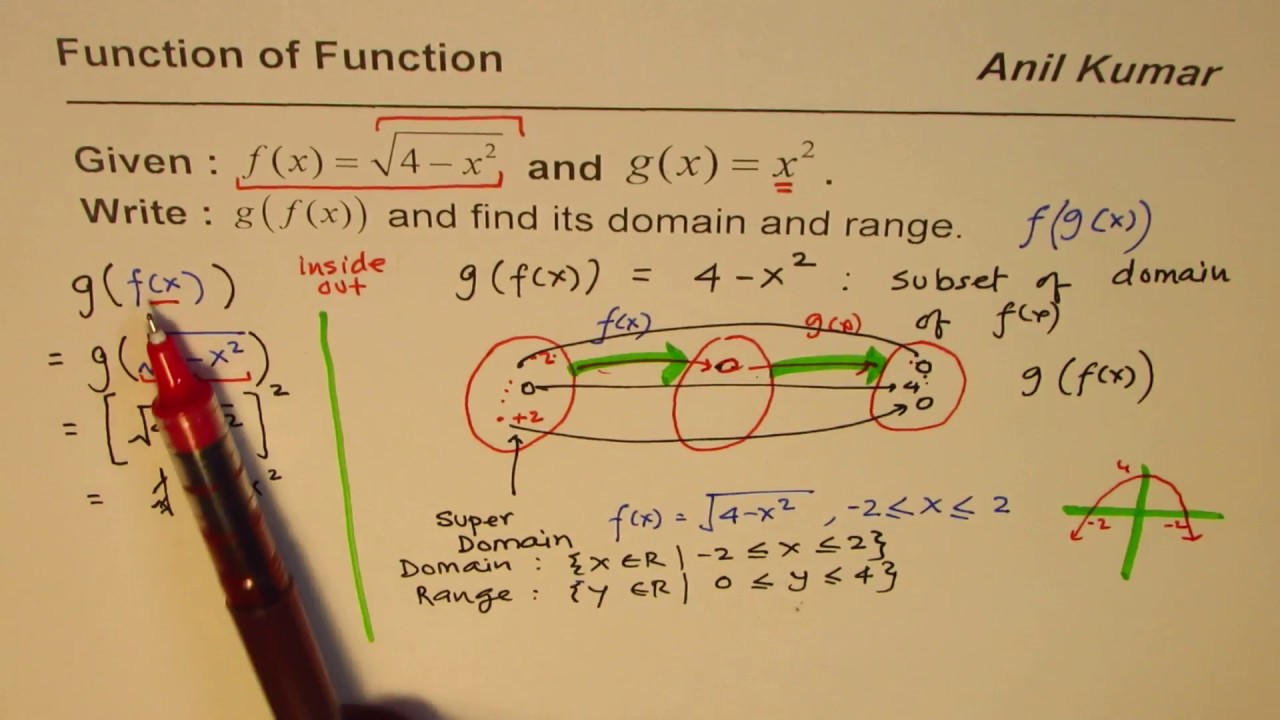
From the above equation, we can deduce that, u(x) = x3 v(x) = x−1 x u ( x) = x 3 v ( x) = x − 1 x. Up to 24% cash back x t ake the square root of each side. Whenever we have functions with square roots, the domain will be the set of numbers for which the expression inside the root isn't negative. H ( x) = 5 − x 2 and g ( x) = x.
Write the functions using standard terminology (f(x) and g(x)).
This algebra 2 and precalculus video tutorial provides an introduction basic overview / review on composite functions that contain fractions and radicals suc. H ( x) = 5 − x 2 and g ( x) = x. F (x) = 2x+3 and g (x) = x2. The above function can be broken down as a composition of two separate functions, f(x) =u(v(x)) =( x−1 x)3 f ( x) = u ( v ( x)) = ( x − 1 x) 3.
F(x) = 2x 2 is a functional square root of g(x) = 8x 4.
Now we need to work this inequality algebraically to get a more useful condition. Find the domain for \(. The below figure shows the representation of composite functions. 5 − x 2 is the inside of the square root.
B → c be two functions.
With that in mind, let's first find the expressions of the composite functions: The original function might be: Also, the composite function calculator determines the function from domain of composite functions at certain point: First, we add 4 to both sides:
(g º f) (x) = g (f (x)) first we apply f, then apply g to that result:
F (input) = 2 (input)+3. To iterate a function f(x), find the function value f(x 0), of the initial value x0. Given a composite function and graphs of its individual functions, evaluate it using the information provided by the graphs. The function v of a is equal to the square root of 980 over a gives flox's maximum speed in meters per second when she skydives with an area of a square meters under her parachute.
Each output of an iterated function is called an iterate.
A functional square root of the n th chebyshev polynomial, g(x) = t n (x), is f(x) = cos(√ n arccos(x)), which in general is not a polynomial. A → c given by g ∘ f (x) = g(f (x)), ∀ x ∈ a. The order of function is an important thing while dealing with the composition of functions since (f ∘ g) (x) is not equal to (g ∘ f) (x). We could then decompose the function as.
(g º f) (x) = (2x+3) 2.