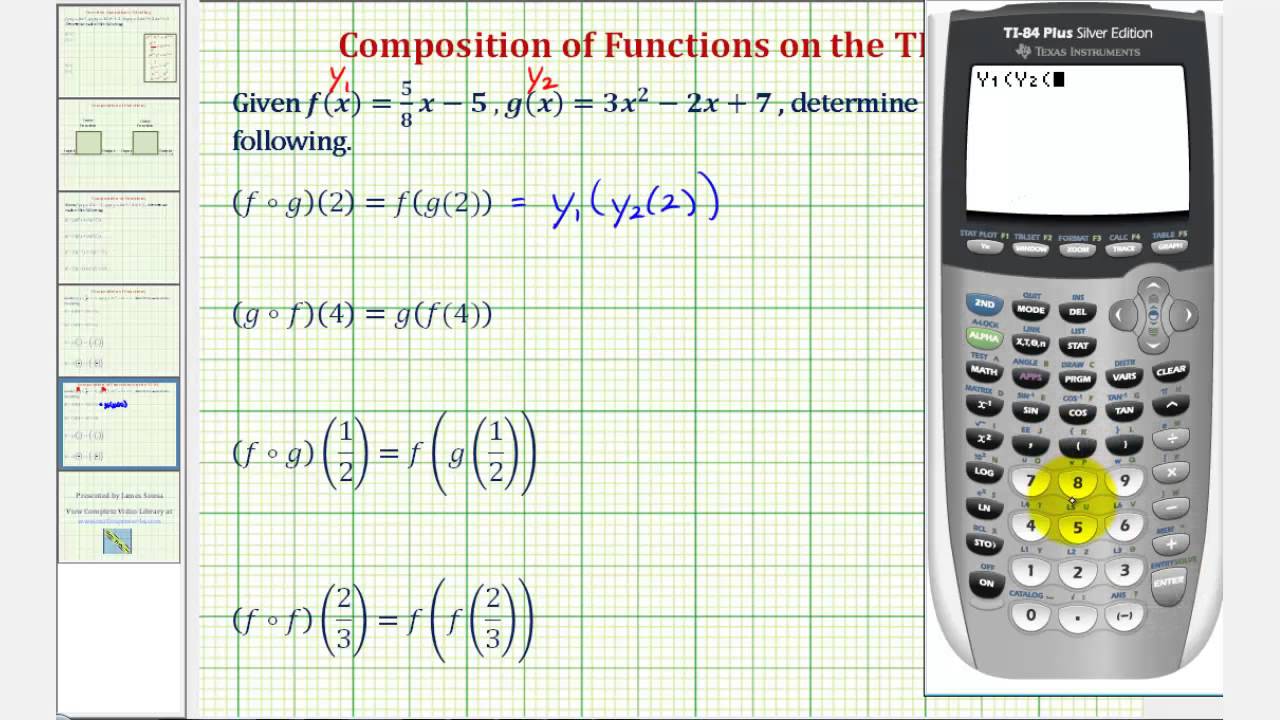
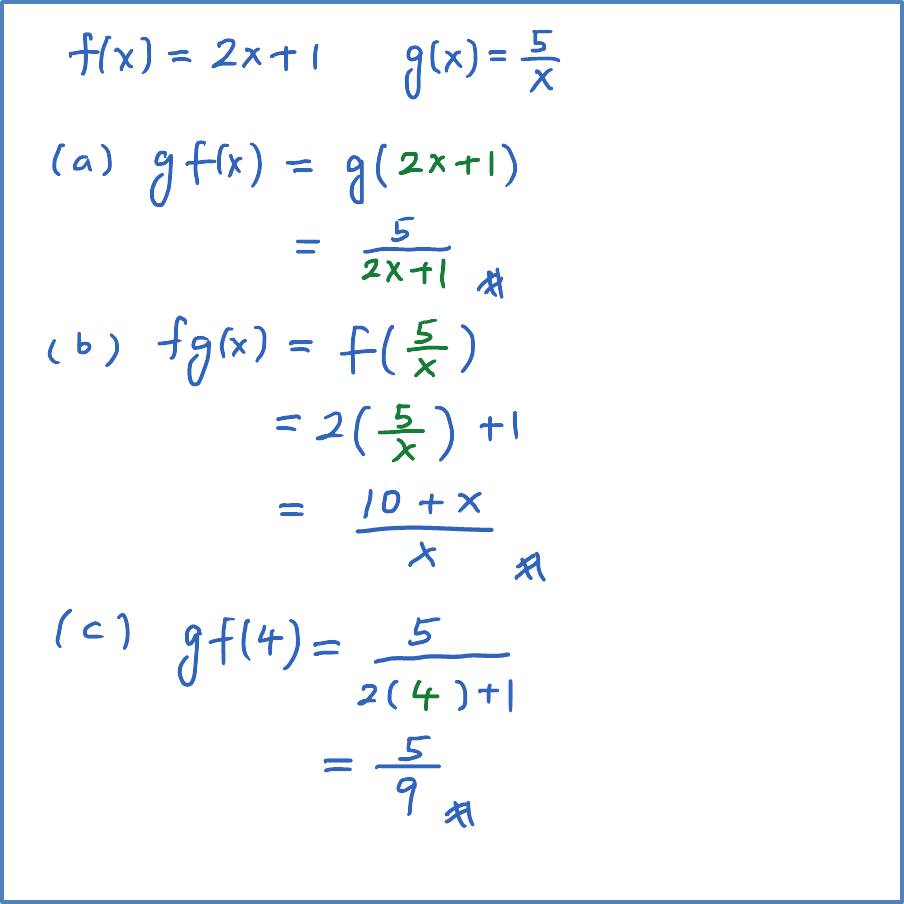
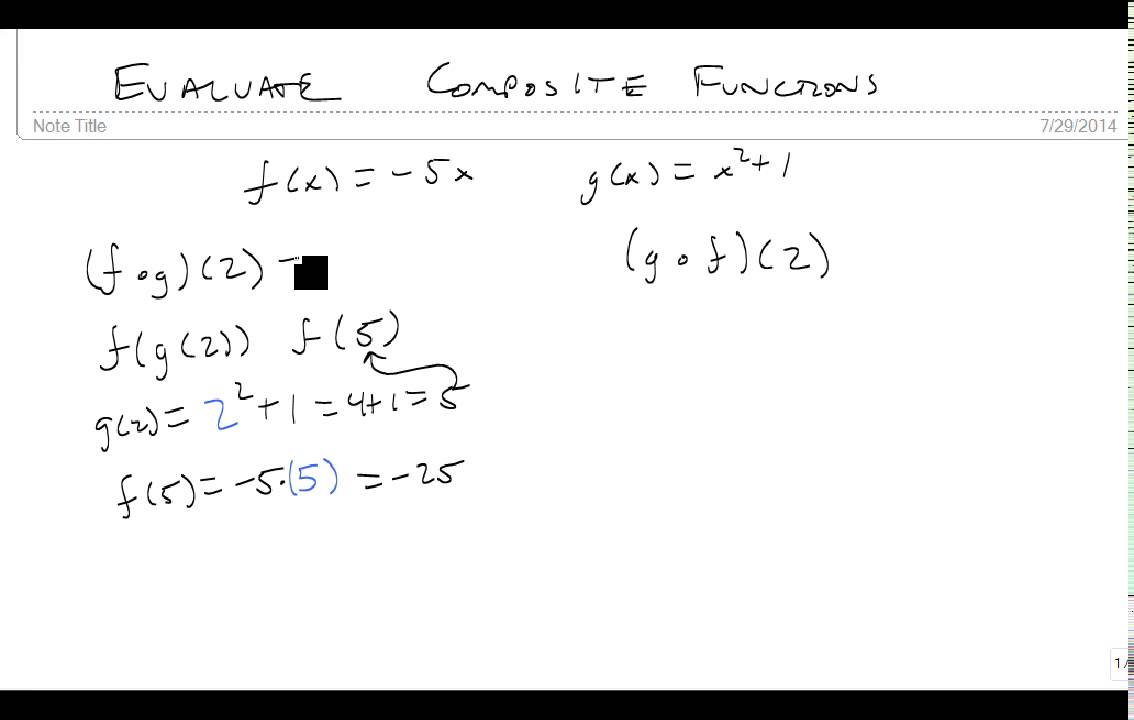The following diagram shows some examples of. A composite function is created when one function is substituted into another function. (f º f) (x) = 2 (2x+3)+3 = 4x + 9.
Composition of functions Word problem worksheets
So, \ (f (2) = 3 (2) + 2 = 8\) and.
G(x) = 2x + 1
F(x) = 4x 2 + 3; F \circ g = f\left [ {g\left ( x \right)} \right] f ∘ g = f [g (x)] , the input or “inner function” is function. You can find the composite of two functions by replacing every x in the outer function with the equation for the inner function (the input). For example, suppose we two fractions, say 3 5 and 5 9 and we want to divide the 3 5 by 5 9, we will have.
G ∘ f = g [ f ( x)]
D(h(x))/dx = df/du × du/dx ⇒ h'(x) = 10u 9 × 3x 2 = 10(x 3 + 7) 9 × 3x 2 = 30 x 2 (x 3 + 7) 9. F(g(x)) can also be written as (f ∘ g)(x) or fg(x), in the composition (f ∘ g)(x), the domain of f becomes g(x). Let us try to solve some questions based on composite functions. 3 5 ÷ 5 9 = 3 5 x 9 5 = 27 25.
The order of composition is important when dealing with composition of functions examples.
F f which is the main or “outer function”. Evaluating composite functions (advanced) next lesson. Home / composite functions with fractions. In mathematics, the composite function is an action in which two functions, f, and g, are combined to produce a new function, h, with the formula h(x) = g(f(x)).
We should be able to do it without the pretty diagram:
The values coming out of a function are. Learn more about composition of functions here. F(g(x)) is read as “f of g of x”. You can use composite functions to check if two functions are inverses of each other because they will follow the rule:
Determine the derivative of the composite function h(x) = (x 3 + 7) 10.
G g because it is to the right of function. So the derivative of h(x) is given by: Derivative of composite function y = sin (cos (x 2)) Given the function f(x) = 3x + 5 and g (x) = 2x3 2 x 3.find ( gof) (x) and ( fog) (x).
We can compose fractions by taking two more fractions and combining them to form a larger fraction.
In terms of the order of composition, do you see the same pattern in. Now, let u = x 3 + 7 = g(x), here h(x) can be written as h(x) = f(g(x)) = u 10. Let’s take an example of composite fractions. Math · precalculus · composite and inverse functions · composing functions.
(f º f) (x) = f (f (x)) = f (2x+3) = 2 (2x+3)+3.
It signifies that the g function is being applied to. Composing fractions using two halves A b ÷ c d = a b x d c = a d b c. It is the value of f(g(x)) of given composite function with fraction.
G was the function performed on x.
Let's look at another composite function example.