The range is all real y ≥−3. We can combine functions using addition, subtraction, multiplication, and division by performing operations with the algebraic expressions that appear on the right side of the equations. For example, f(g(x)) is the composite function that is formed when g(x) is substituted for x in f(x).
Math Plane Composite Functions Topics
A parabola, which has vertex (3,−3), is sketched below.
The graph of y = √ x+4.
A) h(x) = x3, g(x) = 3x Distribute copies the more composition of functions activity sheet. If these two functions are written as h : Have students complete it individually or in.
The composite function rule tells us that f (x)=17(x2 +1)16 ×2x.
Decomposing a composite function write the function given by as a composition of two functions. Inverse functions let f and g be two functions such that f(g(x)) = x for every x in the domain of g, and g(f(x)) = x for every x in the domain of f. The following diagram shows some examples of. Present two graphs, then use them to explain the composition of functions graphically.
5 4 2 ~g~x!!2, we can enterf 8 g asy3 5 4 2 y22andg 8 f as5 ˇy1.observethatforf8gwe follow the defining rule for composition functions:
Take the qth power of both sides to obtain yq = xp: Note that we are assuming that x is chosen in such a way that x p q is de ned example 3.3.3 show that d dx A composite function is created when one function is substituted into another function. Forming composite functions example 1:
Also in example 2, the domain for f(x) = x2 + 2 is all real numbers.
Composite functions the function f(x) = 2x + 3, from example 1, is composed of two simpler functions, i.e. O find f(g(x)) and g(f(x))_____ you try… verify whether the pairs of functions are inverses of each other: Solution the domain of this parabola is all real x. Thus, y0= p q xp 1 x p(q 1) q = p q x p q 1:
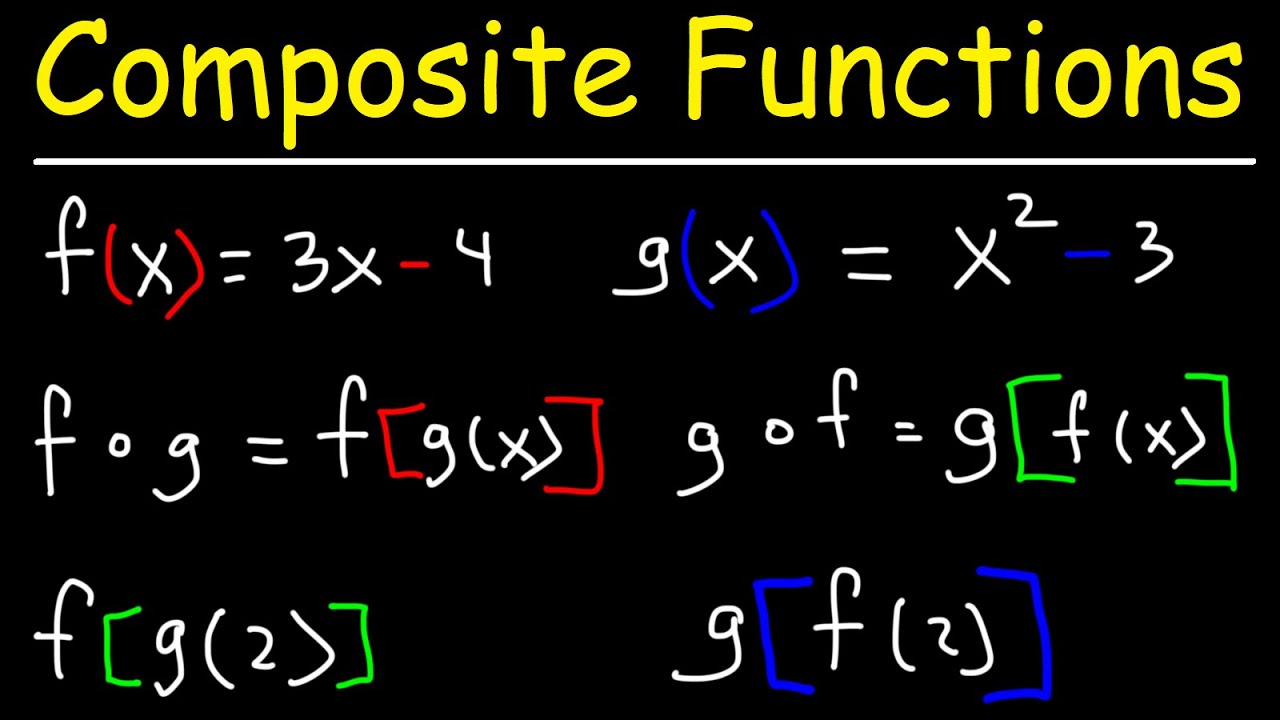
Given f(x) = 5x + 2 and g(x) = 3x − 4, find ( f g)(x) and ( g f)(x).
G(x) = 3 1 x; This can be rewritten as (z3 +4z2 − 3z − 3)−6. Multiply by 2 and add 3. The inside function is z3 +4z2 −3z−3 with derivative 3z2 +8z−3.
Provide several examples and explain how to find the composition of functions in terms of x.
Given two function f and g, the composite function, which we denote by f g and read as \f composed with g, is de ned by (f g)(x) = f(g(x)). So the domain for the composite function is also x ≤ 3. X 7→x + 3 then the composition of these two functions is written gh (sometimes as g h or g(h(x))). For example, the functions and can be combined to form the sum, difference, product, and quotient of and here’s how it’s done:
Answers to composite functions examples (id:
For example, having enteredf and g asy1 5 4 2 x2and. Addition (f + g)(x)= f(x)+ g(x) subtraction (f − g)(x)= f(x) − g(x) multiplication (f · g)(x)= f(x)g(x) division f g (x)= f(x) g(x) when we do one of these four basic operations we can simply evaluate the two functions at the value and then do the operation with both solutions example 1. The outside function is (·)−6 which has derivative −6(·)−7. Know that the square root functions are always positive so the range of y = √ x+4is all real y ≥ 0.
This means, first we evaluate g(1) = 3(1) + 1 = 4;
G (1) = 8, use the definition to get. G x x 2 h h x 1 x 2 2 f x x3 h g x 3x 5 h x3 3 xg 5 31. As another example let us differentiate the function 1/(z3 +4z2 − 3z − 3)6. F x 1 x2 x 2.
Use composition of functions to show that f and g are inverses.
C) if we put together steps (a) and (b) above, we can say that. Di erentiate both sides with respect to x to obtain qyq 1y0= pxp 1: Use the definition of the composite function to write. The domain of f g is the set of
This instructional aid was prepared by the tallahassee community college learning commons.
Suppose that y = x p q; The input function f(x) has no restrictions, so the domain of g(f(x)) is determined only by the composite function. Find the domain and range of this function. The chain rule says that d dz
Relationship between composite functions and inverses example:
F(g(x)) is read as “f of g of x”. Thus f(f −1(x))= x and f(f(x)) = x. Since ƒ(1) = 2 and. In other words, the function f composed with g is the function you get by putting the function g into the function f.
F(x)= x2 − x − 2 g(x)= x +1
H x 1 x 2 2 x 2 2 f x 2 f g x. (fg + ) (1) (fg f g + =+) (1 (1) (1)) (fg f g +=+)(x xx) () = +2 8 =10 Where p and q are integers with q > 0: X 7→2x and g :