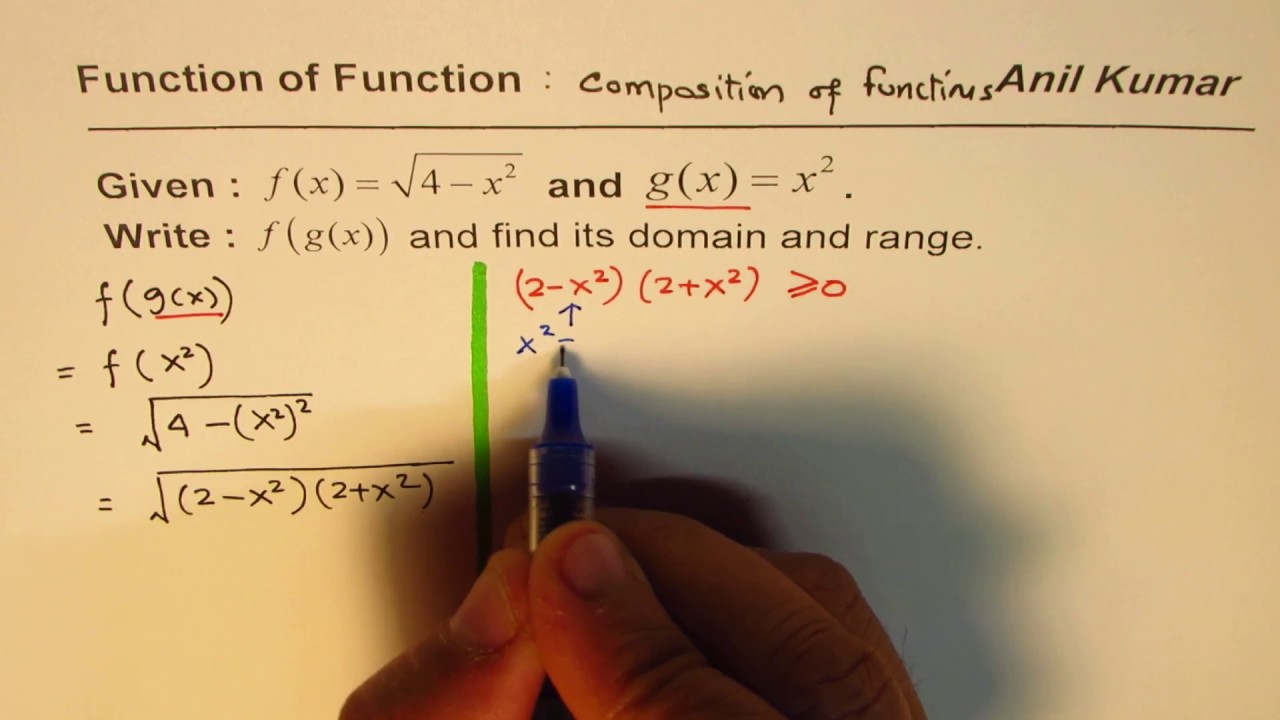
The domain of a composite function [latex]f\left(g\left(x\right)\right)[/latex] is the set of those inputs [latex]x[/latex] in the domain of [latex]g[/latex] for which [latex]g\left(x\right)[/latex] is in the domain of [latex]f[/latex]. The domain of g (x) = x2 is all the real numbers. In this video i will introduce you to finding the domain and range of a composition of two functions.
Finding Domain of Composite Functions YouTube
Just as functions can be combined to form a composite function, composite functions can be decomposed into simpler functions.
To calculate the domain of a composite composition (f∘ g)(x) ( f ∘ g) ( x):
G(x+1) = (x+1)2 g ( x + 1) = ( x + 1) 2. Now, x normally has the domain of all real numbers. Find the domain of the outside function f(x) f ( x). Domain of a composite function.
The domain of will be the values from the domain of g(x) which can “move through” to the end of the composition.
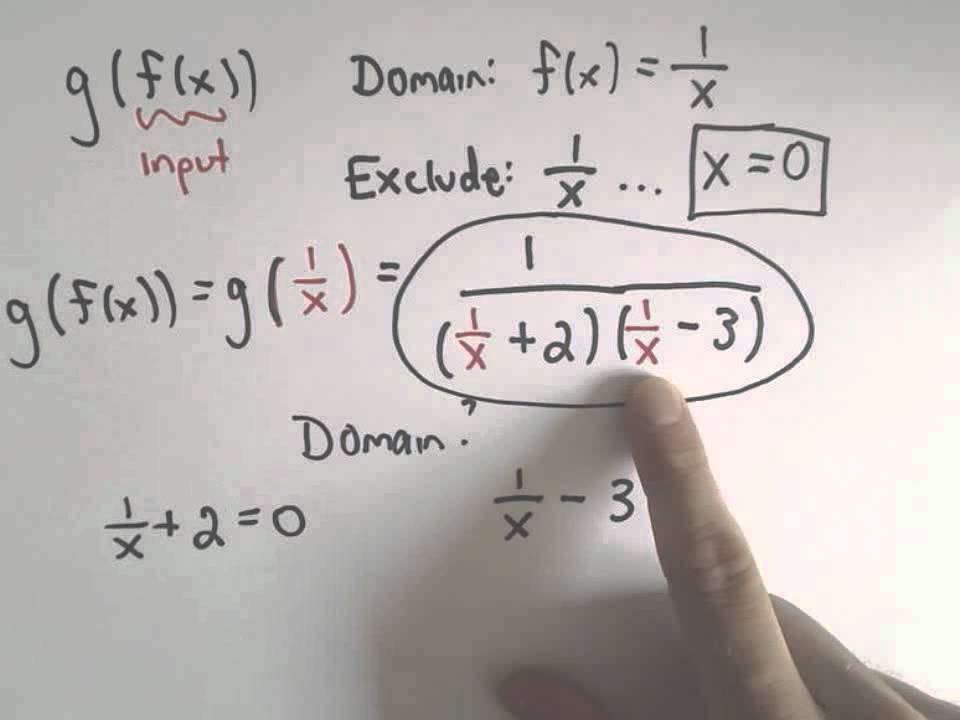
The domain of a composite function consists of those inputs in the domain of the inner function that correspond to outputs of the inner function that are in the domain of the outer function. (g º f) (x) = g (f (x)) = (√x)2. Set f(𝑥) equal to these excluded values and solve for 𝑥 to find the values that must be excluded from the domain. By using this website, you agree to our cookie policy.
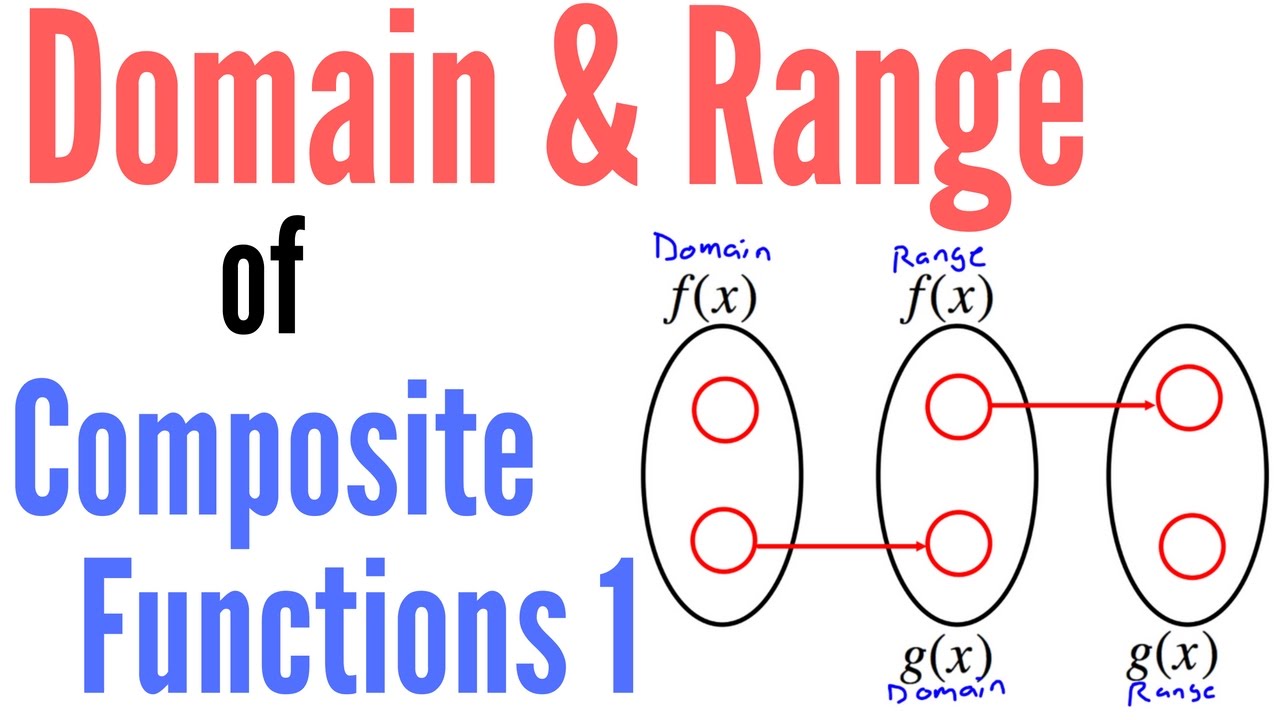
F g domain of g domain of f x g(x) g f (g x)) f ˚ g figure 1.90 the following tables of values help illustrate the composition given in example 4.
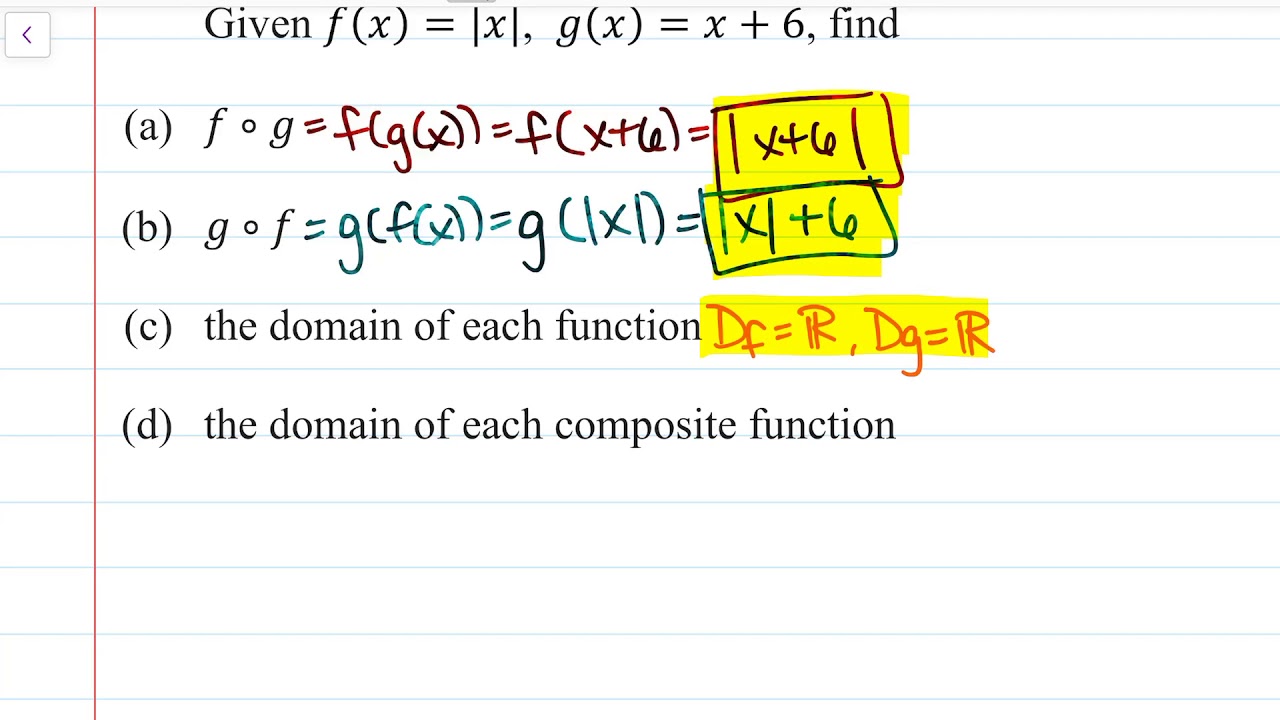
This means that the answers created by these values from function must be “picked up” by function f(x). Thanks to all of you who support me on patreon. We'll be focusing on simple rational functions in this. The composite of two functions f(x) and g(x) must abide by the domain restrictions of f(x) and g(x).
The domain of a composite function is all possible values of the inner function that will yield an output included in the outer function's domain.
A composite function is a function that is dependent on another function. Find the inputs x x in the domain of the inside function g(x) g ( x) for which g(x) g ( x) lies in the domain of f(x). The domain of composite functions is the intersection of the domain of the inside function and the new composite function. Consequently, the composition also cannot pick up the.
The domain of a composite must exclude all values that make the “inside” function undefined, and all values that make the composite function undefined.
In the example above, both functions had domains of all real numbers, so their composite functions did not have any domain restrictions either. There may be hidden obstacles that prevent certain values from completing their trips through a composition, thus eliminating theses values as possible domain elements. Although g(x) = x 2 has a domain of all real numbers, has a. By combining one function within another, a composite function is formed.
Domain of a composite function.
Definition of composition of two functions the composition of the function with the function is the domain of is the set of all in the domain of such that is in the domain of (see figure 1.90.)f. F g x g g x ff g xf g. Let’s follow this process algebraically: Domain of a composition of.
Also in example 2, the domain for f(x) = x2 + 2 is all real numbers.
G(f (x)) g ( f ( x)) evaluate g(x+ 1) g ( x + 1) by substituting in the value of f f into g g. In other words, the domains of each function must. Let's look at an example where domain restrictions apply. Function cannot pick up the value 3.
Find the values that are excluded from the domain of the outer function, g(𝑥).
The expression f(g(x)) can be interpreted as f of g of x. The domain of a composite function [latex]f\left(g\left(x\right)\right)[/latex] is the set of those inputs [latex]x[/latex] in the domain of [latex]g[/latex] for which [latex]g\left(x\right)[/latex] is in the domain of [latex]f[/latex]. The input function f(x) has no restrictions, so the domain of g(f(x)) is determined only by the composite function. So the domain for the composite function is also x ≤ 3.
F (x) = x + 1 f ( x) = x + 1 , g(x) = x2 g ( x) = x 2 , g(f (x)) g ( f ( x)) set up the composite result function.
All this means, is that when we are finding the domain of composite functions, we have to first find both the domain of the composite function and the inside function, and then find where both domains overlap. In other words, given the composite f(g(x)), the domain will exclude all values where g(x) is undefined, and all values where f(g(x)) is undefined. This algebra 2 and precalculus video tutorial provides an introduction basic overview / review on composite functions that contain fractions and radicals suc. The domain of composite functions to calculate the domain of a composite function gf(𝑥):