The domain of a composition will be those values which can move through to the end of the composition. \displaystyle f\circ g f ∘ g, we ask ourselves if there are any further restrictions offered by the domain of the composite function. So, the domain is a set of all values that used in a function and a certain function must work for all given values.
maxresdefault.jpg
To write as a fraction with a common denominator, multiply by.
As we discussed previously, the domain of a composite function such as [latex]f\circ g[/latex] is dependent on the domain of [latex]g[/latex] and the domain of [latex]f[/latex].
Informally, if a function is defined on some set, then we call that set the domain. Find the domain of this new function. For example, a function that is defined for real values in has domain , and is sometimes said to be a function over the reals. the set of values to which is sent by the function is called the range. The composite may also result in a domain unrelated to the domains of the original functions.
It is important to know when we can apply a composite function and when we cannot, that is, to know the domain of a function such as [latex]f\circ g[/latex].
The obstacle is whether all of the values created by g(x), in this case, can be picked up by function f (x). Let’s examine what happens to values as they “travel” through a composition of functions. Algebraic interpretation of this example: Function g ( x) cannot pick up the value +2 since it creates a zero denominator.
Find the domain of the inner function g(x) (let this be a)
By using this website, you agree to our cookie policy. But when the functions are defined algebraically, here are the steps to find the domain of the composite function f(g(x)). Evaluate by substituting in the value of into. The domain of composite functions is the intersection of the domain of the inside function and the new composite function.
Get the free composite function calculator widget for your website, blog, wordpress, blogger, or igoogle.
You can use desmos to explore composite functions. X → y and f : Enter the function you want to domain into the editor. In general, if g :
The domain of this function is.
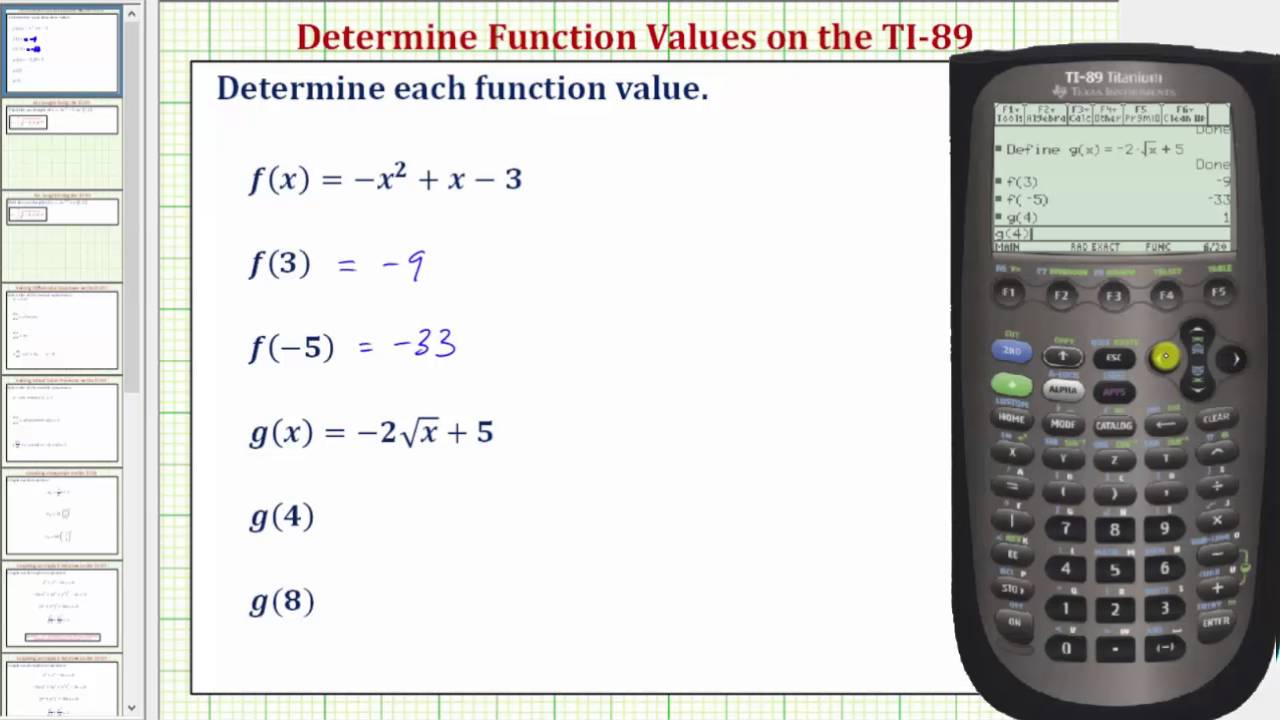
To find the domain of. F x = x + 2. The domain calculator allows you to take a simple or complex function and find the domain in both interval and set notation instantly. If there are restrictions on this domain, add them to the restrictions from step 1.
The values taken by the function are collectively referred to.
G x = x 2. If there is an overlap, use the more restrictive domain (or the intersection of the domains). The answer is no, since. The domain of a composite must exclude all values that make the “inside” function undefined, and all values that make the composite function undefined.
Write each expression with a common denominator of , by multiplying each by an appropriate.
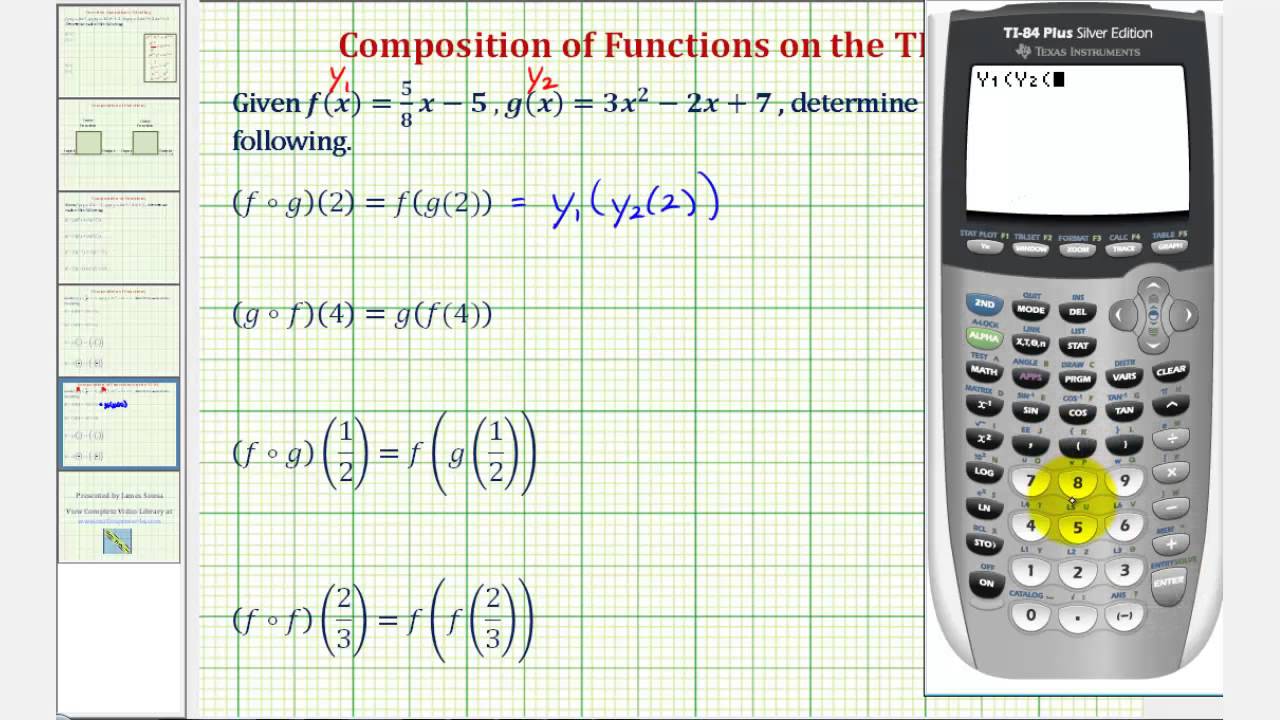
F(x)=2x+1, g(x)=x+5, find fog(x) 2. You can then enter a composite functions such as fg(x) and gf(x) 1. At times, the domain of a composite function can be a bit confusing. In other words, given the composite f(g(x)), the domain will exclude all values where g(x) is undefined, and all values where f(g(x)) is undefined.
Y → z then f ∘ g :
The domain of composite functions g (f(x)) is always dependent on the domains of the g(x) function and the domain of the f(x) function. Also, the composite function calculator determines the function from domain of composite. The cool thing is that the result is a brand new function, with it’s own domain and range. Find the domain (fog)(x) , f(x)=1/(x+3) , g(x)=2/x.
Use of the composition calculator.
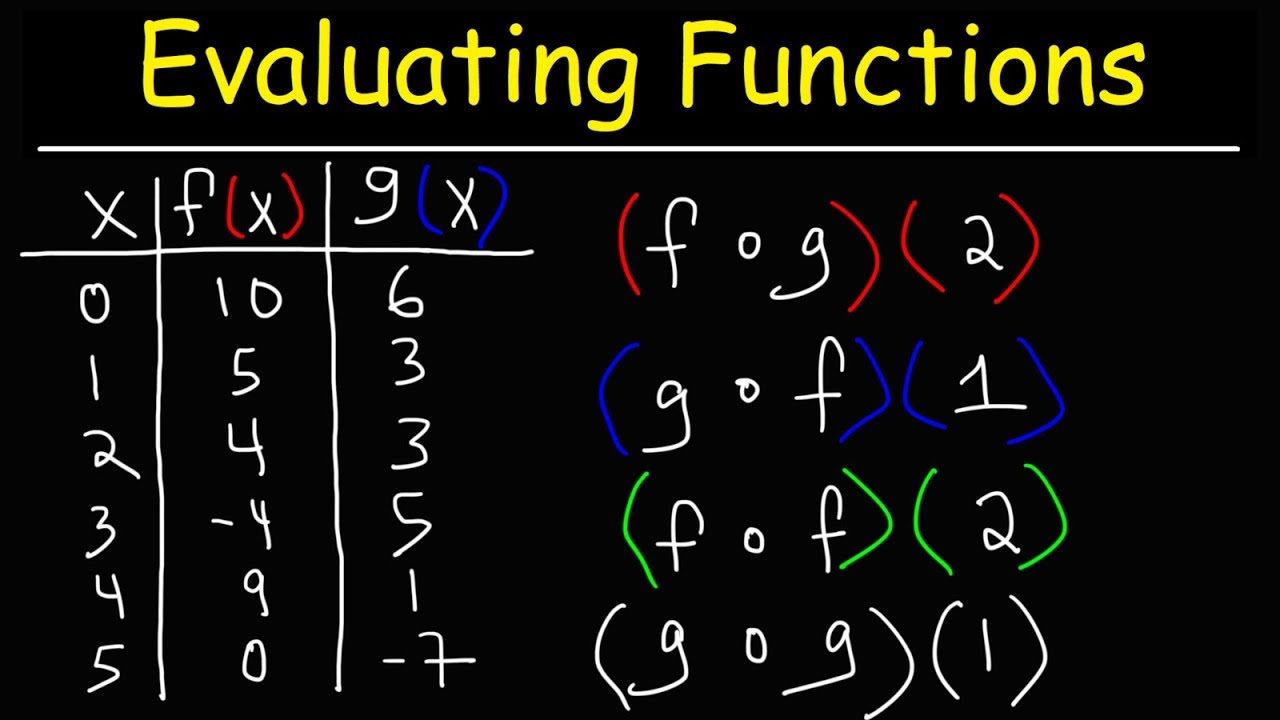
Finding domains of composite functions. Composite functions and evaluating functions : Use this calculator to obtain the composite function fg (x) use # as the variable. I.e., the domain of f ∘ g is x and its range is z.
All this means, is that when we are finding the domain of composite functions, we have to first find both the domain of the composite.
Click the blue arrow to submit and see the result! Find more education widgets in wolfram|alpha. Set up the composite result function. Note that f(x) and g(x) have been defined first;