Find the domain of this new function. The range is also [0, ∞). Below is a summary of what we've seen.
Composite functions
Composition of functions can make both the domain and the range of functions change in strange ways.
The domain is r = ( − ∞, ∞) and its range is [ − 1, 1].
Procedure must be used to find the composite domain. This answer, unlike my previous answer, assumes that f ( x) = x 2 is the restricted function with domain [ 0, ∞). You are right about g ( f ( x)): The input function f(x) has no restrictions, so the domain of g(f(x)) is determined only by the composite function.
What are the domain and range of composite functions?
To find the domain of the composite function $$( f \circ g )(x)=f(g(x))$$ one can follow these two steps. Therefore, the domain of g ( x) g (x) g ( x) is all real numbers x x x such that x ≠ 5 x \neq 5 x ≠ 5. It also shows that the domain of [latex]f\circ g[/latex] can contain values that are not in the domain of [latex]f[/latex], though they must be in the domain of [latex]g[/latex]. Find the domain of the inside (input) function.
In general, if g :
A useful tool is a composition of functions (or composite function), which we can describe in one sense as a function of a function. consider two functions f(x) and g(x). This example shows that knowledge of the range of functions (specifically the inner function) can also be helpful in finding the domain of a composite function. The implied domain of the composite function $\csc(\arctan x)$ is the largest subset of the domain of $\arctan x$ that maps to elements in the domain of $\csc x$. If there are restrictions on this domain, add them to the restrictions from step 1.
Section 1.8 combinations of functions:
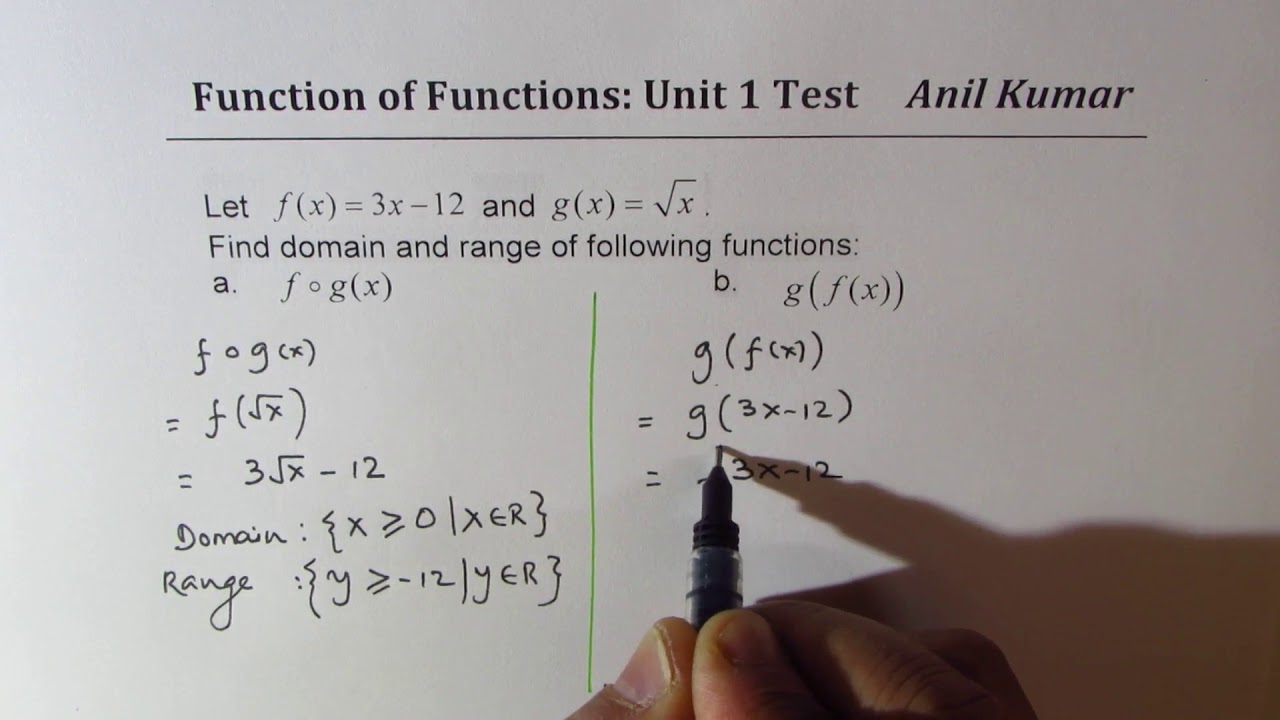
(f ∘g)(x) wheref (x) = √x+2 and g(x) = √3−x ( f ∘ g) ( x) where f ( x) = x + 2 and g ( x) = 3 − x. Let f x x( ) ln 2 2 for all , and let g x e() x for all xt0. F o g = 2 x 2 + 1. Since g ( x) = sin.
{y | y = 2x 2 + 1 and x ∊ n} g o f = g[f(x)] = g[2x + 1] now we apply 2x + 1 instead of x in g(x).
For example the element {2} is a part of the range but {6} is not. Worksheets are domain and range work 1 name, artifacts examples domain 1 planning and preparation, domain and range ordered pairs 1, domain and range graph 1, domain and range packet, composite functions and their domains, work 1 functions and inverse functions, name date ms. So, y = f ( x) z = g ( y) a n d z = g ∘ f ( x) the domain of the composite function is the domain of f (i.e set a) and the range of the composite function is the range of g. The composite range of is f ,0@.
Let's look at an example where domain restrictions apply.
Basically, since s x x( ) ln cos and the composite domain is , you are taking the natural log of numbers between 0 and 1. Y → z then f ∘ g : Y = 2x 2 + 1. F o g = f[g(x)] = f[x 2] now we apply x 2 instead of x in f(x).
As for f ( g ( x)), it is defined whenever g ( x) ≥ 0, so in contrast with what you wrote it does sometimes exist.
Although g(x) = x 2 has a domain of all real numbers, has a. What should you get out of this, please? I.e., the domain of f ∘ g is x and its range is z. But when the functions are defined algebraically, here are the steps to find the domain of the composite function f(g(x)).
In this video i will introduce you to finding the domain and range of a composition of two functions.
The composition ln(sin(x)) does not have the same range as just ln. You may also think about the graph of. Composite functions 87 finding the domain of a composite function given and find the composition then find the domain of solution from this, it might appear that the domain of the composition is the set of. That means x = 5 x =5 x = 5 isn’t in the domain of g ( x) g (x) g ( x).
The domain of a function is the set of all acceptable inputs, whereas the range of.
About press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features press copyright contact us creators. Find the range of f o g and g o f. G o f = (2 x + 1) 2. So the domain for the composite function is also x ≤ 3.
We'll be focusing on simple rational functions in this.
Let hx() be the composition of A → b and another function g: In the example above, both functions had domains of all real numbers, so their composite functions did not have any domain restrictions either. Find the domain of the inner function g(x) (let this be a)
Composition of functions can make both the domain and the range of functions change in strange ways.
The composite of two functions f(x) and g(x) must abide by the domain restrictions of f(x) and g(x). Because we cannot take the square root of a negative number, the domain of g g is (. X → y and f : Then, we have a composite function g ∘ f: