Physical sciences index classical mechanics index: Finds mass or velocity after collision. Any objects that collide in this way will reduce the total kinetic energy (and total velocity) by this ratio.
Lesson 7 ELASTIC & INELASTIC COLLISIONS WillowWood Lessons
V 1i +v 1f = v 2f +v 2i eqn.
After the collision, we can observe that the ball completely loses its energy, and the speed of the ball is reduced drastically.
If it is 1 we have an elastic collision; Since the momentum equation is easier, lets use that. M 1 v 1i 2 −v 1f (2) m 1 (v 1i −v 1f) = m 2 v 2f 2 −v 2i (2) m 2 (v 2f −v 2i) after all the cancellations, we are left with: Coefficient of restitution generally lies between 0 and 1.
Macroscopic collisions are generally inelastic and do not conserve kinetic energy, though of course the total energy is conserved as required by the general principle of conservation of energy.the extreme inelastic collision is one in which the colliding objects.
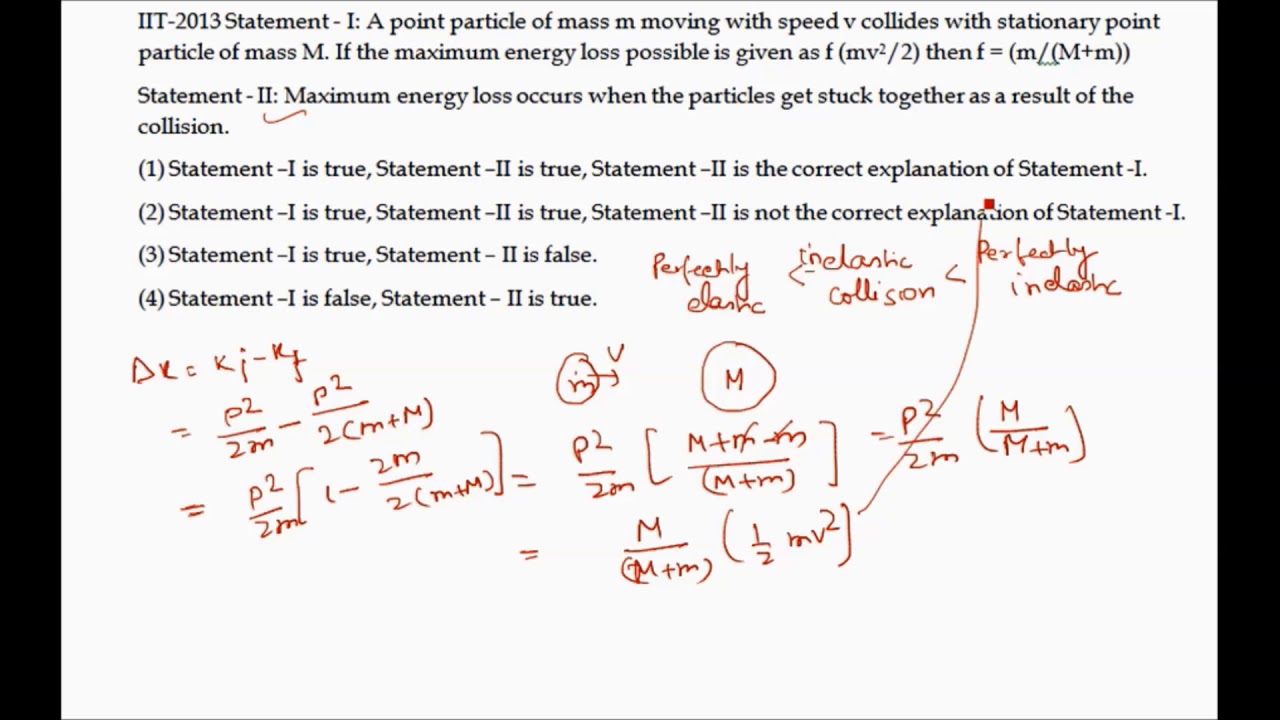
Kf / ki = m1 / ( m1 + m2 ) some basic mathematical analysis will allow you to look at the expression m1 / ( m1 + m2) and see that for any objects with mass, the denominator will be larger than the numerator. The most general case of a completely inelastic collision is two particles m 1 and m 2 moving at an angle of θ 1 to each other with velocities v 1 and v 2, respectively. Velocity of the moving object, in m/s. In fact, such a collision reduces internal kinetic.
4 now we take equation 4 and substitute back into one of our original equations to solve for v 2f.
Most collisions in nature are inelastic collisions. The change in momentum for this is given by δp = p f − p i = (m 1 +m 2)v f −(m 1 v 1i +m 2 v 2i) = 0 (equation 6) and the change in kinetic energy is given by ) 0 2 C r is the coefficient of restitution; The two objects come to rest after sticking together, conserving momentum.
Collisions involve forces (there is a change in velocity).
The formula for inelastic collision: Mass of object 1 × initial velocity 1 + mass of object 1 × initial velocity 1 = (mass of 1 + mass of 2) × final velocity of combined objects) in. Inelastic collisions perfectly elastic collisions are those in which no kinetic energy is lost in the collision. An inelastic collision is any collision between objects in which some energy is lost.
The inelastic collision equation is:
The parameters of the collision, including mass, velocity, and. Inelastic collision formula when two objects collide with each other under inelastic conditions, the final velocity of the object can be obtained as; These include balls that produce elastic collisions, balls that produce completely inelastic collisions, and balls that have different masses, materials, and initial velocities. In a perfectly inelastic collision, the colliding particles stick together.
Students will produce collisions between balls with different properties in a wide range of scenarios.
= 1/2 (100,000 kg)(3 m/s)2. But the internal kinetic energy is zero after the collision. 3 solving for v 1f we get: A collision in which the objects stick together is sometimes called a perfectly inelastic collision because it reduces internal kinetic energy more than does any other type of inelastic collision.
The inelastic collision formula is articulated as.
V 1f = v 2f +v 2i −v 1i eqn. Mass of the stationary object, in kg. Mass of the moving object, in kg. • energy is not conserved in a perfectly inelastic collision.
If it is 0 we have a perfectly inelastic collision, see below.
A special case of this is sometimes called the perfectly inelastic collision. In a perfectly inelastic collision, two objects collide and stick together. Coefficient of restitution is 0 for the perfectly inelastic collision. Mass of body 2 = m 2 the initial velocity of body 1 = u 1 the initial velocity of body 2 = u 2 the final velocity of both the bodies = v.
If one body (a) with a velocity (a) strikes a second body (b) and sticks to it, then the resulting larger body will have a slower velocity.
Where mass of body 1 = m 1. • if the objects bounce apart instead of sticking together, the. Reactive scattering lif + h !li + hf in an electric field. In a perfectly inelastic collision, the two collided bodies stick together and move with the same velocity v.
Kinetic energy is lost in an inelastic collision but the net energy of the colliding system remains the same.
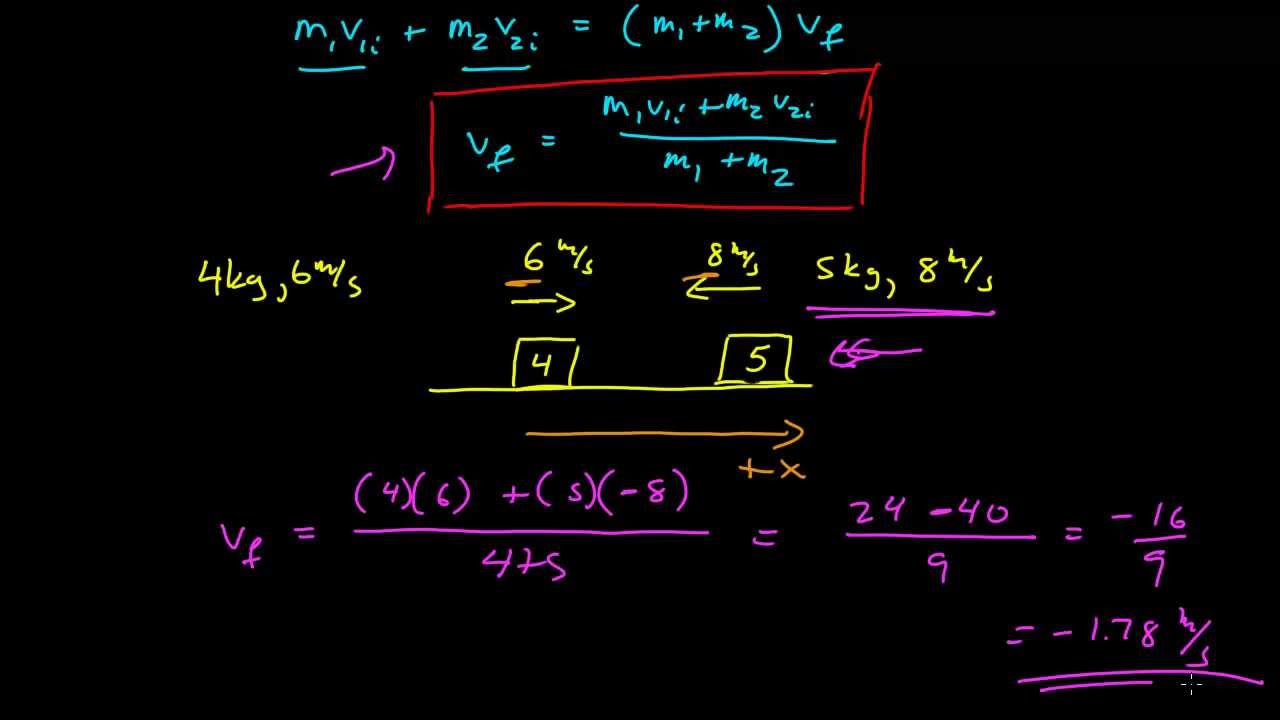
V = m 1 u 1 +m 2 u 2 m 1 +m 2. Velocity of the stationary object after collision, in m/s. Divide equation 1 by equation 2. A perfectly inelastic collision (or completely inelastic collision) is a specific case of inelastic collision in which the two colliding body stick together and move as one mass after the collision.
Elastic and inelastic scattering of nh(3) molecules with mg(1s) atoms in a magnetic field;
The momentum of the objects before the collision is conserved, but the total energy. Given data, u=3 m/sv=5 m/s∆t=6 s so the formula for velocity is given as;