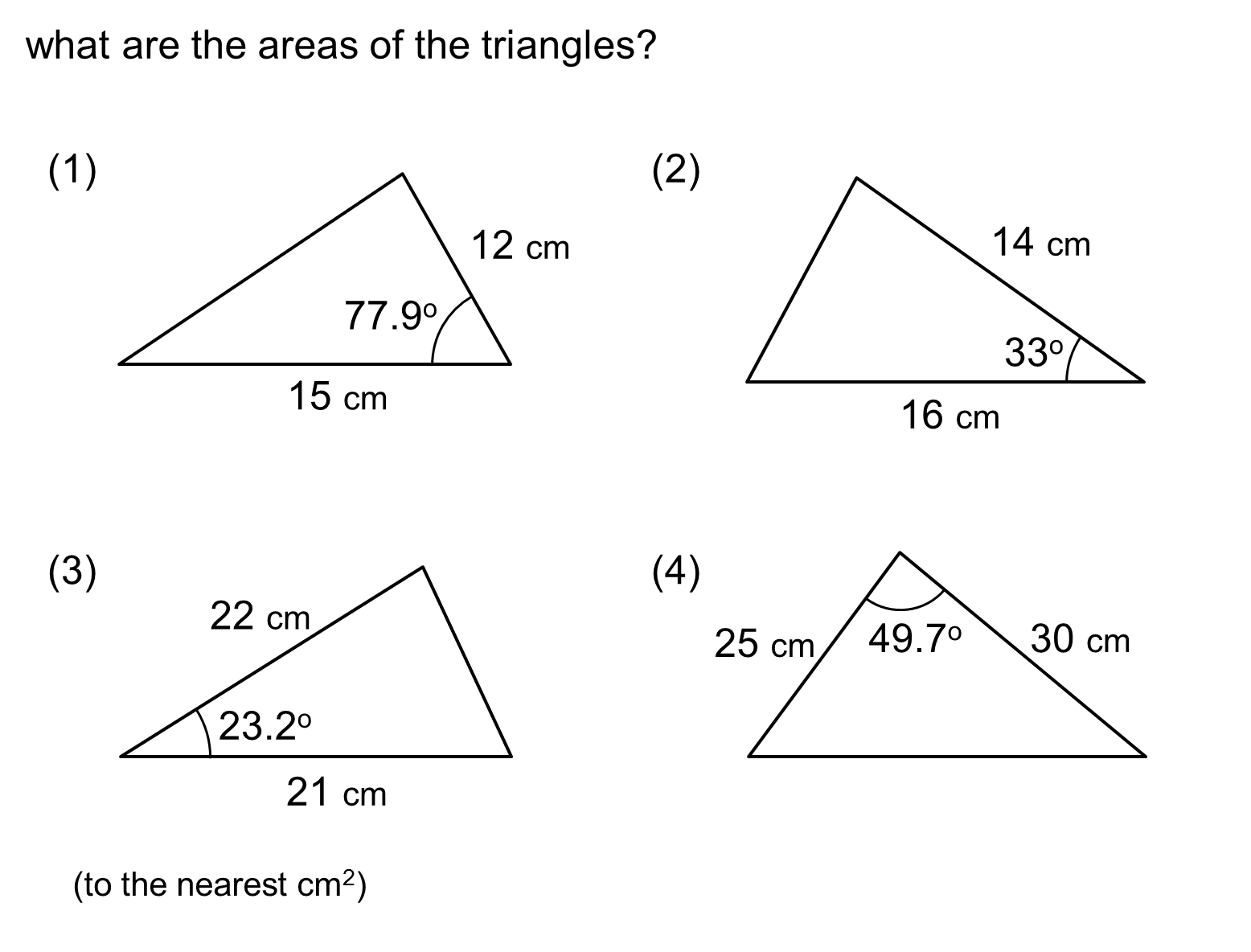
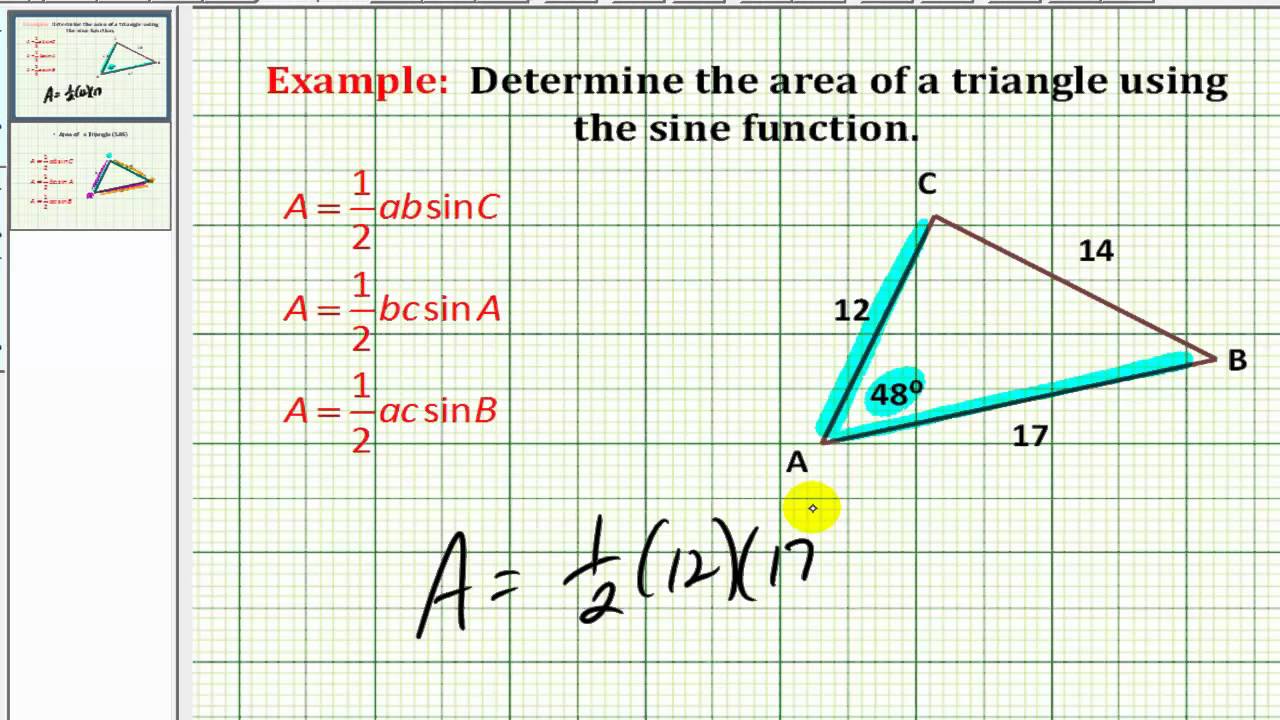
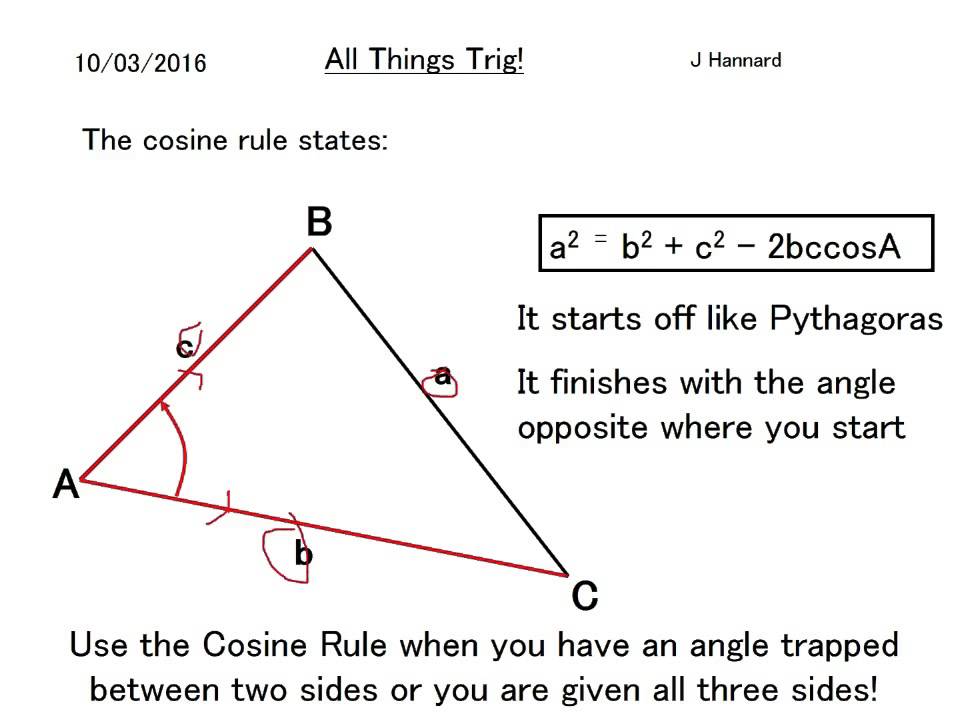
\frac{a}{sin(a)} = \frac{b}{sin(b)} = \frac{c}{sin(c)} cosine rule: Cosine rule (the law of cosine): Area = ½ ab sin c
PPT The Sine Rule The Cosine Rule PowerPoint
Sina a = sinb b = sinc c.
Area = 1 2 bcsin ^a = 1 2 (7)(7)sin80° = 24.13 area = 1 2 b c sin.
Area ∆pqs = ½ qs sin p Walkthrough examples followed by practice. Another formula that can be used to obtain the area of a triangle uses the sine function. 6.89 sin100 sin 0.5219 13 d so d = 31.5° a b c 7 cm 12 cm 30o b a c a c d 13 cm 100o 6.89 cm a c d
In abc a b c:
The area of any other triangle can be found with the formula below. Sine rule (the law of sine): The sine rule tells us that: Area of abc = 1 2absinc.
In ∆pqs ps = 9,24 sin42.45° sin95,1 ps = 9,24 sin 42,45° sin95.1 ps = 6,26 cm (6) to find the areas of pqrs, find the area of the two triangles and add them together.
The area rule states that the area of any triangle is equal to half the product of the lengths of the two sides of the triangle multiplied by the sine of the angle included by the two sides. (1.2) for a fishing trip on a lake, a boat leaves  pier 1 on a bearing of 227 °, to the spot where fishing will commence. The cosine rule tells us that: Find the area of triangle pqr if p = 6.5 cm, r = 4.3 cm.
H sin(38∘) h s i n ( 3 8 ∘) \bf {\frac {\boldsymbol {\tt h}} {sin (38^\circ)}} sin(38∘)h.
Cos 90° = 0 so if a = 90°, this becomes pythagoras’ theorem; = 12.8 ( × sin38°  both sides ) h = 12.8 × sin ( 38 °) = 7.9 cm. (b) calculate the area of the triangle abc. The area of any triangle can be calculated using the formula:
Notice that we do not know the length of side a a and must therefore choose the form of the area rule that does not include this side of the triangle.
Enter sides a and b and angle c in degrees as positive real numbers and press enter. Use the area rule to calculate the area of abc a b c. So, we use the sine rule to find unknown lengths or angles of the triangle. A = b = c.
Remember that the given angle must be between the two given sides.
The area of a triangle formula tells us that: As is the case with the sine rule and the cosine rule, the sides and. Whether you want a homework, some cover work, or a lovely bit of extra practise, this is the place for you. Now we can find ps using the sine rule and ^p.
Area = (1 / 2) b c sin(a) = (1 / 2) c a sin(b) = (1 / 2) a b sin(c) how to use the calculator here we assume that we are given sides a and b and the angle between them c.
To find the area of ∆pqs, use ^p = 95,1° and ps = pq = 6,26 cm. The students have to then put the cards in order showing how the formula for the area of a triangle using sine has been developed. The area area of a triangle given two of its sides and the angle they make is given by one of these 3 formulas: The area of a triangle using sine.
This formula works for a right triangle as well, since the since of 90 is one.
\sin a = \frac { (\text {opposite})} { (\text {hypotenuse})} = \frac {h} {b} sina= (hypotenuse)(opposite). The diagram shows triangle abc. Sin sin sinda c da c substituting into this formula gives: \[\text{area of a triangle} = \frac{1}{2} ab \sin{c}\]
As a consequence of the law of sine, we can neatly put a formula for the area of a triangle:
It means that sin a/a, instead of taking a/sin a. (\text {area}) = \frac {1} {2} ch. (sin a/a) = (sin b/b) = (sin c/c) in this case, the fraction is interchanged. Arrow_back back to sine rule, cosine rule and area of a triangle sine rule, cosine rule and area of a triangle:
Up to10%cash back · finding the area of a triangle using sine you are familiar with the formula r = 1 2 b h to find the area of a triangle where b is the length of a base of the triangle and h is the height, or the length of the perpendicular to the base from the opposite vertex.
Area of triangle = ½ ab sinc. ( area) = 1 2 c h. Up to 10% cash back sin ( a ) = opposite side hypotenuse = h c sin ( a ) = h c ⇒ h = c sin ( a ) substituting the value of h in the formula for the area of a triangle, you get r = 1 2 b (. Sin sin 6.89 da a sin100 13 rearranging gives:
It allows us to find the area of a triangle when we know the lengths of two sides and the size of angle between them.
Secure learners will be able to find a missing length or angle in a scalene triangle given its area. If a, b and c are the lengths of the sides opposite the angles a, b and c in a triangle, then: (a) find the size of pâr. A = \frac{1}{2} a b sin(c).
It is also called as sine rule, sine law or sine formula.
Excelling learners will be able to solve unfamiliar problems using the formula for the area of a scalene triangle. Now, sin a = ( opposite) ( hypotenuse) = h b. The two attached word documents are two versions of a match up type activity. C c to the opposite side, we know that the area of the triangle is.
While finding the unknown angle of a triangle, the law of sines formula can be written as follows: