Types isosceles equilateral right angle how to calculate | studysmarter original. Up to 10% cash back finding the area of a triangle using sine. The area of a right triangle is the region covered by its boundaries or within its three sides.
Triangle Area NonRight Triangles Pt 1 YouTube
Area = a * a * tan(β) / 2 = a² * tan(β) / 2.
Where b and h refer to the.
You are familiar with the formula r = 1 2bh to find the area of a triangle where b is the length of a base of the triangle and h is the height, or the length of the perpendicular to the base from the opposite vertex. Answering the question given amounts to finding side a in this new triangle. Up to 10% cash back the formula for the area of a triangle is where is the base of the triangle and is the height. The formula to find the area of a right triangle is given by:
How to find the area of a right triangle with one formula
This indicates how strong in your memory this concept is. Area = ½ ab sin c. The pythagorean theorem, a2+b2=c2, a 2 + b 2 = c 2 , can be used to find the length of any side of a right triangle. Now, you’ve solved the formula for the area of a right triangle.
Obviously using both a tangent calculator and an exponent calculator is quite helpful.
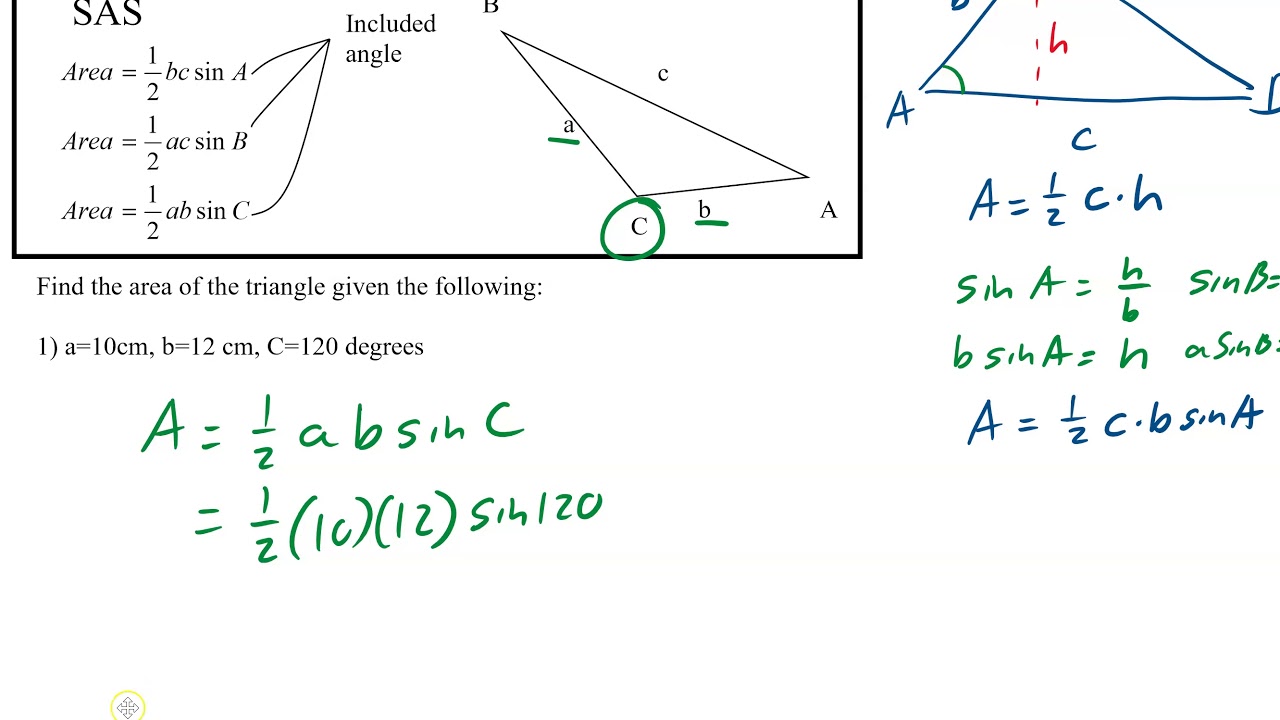
The height is b × sin a. According to the law of sines, the ratio of the measurement of one of the angles to the length of its opposite side equals the other two ratios of angle measure to opposite side. There are three possible cases: Area = 1 2 bc sin a.
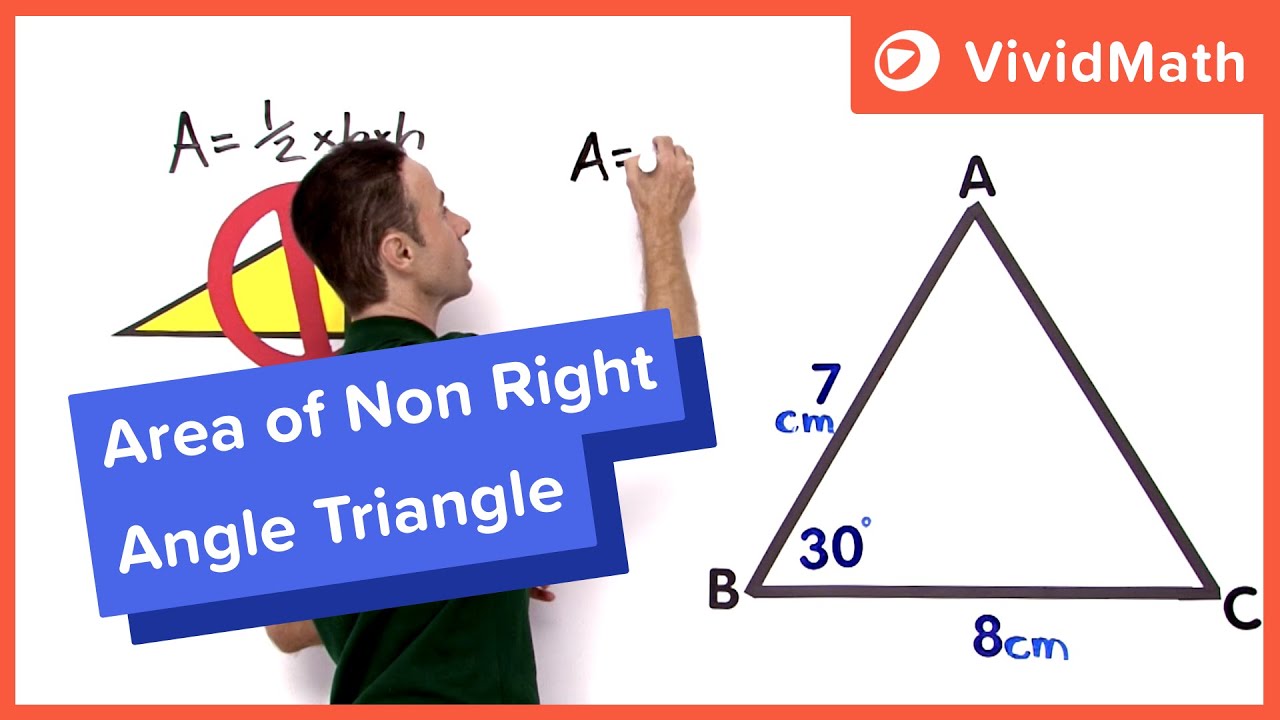
Area = 0.5 * a * b * sin (γ) two angles and a side between them (asa)
Once the length of the base and height have been multiplied, divide them in half. Area equals half the product of two sides and the sine of the included angle. If you've just noticed that your triangle is not a right triangle,. Area equals half the product of two sides and the sine of the included angle.
Therefore, the area is equal to or, based on the units given, 42 square centimeters
This usually requires us to draw a line, called height or altitude, from one vertex of the triangle to the side opposite it, which is perpendicular to that side. Area = b * tan(α) * b / 2 = b² * tan(α) / 2. To be noted, the base and height of the triangle are perpendicular to each other. The general formula for the area of a triangle is the base times the height to that base, divided by two.
English (de) english (uk) find study materials create study materials.
The base and the height. The area of any other triangle can be found with the formula below. Area = ½ × (c) × (b × sin a) which can be simplified to: Heron’s formula finds the area of oblique triangles in which sides a,b, a, b, and c c are known.
Area = ½ × base × height.
The area of the triangle is 88.47. To find the area of the triangle, you must multiply the hypotenuse’s two adjacent sides: Area of a right triangle = 1 2bh a r e a o f a r i g h t t r i a n g l e = 1 2 b h. We know the base is c, and can work out the height:
It is applicable to all types of triangles, whether it is scalene, isosceles or equilateral.
Suppose δabc has side lengths a , b , and c. Let h be the length of the. For the triangle shown, side is the base and side is the height. This formula is derived from the area of a triangle formula, a=1/2bh for any triangle abc with side a opposite a, side b opposite b and side c opposite c, height h is represented
The side opposite the right angle is called the hypotenuse (side c in the figure).
Here it is as an equation: