∫ ∫u dv uv v du= − _____ ( ) [ ] ( ) 2 Up to 24% cash back integral calculus all formulas pdf. 2 1 sin ( ) 1 cos(2 )x 2 sin tan cos x x x 1 sec cos x x cos( ) cos( ) x x 22sin ( ) cos ( ) 1xx 2 1 cos ( ) 1 cos(2 )x 2 cos cot sin x x x 1 csc sin x x sin( ) sin( ) x x 22tan ( ) 1 sec ( )x x geometry fomulas:
Maths Formula Integration Formula for HSC Board HSC
R 1 xlnx dx solution.
Rational function, except for x’s that give division by zero.
Derivatives calculus lessons indefinite integrals the notation is used for an antiderivative of f and is called the indefinite integral. Strip 2 secants out and convert rest Symbols f(x) → integrand f(x)dx → element of integration ∫→ sign of integral φ(x) → anti. Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2;
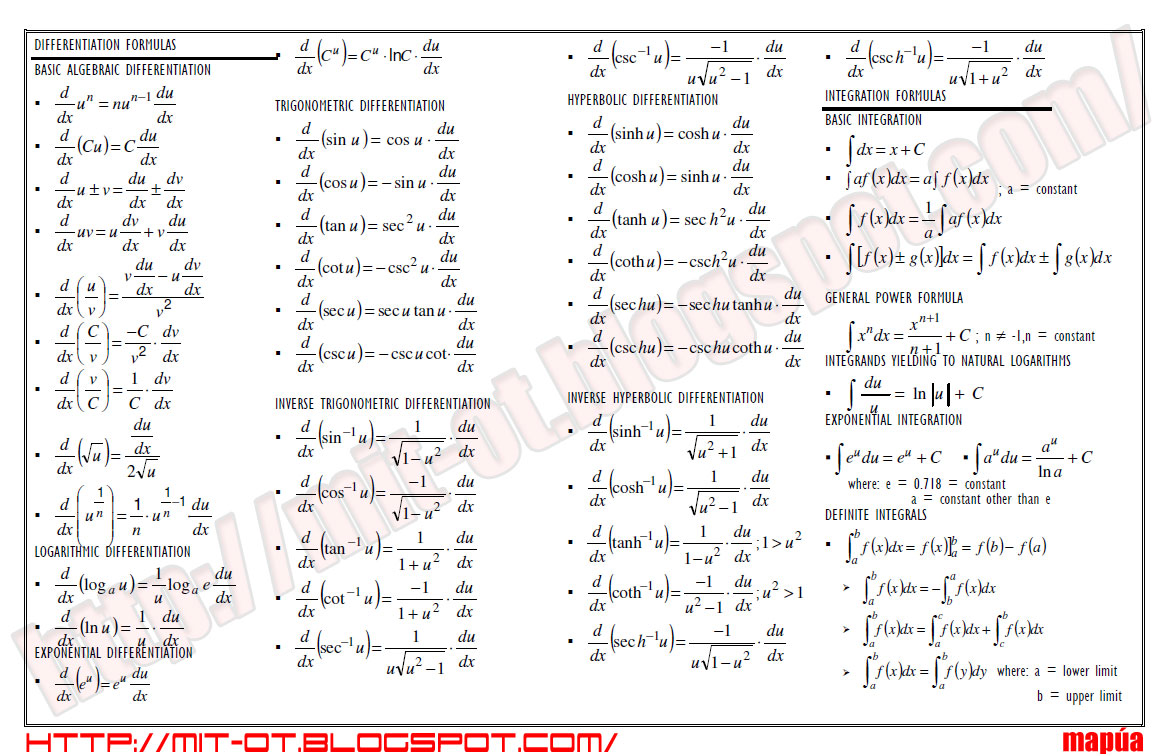
Therefore, it is essential to know the formula as well as the differential to solve the questions of integration.
Adapted from notes by nancy stephenson, presented by joe milliet at tcu ap calculus institute, july 2005 ap calculus formula list math by mr. Integration formulas is the process with. 2) if fxcc 0 for all x in i, then the graph of f is concave downward in i. The following is a table of formulas of the commonly used indefinite integrals.
Since calculus plays an important role to get the.
Integration formula calculus formula is a most using formula for calculus in mathematic actually, integration is the reverse process of differentiation. Cos x and sin x for all x. Cot x and csc x provided N x (n even) for all x 0.
= ln |x| + c trigonometric integrals 1.
3 2;cos2 ax (65) z. N x (n odd) for all x. Indefinite integral :∫f x dx f x c( ) = +( ) Differentiation is an important topic of class 12th mathematics.
Interpret the constant of integration graphically.
All important calculus formulas pdf. Common integrals v clx = kx+c idx=lnlxl+c l in c uln (u) —u + c ax +1) on u du = for vann xsecl xdx we have the following : We have different methods to find the integral of a given function in integral calculus. Similar to differentiation formulas, we have integral formulas as well.
It has two large branches, differential calculus concerning the rates of change and the slopes of curves, and integral calculus on the accumulation of quantities and.
Mueller page 5 of 6 calculus bc only integration by parts: Integration formulas z dx = x+c (1) z xn dx = xn+1 n+1 +c (2) z dx x = ln|x|+c (3) z ex dx = ex +c (4) z ax dx = 1 lna ax +c (5) z lnxdx = xlnx−x+c (6) z sinxdx = −cosx+c (7) z cosxdx = sinx+c (8) z tanxdx = −ln|cosx|+c (9) z cotxdx = ln|sinx|+c (10) z secxdx = ln|secx+tanx|+c (11) z cscxdx = −ln |x+cot +c (12) z sec2 xdx = tanx+c (13) z csc2 xdx = −cotx+c (14) z The most commonly used methods of integration are: Ex dx = ex + c 6.
Now z4 2 xsin(x2)dx = − 1 2 cos(x2) 4 2 = − 1 2 cos(16)+ 1 2 cos(4).
Up to 24% cash back ap calculus ab & bc formula list. Divide [ab,] into n subintervals of width ∆x and choose * x i from each interval. Ax dx = + c, and 0, a1 7. R lnx2 x dx solution.
The differential calculus splits up an area into small parts to calculate the rate of change.the integral calculus joins small parts to calculates the area or volume and in short, is the method of reasoning or calculation.in this page, you can see a list of calculus formulas such as integral formula, derivative formula, limits formula etc.
Suppose f x( ) is continuous on [ab,]. Substituting u = x4 −x2 +6and 5 2 du =(10x3 −5x)dx,you get z 10x3 −5x √ x4 −x2 +6 dx = 5 2 z 1 √ u du = 5 2 z u−1 2 du = 5 2 ·2u12 +c = =5 √ x4 −x2 +6+c. Up to 24% cash back all integration and differentiation formulas pdf calculus is one of the branches of mathematics involved in the study of the change rate and their application in the resolution of equations. Integration as inverse operation of differentiation.
Differentiation formulas pdf class 12:
A somewhat neater alternative to this method is to change the original limits to match the variable u. If d/dx {φ(x)) = f(x), ∫f(x)dx = φ(x) + c, where c is called the constant of integration or arbitrary constant. Then z xsin(x2)dx = z 1 2 sinudu = 1 2 (−cosu)+c = − 1 2 cos(x2)+ c. Let us go ahead and look at some of the integral calculus formulas.
A s2 1 area of a triangle:
7.1 indefinite integrals calculus learning objectives a student will be able to: Since u = x2, when x = 2, u = 4, and when x = 4, u = 16. Tan x and sec x provided 33,,,,, 2222 x 9. Methods of finding integrals of functions.
Equation of a plane a point r (x, y, z)is on a plane if either (a) r bd= jdj, where d is the normal from the origin to the plane, or (b) x x + y y + z z = 1 where x,y, z are the intercepts on the axes.
For all x in i, then the graph of f is concave upward in i. K dx = kx + c 4. Functions ∫sin cosxdx x= − ∫cos sinxdx x= − sin sin22 1 2 4 x ∫ xdx x= − cos sin22 1 2 4 x ∫ xdx x= + sin cos cos3 31 3 ∫ xdx x x= − cos sin sin3 31 3 ∫ xdx x x= − ln tan sin 2 dx x xdx x ∫= Then ( ) (*) 1 lim i b n a n i f x dx f x x →∞ = ∫ =∑ ∆.
_____ definition of an inflection point:
R √10x3−5x x4−x2+6 dx solution. Definite integrals 81 riemann sums 86 rules of definite integration 86 fundamental theorems of calculus 87 properties of definite integrals 88 solving definite integrals with directed line segments 89 u‐subsitution 91 special techniques for evaluation 93 derivative of an integral A function f has an inflection point. You can verify any of the formulas by differentiating the.
Kƒ(x) dx = k ƒ(x) dx 2.
Ln x for x 0. Substituting u =lnx and du = 1 x dx,youget z 1 xlnx dx = z 1 u du =ln|u|+c =ln|lnx|+c. Up to 24% cash back basic integration formulas general and logarithmic integrals 1. Differentiation is an important concept in calculus, on the other hand integration also involves the usage of differentiation formulas and concepts to solve the integration questions.