The integration of a function f (a) is given by f (a) and is written as. A definite integral is used to compute the area under the curve So to integrate a function f(x), you write ∫ f (x)dx
26+ All Differentiation Formulas Pdf PNG Ugot
Because the derivative of a constant function is always zero, so the differentiation process eliminates the ‘c’.
Knowing which function to call u and which to call dv takes some practice.
Z 1 p 1 x2 dx= sin 1 x+ c z 1 x2 + 1 dx= tan 1x+ c. Actually, there exist infinitely many anti derivatives of each of these functions which Derivative of a constant, a: 0 0 sin sin 1 cos lim 1 lim 0 lim 0 x x x x x x −> −>∞ −>x x x − = = =
Here is a general guide:
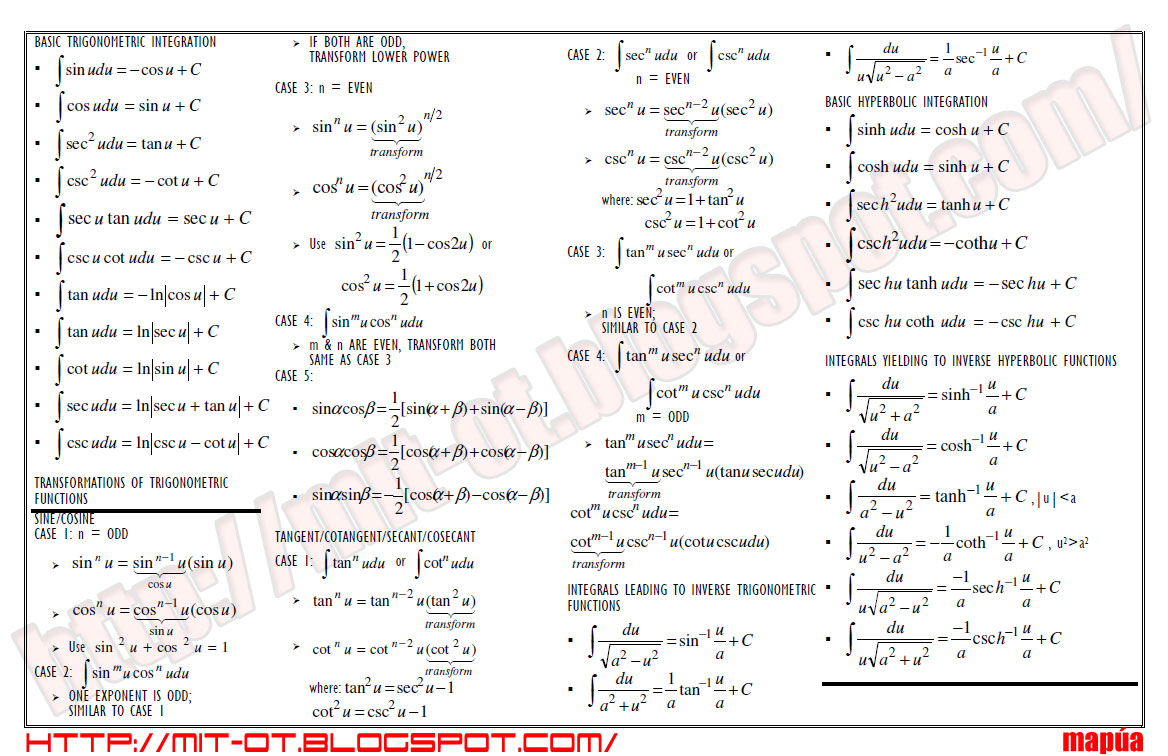
F (a) is called primitive, f (a) is called the integrand and c is constant of integration, a is variable. Product rule (f(x) g(x)) quotient rule — (x) g(x) + f (x) g' (x) dx g (x) chain rule = (g(x)) g' (x) first derivative rule f'(x) = lim f g(x) — f (x) g' (x) d(cosec dx x) x2 _. The above formulas for the the derivatives imply the following formulas for the integrals. Integration formulas y d a b x c= + −sin ( ) a is amplitude b is the affect on the period (stretch or shrink) c is vertical shift (left/right) and d is horizontal shift (up/down) limits:
Differentiation and integration rules a derivative computes the instantaneous rate of change of a function at different values.
∫a→b f (x) dx = ∫a→c f (x) dx + ∫c→b f (x) dx. U inverse trig function (sin ,arccos , 1 xxetc) logarithmic functions (log3 ,ln( 1),xx etc) algebraic functions (xx x3,5,1/, etc) trig functions (sin(5 ),tan( ),xxetc) Differentiation is an important concept in calculus, on the other hand integration also involves the usage of differentiation formulas and concepts to solve the integration questions. Both f and g are the functions of x and differentiated with respect to x.
If d/dx {φ(x)) = f(x), ∫f(x)dx = φ(x) + c, where c is called the constant of integration or arbitrary constant.
∫a→b f (x) dx = ∫a→b f (t) dt. An indefinite integral computes the family of functions that are the antiderivative. Functions ∫sin cosxdx x= − ∫cos sinxdx x= − sin sin22 1 2 4 x ∫ xdx x= − cos sin22 1 2 4 x ∫ xdx x= + sin cos cos3 31 3 ∫ xdx x x= − cos sin sin3 31 3 ∫ xdx x x= − ln tan sin 2 dx x xdx x ∫= Some of the general differentiation formulas are;
Laws of exponential functions and logarithms functions ax ·ay = ex+y log a.
Identities of trigonometric functions tanx = sinx cosx cotx = cosx sinx secx = 1 cosx cscx = 1 sinx sin2 x+cos2 x = 1 1+tan2 x = sec2 x 1+cot2 x = csc2 x 4. For any real number c, treated as constant function, its derivative is zero and hence, we can write (1), (2) and (3) as follows : Differentiation formulas pdf class 12: Using the chain rule, we have ( )( ) ( ) 2 3 4 4 3 8 3 3x 4 dx dy = + , where ( ) 2 3 x4 du dy = + and 4x3 dx du = special integration formulas:
Exponential & logarithmic functions dx dx dx dx dx u dx dx u.
Differentiation is an important topic of class 12th mathematics. If a term in your choice for yp happens to be a solution of the homogeneous ode corresponding to (4), multiply this term by x (or by x 2 if this solution corresponds to a double root of the characteristic equation of the homogeneous ode). Common derivatives polynomials ( ) 0 d c dx = ( ) 1 d x dx = ( ) d cx c dx = (nn) 1 d x nx dx = − d (cx ncxnn) 1 dx = − trig functions (sin cos) d xx dx = (cos sin) d x x dx =− (tan sec) 2 d xx dx = (sec sec tan) d x xx dx = (csc csc cot) d x xx dx =− (cot csc) 2 d xx dx =− inverse trig functions (1 ) 2 1 sin 1 d x dx x − = − (1) 2 1 cos 1 d x dx − =− (1) 2 1 tan 1 d x dx x − = + (1) 2 1 sec 1 d x dx. We can also represent dy/dx = dx y.
Calculus trigonometric derivatives and integrals trigonometric derivatives d dx (sin( x)) = cos( )·0 d dx (cos( )) = sin(0 d dx (tan( x)) = sec2( )· 0 d dx (csc( x)) = csc( )cot( )·0 d dx (sec( )) = sec( )tan(0 d dx (cot(x)) = csc2( x)· 0 d dx (sin 01 (x)) =p 1 1x2 ·xd dx (cos (tan1(x)) = p 1 1x2 0 d dx 1 1 1+x2 ·x 0 d dx (csc 1(x)) = 1 x p x21 ·x0 d dx (sec (cot1 (x)) =1 x p x21 ·x0 d.
Coefficients by substituting yp and its derivatives into (4). Differentiation is used to find the derivative of a. Symbols f(x) → integrand f(x)dx → element of integration ∫→ sign of integral φ(x) → anti. '( ) 3 ( ) '( ) 3 ( ) '( ) 3 ( ) 2 2 2 h x x f x g x x f x f x x f x = = = = = = although integration has been introduced as an antiderivative, the symbol for integration is ‘∫’.
Integration as inverse operation of differentiation.
The derivative of the second term is 1 2 (1 x 2) 1= ( x2x) = 1p 1 x2:hence the derivative of the function y= sin x2 + p 1 x2 is y0= 2x p 1 x4 x p 1 x2: Derivativeof d du d du d 1 du d 1 du • e =e. Integrals producing inverse trigonometric functions. In all the formulas below, f’ means d ( f ( x)) d x = f ′ ( x) and g’ means d ( g ( x)) d x = g ′ ( x).
Differentiation formulas d dx k = 0 (1) d dx [f(x)±g(x)] = f0(x)±g0(x) (2) d dx [k ·f(x)] = k ·f0(x) (3) d dx [f(x)g(x)] = f(x)g0(x)+g(x)f0(x) (4) d dx f(x) g(x.
(sin +c) cos= d xx dx, 3 ( +c) 2 3 = dx x dx and ( +c)x = x d ee dx thus, anti derivatives (or integrals) of the above cited functions are not unique. If y = y(x) is given implicitly, find derivative to the entire equation with respect to x. Now ( ) 3+x4 = u, and the result is the function y =u2.