In order to prevent damage to the organ, the radiologist must angle the rays to the tumor. Calculate the length of its base and. Our final answer is 8√3.
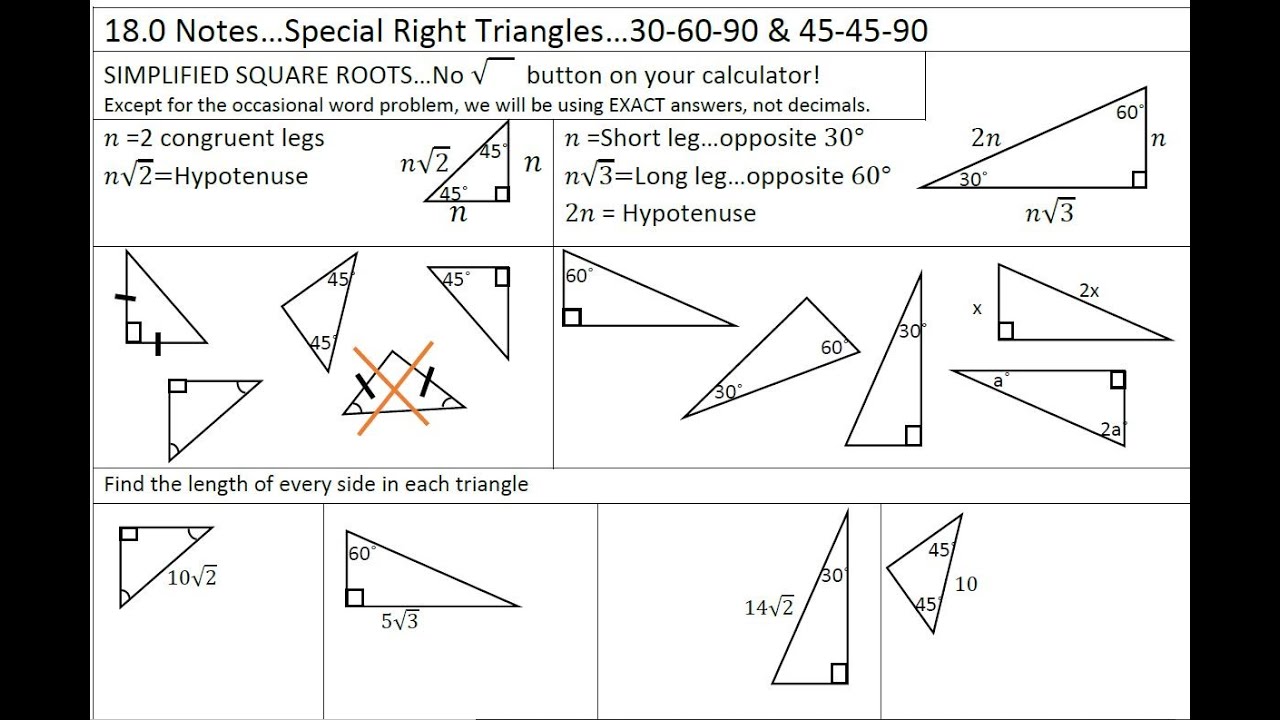
18.0 Notes Special Right Triangles 306090 & 454590
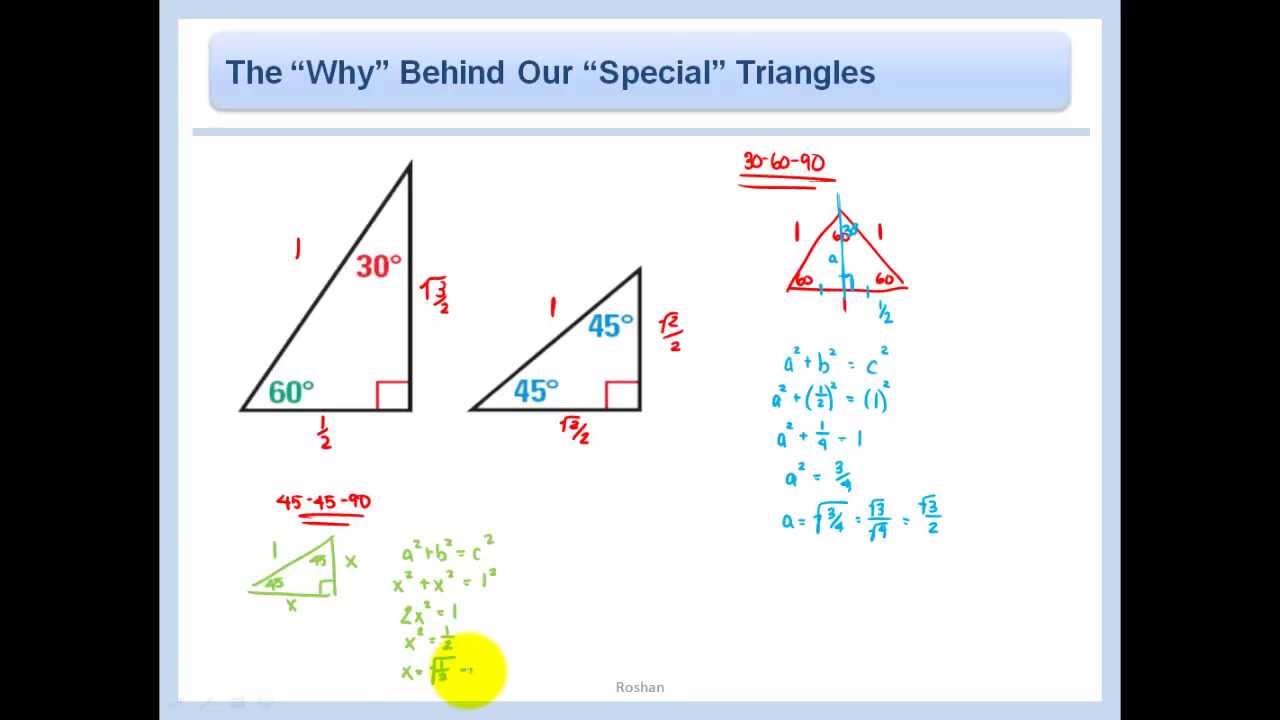
Also, if you know two sides of the triangle, you can find the third one from the pythagorean theorem.
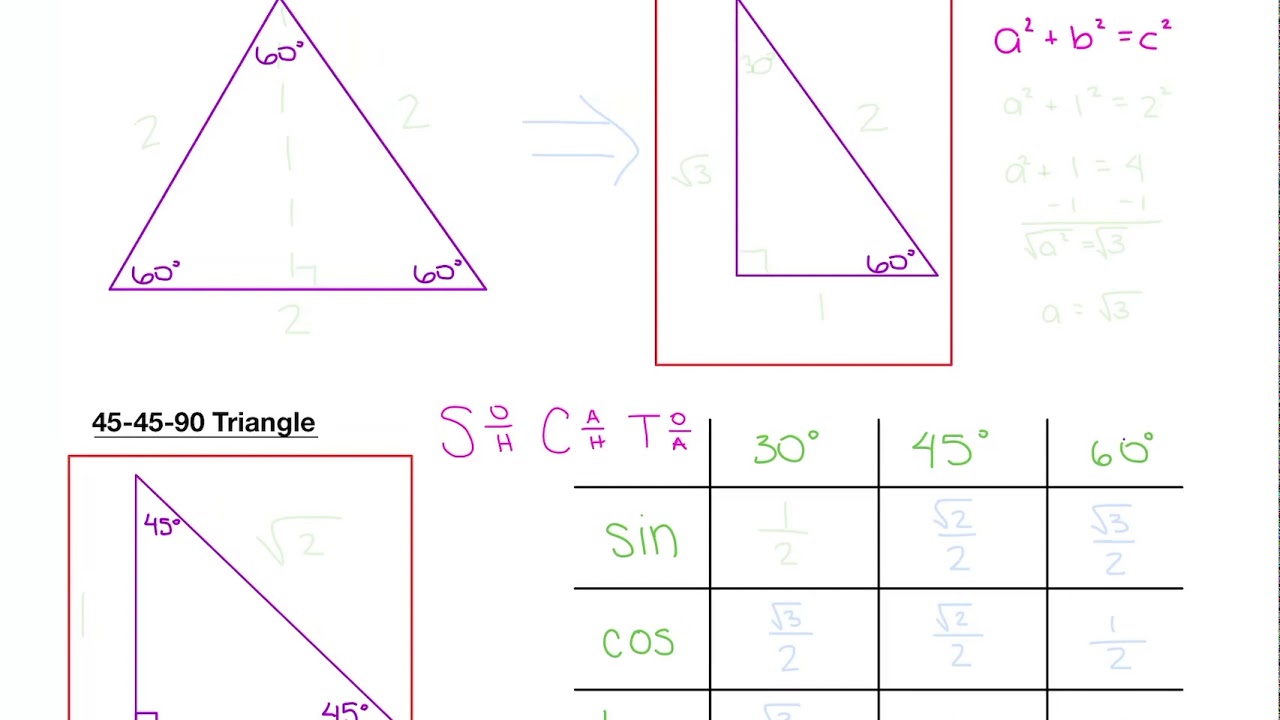
Note that it’s the shape of half a square, cut along the square’s diagonal, and that it’s also an isosceles triangle (both legs have the same length).
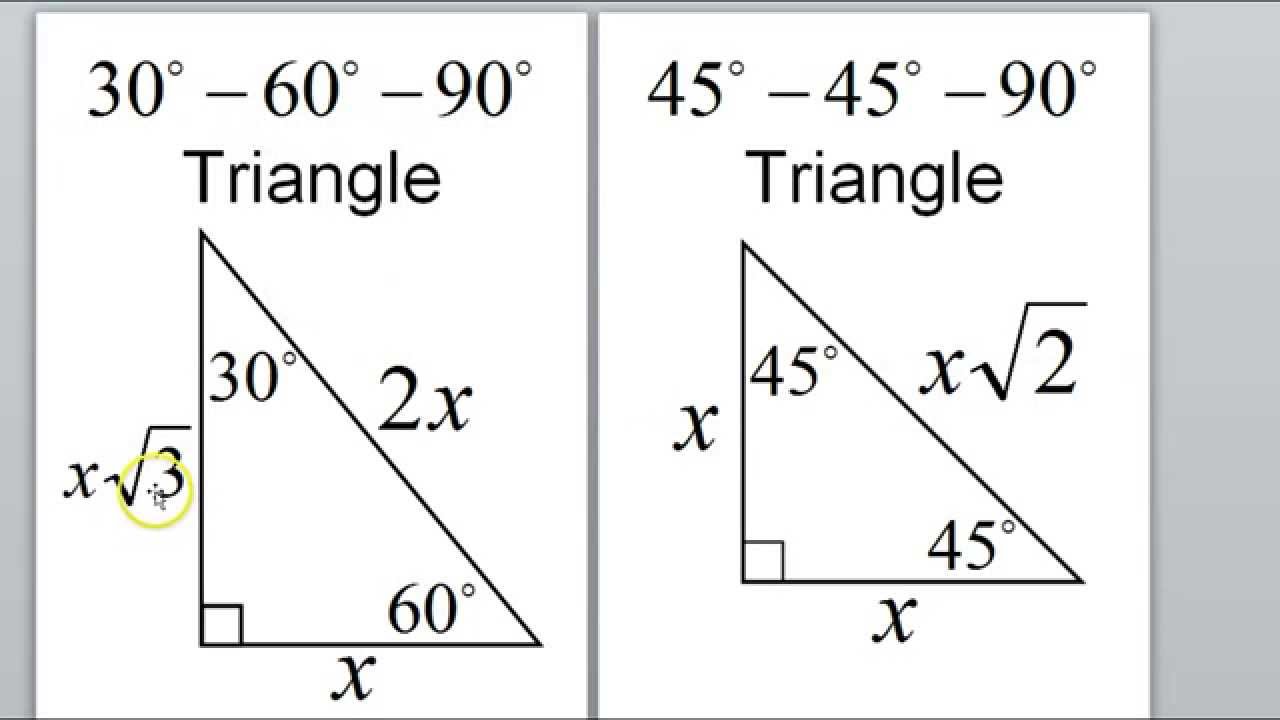
So, x√3 = 4√3 and 2x = 8. This is an isosceles right triangle. A/c = √2/2 so c = a√2. 30 60 90 triangle short side, medium side or hypotenuse.
Leave your answers as radicals in simplest form.
It has angles of 30°, 60°, and 90°. `area = a² / 2`. In our case, one leg is a base and the other is the height, as there is a right angle between them. Long side (opposite the 60 60 degree angle) = x√3 x 3.
A = first side length.
Up to 24% cash back notes: The hypotenuse of the triangle is. So, the side lengths of the triangle are as follows: A patient is being treated with radiotherapy for a tumor that is behind a vital organ.
90° triangle is 6√2 mm.
This page summarizes two types of right triangles which often appear in the study of mathematics and physics. The hypotenuse of a 45°; Relationships between sides of special triangles. Hence, the triangle has the side lengths as follows:
You simply apply pythagorean theorem as follows:
Thanks to all of you who support me on patreon. Here, x√2 = 4√2, so x = 4. Remember, the hypotenuse is always the measure of each. B/c = sin (60°) = √3/2 so b = c√3/2 = a√3.
Hypotenuse (opposite the 90 90 degree angle) = 2x 2 x.
However, the methods described above are more useful as they need to have only one side of the 30 60 90 triangle given. A/c = sin (30°) = 1/2 so c = 2a. Short side (opposite the 30 30 degree angle) = x x. Clicking reset clears all of the boxes.
To find the area of such triangle, use the basic triangle area formula is area = base * height / 2.
As soon as you click that box, the output boxes will automatically get filled in by the calculator. So the area of 45 45 90 triangles is: The 5 choices you have are: 1) x 20 y 45° 2) a63 b 30° 3) x 72 y 45° 4) x y17 60°
B = second side length (equals to first side) c = hypotenuse.