Unit 7 day 1 notes/hw. Also, if you know two sides of the triangle, you can find the third one from the pythagorean theorem. Special right triangles cheat sheet.
BowerPoints & Examples
The 5 choices you have are:
In our case, one leg is a base and the other is the height, as there is a right angle between them.
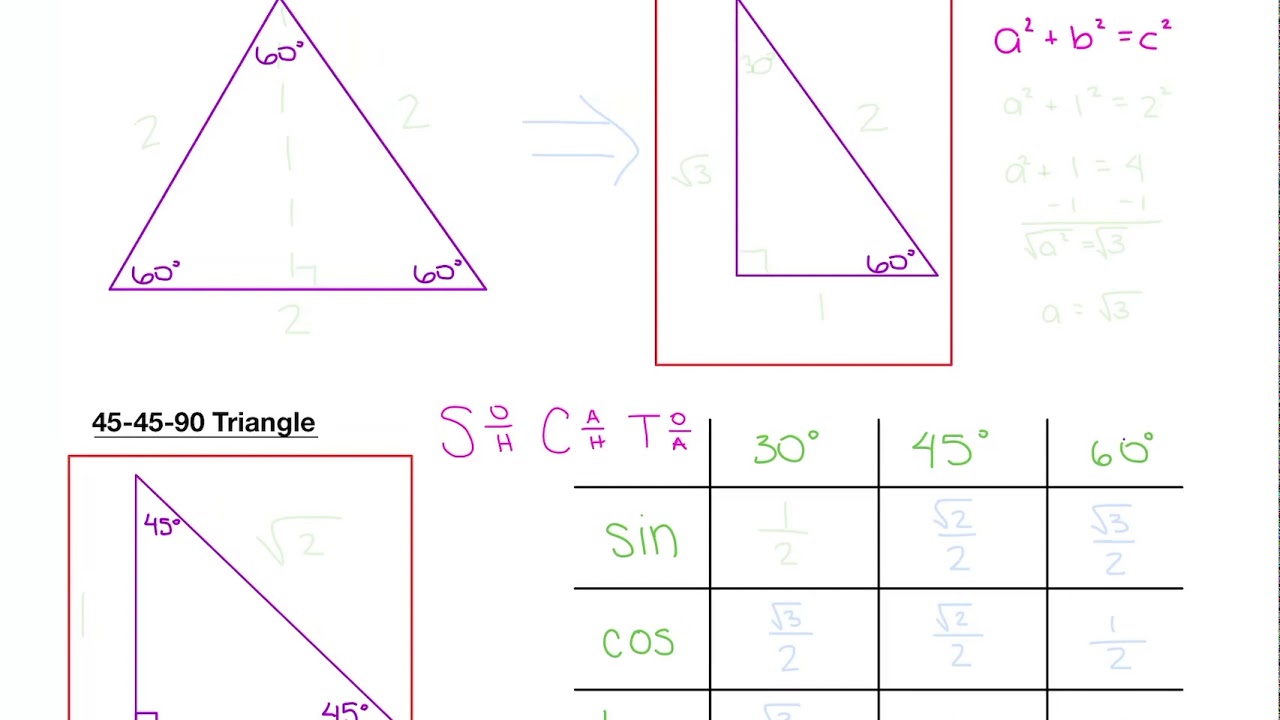
I n s t r u c t i o n s start by entering the length of a triangle side. 45 45 90 triangle side or hypotenuse. B/c = sin (60°) = √3/2 so b = c√3/2 = a√3. Assuming x is the length of the leg and b is the length of the hypotenuse and using the pythagorean theorem:
The sum of the angles is 180°
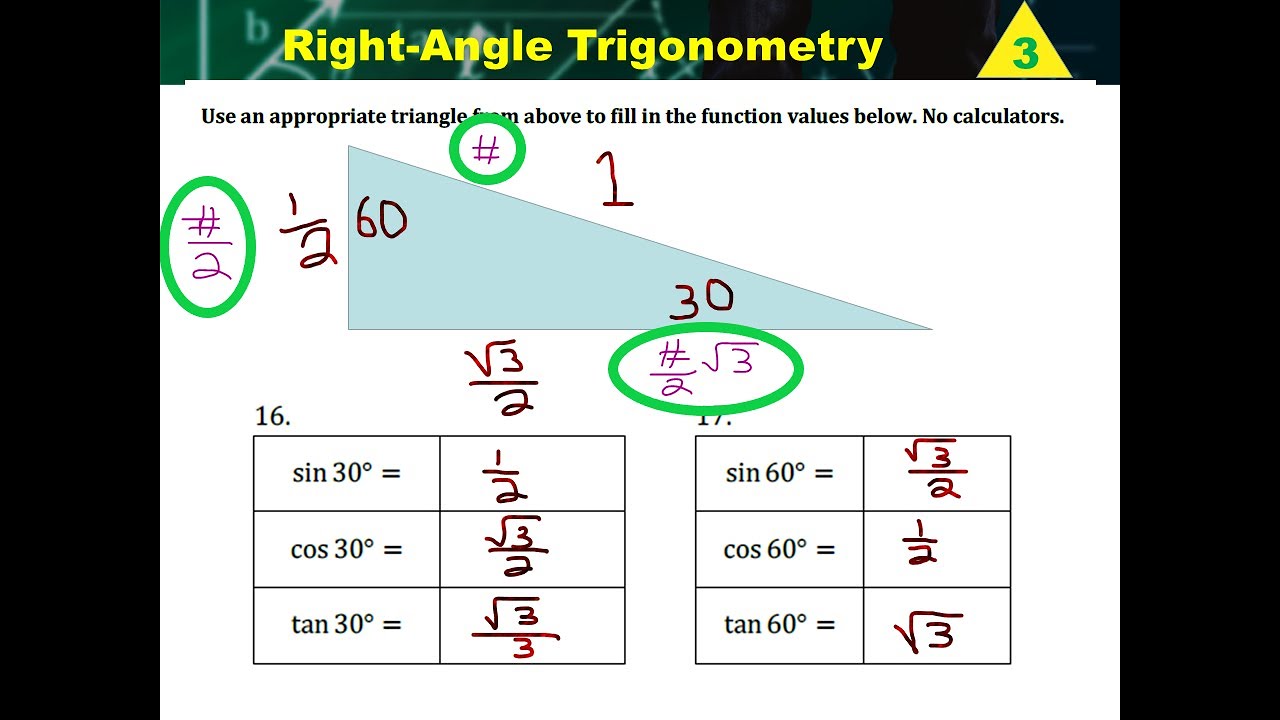
Calculate the length of its base and. 30 60 90 triangle short side, medium side or hypotenuse. `area = a² / 2`. 45° x = 202, y = 20 2) a63 b 30° a = 12, b = 6 3) x 72 y 45° x = 14, y = 72 4) x y17 60° x = 34, y = 173 5) 143 x y 45° x = 76, y = 76 6) x y 19 30° x = 38, y = 193 7) 182 m n 45° m = 18, n = 18 8) u v 5 60° u = 25, v = 15 9) x 83 y 60° x = 16, y = 8 10) xy 13 2 30° x = 13, y = 133 2
This page summarizes two types of right triangles which often appear in the study of mathematics and physics.
Long side (opposite the 60 degree angle) = x√3. The hypotenuse of a 45°; Knowing the ratios of the angles or sides of these. And the hypotenuse is 2 times the shortest leg, or 2 √ 3) and so on.
A special right triangle is a right triangle with some regular feature that makes calculations on the triangle easier, or for which simple formulas exist.
Thus if you know that the side opposite the 60 degree angle measures 5 inches then then this is √3 times as long as the side opposite the 30 degree so the side opposite the 30 degree angle is 5/√3 inches long. With special right triangles with angles of 45 45 90, this same formula can be applied. This is an isosceles right triangle. Explains a simple pictorial way to remember basic reference angle values.
Hypotenuse (opposite the 90 90 degree angle) = 2x 2 x.
30 60 90 and 45 45 90 triangle calculator. Hypotenuse (opposite the 90 degree angle) = 2x. It has angles of 30°, 60°, and 90°. (angles that form a simple ratio) side based right triangle:
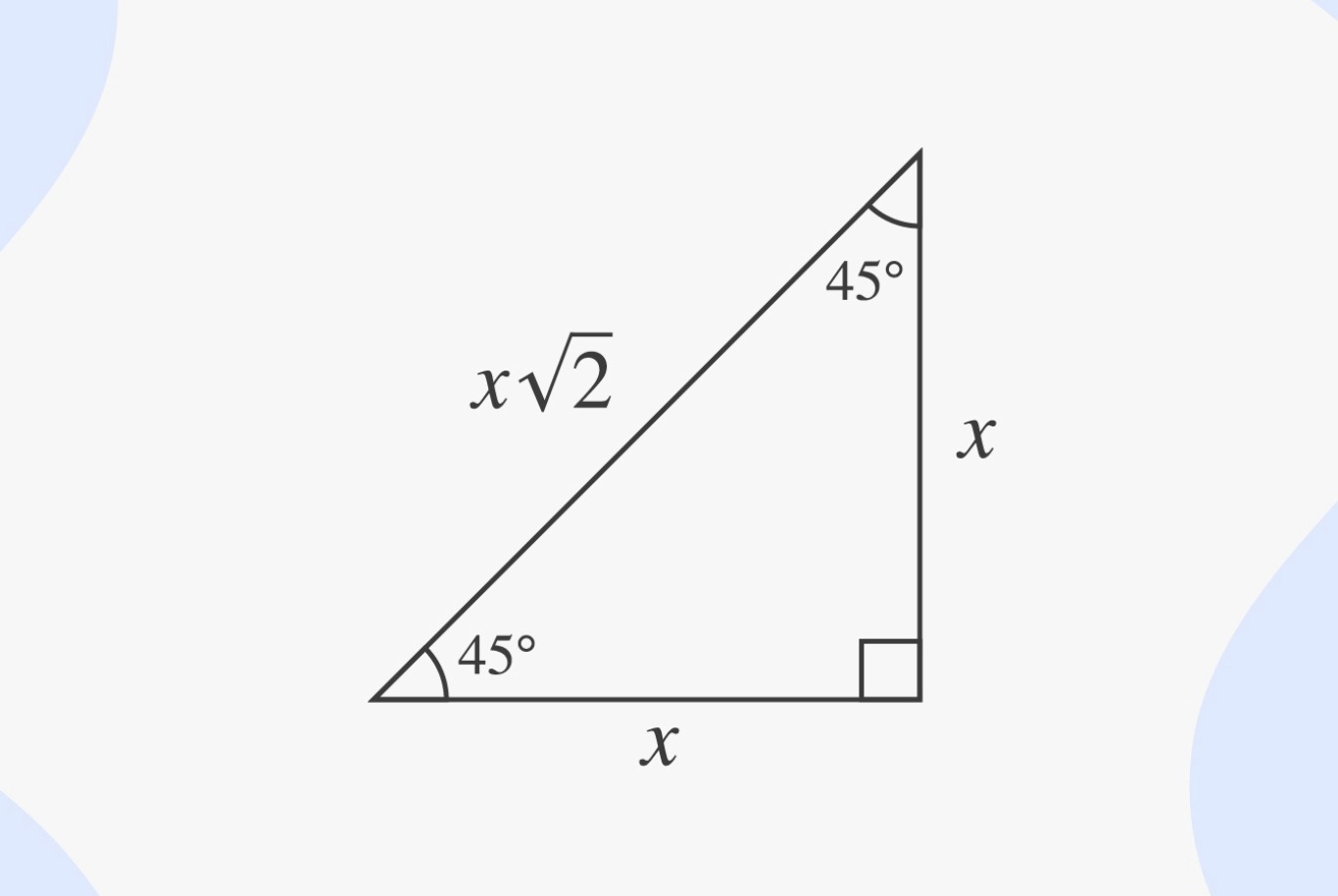
What are the characteristics of a 45 45 90 triangle?
X 2 + x 2 = b 2. 90° triangle is 6√2 mm. Refers to the angles of the triangle. A= \frac {1} {2} \cdot b \cdot h a= 21.
A/c = √2/2 so c = a√2.
B = second side length (equals to first side) c = hypotenuse. How do you find the sides of a 30 60 90 triangle? A/c = sin (30°) = 1/2 so c = 2a. Long side (opposite the 60 60 degree angle) = x√3 x 3.
Hypotenuse = leg * hypotenuse leg = find the value of x in.
In this triangle, the shortest leg ( x) is √ 3, so for the longer leg, x √ 3 = √ 3 * √ 3 = √ 9 = 3. Short side (opposite the 30 degree angle) = x. You simply apply pythagorean theorem as follows: Short side (opposite the 30 30 degree angle) = x x.
Then click on which type of side it is.
However, the methods described above are more useful as they need to have only one side of the 30 60 90 triangle given. The side opposite the 30° angle is always the smallest, because 30 degrees is the smallest angle. So the area of 45 45 90 triangles is: A = first side length.